We present several functional inequalitiesfor finite difference gradients, such asa Cheeger inequality, Poincaré and (modified) logarithmic Sobolev inequalities,associated deviation estimates,and an exponential integrability property.In the particular case of the geometric distribution on ${\mathbb{N}}$ 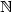 we use an integration by parts formula to computethe optimal isoperimetric and Poincaré constants,and to obtain an improvement of ourgeneral logarithmic Sobolev inequality.By a limiting procedure we recover the correspondinginequalities for the exponential distribution.These results have applications to interacting spin systems undera geometric reference measure.
we use an integration by parts formula to computethe optimal isoperimetric and Poincaré constants,and to obtain an improvement of ourgeneral logarithmic Sobolev inequality.By a limiting procedure we recover the correspondinginequalities for the exponential distribution.These results have applications to interacting spin systems undera geometric reference measure.