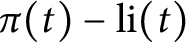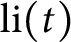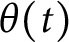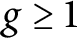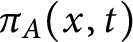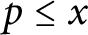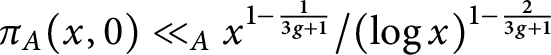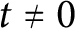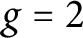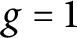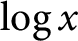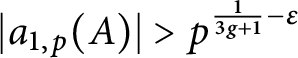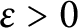Let
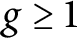 $g \geq 1$
be an integer and let
$g \geq 1$
be an integer and let
 $A/\mathbb Q$
be an abelian variety that is isogenous over
$A/\mathbb Q$
be an abelian variety that is isogenous over
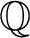 $\mathbb Q$
to a product of g elliptic curves defined over
$\mathbb Q$
to a product of g elliptic curves defined over
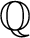 $\mathbb Q$
, pairwise non-isogenous over
$\mathbb Q$
, pairwise non-isogenous over
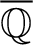 $\overline {\mathbb Q}$
and each without complex multiplication. For an integer t and a positive real number x, denote by
$\overline {\mathbb Q}$
and each without complex multiplication. For an integer t and a positive real number x, denote by
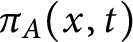 $\pi _A(x, t)$
the number of primes
$\pi _A(x, t)$
the number of primes
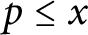 $p \leq x$
, of good reduction for A, for which the Frobenius trace
$p \leq x$
, of good reduction for A, for which the Frobenius trace
 $a_{1, p}(A)$
associated to the reduction of A modulo p equals t. Assuming the Generalized Riemann Hypothesis for Dedekind zeta functions, we prove that
$a_{1, p}(A)$
associated to the reduction of A modulo p equals t. Assuming the Generalized Riemann Hypothesis for Dedekind zeta functions, we prove that
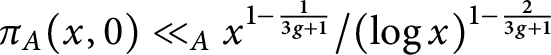 $\pi _A(x, 0) \ll _A x^{1 - \frac {1}{3 g+1 }}/(\operatorname {log} x)^{1 - \frac {2}{3 g+1}}$
and
$\pi _A(x, 0) \ll _A x^{1 - \frac {1}{3 g+1 }}/(\operatorname {log} x)^{1 - \frac {2}{3 g+1}}$
and
 $\pi _A(x, t) \ll _A x^{1 - \frac {1}{3 g + 2}}/(\operatorname {log} x)^{1 - \frac {2}{3 g + 2}}$
if
$\pi _A(x, t) \ll _A x^{1 - \frac {1}{3 g + 2}}/(\operatorname {log} x)^{1 - \frac {2}{3 g + 2}}$
if
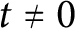 $t \neq 0$
. These bounds largely improve upon recent ones obtained for
$t \neq 0$
. These bounds largely improve upon recent ones obtained for
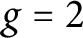 $g = 2$
by Chen, Jones, and Serban, and may be viewed as generalizations to arbitrary g of the bounds obtained for
$g = 2$
by Chen, Jones, and Serban, and may be viewed as generalizations to arbitrary g of the bounds obtained for
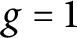 $g=1$
by Murty, Murty, and Saradha, combined with a refinement in the power of
$g=1$
by Murty, Murty, and Saradha, combined with a refinement in the power of
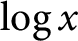 $\operatorname {log} x$
by Zywina. Under the assumptions stated above, we also prove the existence of a density one set of primes p satisfying
$\operatorname {log} x$
by Zywina. Under the assumptions stated above, we also prove the existence of a density one set of primes p satisfying
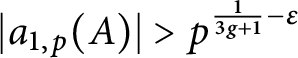 $|a_{1, p}(A)|>p^{\frac {1}{3 g + 1} - \varepsilon }$
for any fixed
$|a_{1, p}(A)|>p^{\frac {1}{3 g + 1} - \varepsilon }$
for any fixed
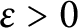 $\varepsilon>0$
.
$\varepsilon>0$
.
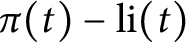 $\pi (t)-\operatorname {\textrm {li}}(t)$
$\pi (t)-\operatorname {\textrm {li}}(t)$