We discuss ℓp-maximal regularity of power-bounded operators and relate the discrete to the continuous time problem for analytic semigroups. We give a complete characterization of operators with ℓ1 and 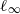 -maximal regularity. We also introduce an unconditional form of Ritt’s condition for power-bounded operators, which plays the role of the existence of an
-maximal regularity. We also introduce an unconditional form of Ritt’s condition for power-bounded operators, which plays the role of the existence of an  -calculus, and give a complete characterization of this condition in the case of Banach spaces which are L1-spaces, C(K)-spaces or Hilbert spaces.
-calculus, and give a complete characterization of this condition in the case of Banach spaces which are L1-spaces, C(K)-spaces or Hilbert spaces.
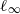 -MAXIMAL REGULARITY FOR POWER-BOUNDED OPERATORS
-MAXIMAL REGULARITY FOR POWER-BOUNDED OPERATORS

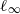 -MAXIMAL REGULARITY FOR POWER-BOUNDED OPERATORS
-MAXIMAL REGULARITY FOR POWER-BOUNDED OPERATORS
 -calculus, and give a complete characterization of this condition in the case of Banach spaces which are
-calculus, and give a complete characterization of this condition in the case of Banach spaces which are 