A Numerical Method of Finding a Suitable Bonus Scale

Extract

The bonus system used by Finnish insurers is as follows: Policies are divided into 4 bonus classes, C₁, C₂, C₃, C₄, each of them being initially placed in class C₁. After one claimless year, a policy placed in class C_h is transferred to class C_h+1 (h ≤ 3) from the beginning of the following year. After a claim year, each policy is entered back to class C₁. (Whether the number of claims is one or more does not play any role in this transition system.) From the initial premium, i.e. the premium in class C₁, a reduction of p_i, per cent is granted in class C_i. In numbers these percentages are as follows:

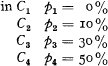
In present investigations there is a trend to replace this scale by another in which mere contingency would not cause too great a change in the premium and in which the „test period” would be longer.

Let us suppose that we want to find a suitable percentage scale P = (P₁, P₂, …, P_m) for a bonus system (C_l, C₂, …, C_m) with a given transition mechanism according to which for each bonus class C_h two bonus classes C_i and C_j are given to which the policy is transferred after one year from C_h according as there have been claims or not.