Research Article
Geometric coverings of groups and their directions
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 177-184
-
- Article
-
- You have access
- Export citation
A natural proof of the cyclotomic identity
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 185-189
-
- Article
-
- You have access
- Export citation
Sums of deficiencies of algebroid functions
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 191-200
-
- Article
-
- You have access
- Export citation
The differentiability of convex functions on topological linear spaces
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 201-213
-
- Article
-
- You have access
- Export citation
On local equivalence for vector field systems
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 215-229
-
- Article
-
- You have access
- Export citation
On the isometry of surfaces preserving the lines of curvature
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 231-245
-
- Article
-
- You have access
- Export citation
Burnside rings of finite representation type
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 247-251
-
- Article
-
- You have access
- Export citation
Approximation by Boolean sums of linear operators: Telyakovskiǐ-type estimates
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 253-266
-
- Article
-
- You have access
- Export citation
Frattini classes of saturated formations of finite groups
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 267-286
-
- Article
-
- You have access
- Export citation
Translation invariant linear functionals on segal algebras
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 287-292
-
- Article
-
- You have access
- Export citation
Orbital decompositions of representations of non-simply connected nilpotent groups
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 293-306
-
- Article
-
- You have access
- Export citation
On the conformal deformation of Riemannian structures
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 307-313
-
- Article
-
- You have access
- Export citation
A class of null sets associated with convex functions on Banach spaces
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 315-322
-
- Article
-
- You have access
- Export citation
Average distance constants for polygons in spaces with non-positive curvature
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 323-333
-
- Article
-
- You have access
- Export citation
The C*-algebras of some inverse semigroups
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 335-348
-
- Article
-
- You have access
- Export citation
The Stone-Čech compactification and weakly Fréchet spaces
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 349-352
-
- Article
-
- You have access
- Export citation
Front matter
BAZ volume 42 issue 2 Cover and Front matter
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back matter
BAZ volume 42 issue 2 Cover and Back matter
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. b1-b2
-
- Article
-
- You have access
- Export citation


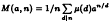 and
and  , where
, where 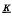 means the conjugacy class of
means the conjugacy class of 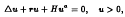
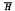 < 0, where
< 0, where