Solitary water waves travelling through a forked channel region are studied via a new nonlinear wave model. This novel (reduced) one-dimensional (1D) model captures the effective features of the reflection and transmission of solitary waves passing through a two-dimensional (2D) branching channel region. Using an appropriate change of coordinates, the 2D wave system is defined in a simpler geometric configuration that allows a straightforward reduction to a 1D graph-like configuration. The Jacobian of the change of coordinates leads to a variable coefficient in the 1D model, which contains information about the angles of the diverting reaches, a feature that is not present in any previous water wave model on networks. Furthermore, this new formulation is more general, in allowing both symmetric and asymmetric branching configurations to be considered. A new compatibility condition is deduced, which is used at the corresponding branching node. The 2D and 1D dynamics are compared, with very good agreement.

The fluid dynamics of the atmosphere and oceans is to a large extent controlled by the slow evolution of a scalar field called ‘potential vorticity’ (PV), with relatively fast motions such as inertia-gravity waves playing only a minor role. This state of affairs is commonly referred to as ‘balance’. Potential vorticity is a special scalar field which is materially conserved in the absence of diabatic effects and dissipation, effects that are generally weak in the atmosphere and oceans. Moreover, in a balanced flow, PV induces the entire fluid motion and its thermodynamic structure (Hoskins et al., Q. J. R. Meteorol. Soc., vol. 111, 1985, pp. 877–946). While exact balance is generally not achievable, it is now well established that balance holds to a high degree of accuracy in rapidly rotating and strongly stratified flows. Such flows are characterised by both a small Rossby number, 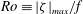$\mathit{Ro}\equiv |{\it\zeta}|_{max}/f$, and a small Froude number, 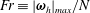$\mathit{Fr}\equiv |{\bf\omega}_{h}|_{max}/N$, where 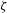${\it\zeta}$ and 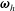${\bf\omega}_{h}$ are the relative vertical and horizontal vorticity components, while $f$ and 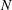$N$ are the Coriolis and buoyancy frequencies. In fact, balance can even be a good approximation when 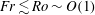$\mathit{Fr}\lesssim \mathit{Ro}\sim \mathit{O}(1)$. In this study, we examine how balance depends specifically on Prandtl’s ratio, 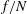$f/N$, in unforced freely evolving turbulence. We examine a wide variety of turbulent flows, at a mature and complex stage of their evolution, making use of the fully non-hydrostatic equations under the Boussinesq and incompressible approximations. We perform numerical simulations at exceptionally high resolution in order to carefully assess the degree to which balance holds, and to determine when it breaks down. For this purpose, it proves most useful to employ an invariant PV-based Rossby number ${\it\varepsilon}$, together with 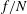$f/N$. For a given ${\it\varepsilon}$, our key finding is that – for at least tens of characteristic vortex rotation periods – the flow is insensitive to 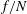$f/N$ for all values for which the flow remains statically stable (typically 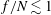$f/N\lesssim 1$). Only the vertical velocity varies in proportion to 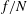$f/N$, in line with quasi-geostrophic (QG) scaling for which 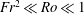$\mathit{Fr}^{2}\ll \mathit{Ro}\ll 1$. We also find that as ${\it\varepsilon}$ increases towards unity, the maximum 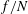$f/N$ attainable decreases towards 0. No statically stable flows occur for 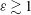${\it\varepsilon}\gtrsim 1$. For all stable flows, balance is found to hold to a remarkably high degree: as measured by an energy norm, imbalance never exceeds more than a few per cent of the balance, even in flows where 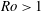$\mathit{Ro}>1$. The vertical velocity 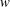$w$ remains a tiny fraction of the horizontal velocity 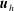$\boldsymbol{u}_{h}$, even when 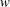$w$ is dominantly balanced. Finally, typical vertical to horizontal scale ratios 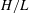$H/L$ remain close to 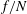$f/N$, as found previously in QG turbulence for which $\mathit{Fr}\sim \mathit{Ro}\ll 1$.

We propose a new rigorous method for estimating statistical quantities in fluid dynamics such as the (average) energy dissipation rate directly from the equations of motion. The method is tested on shear flow, channel flow, Rayleigh–Bénard convection and porous medium convection.

The Faraday problem is an important pattern-forming system that provides some middle ground between systems where the initial instability involves just a single mode, and in which complexity then results from mode interactions or secondary bifurcations, and cases where a system is highly turbulent and many spatial and temporal modes are excited. It has been a rich source of novel patterns and of theoretical work aimed at understanding how and why such patterns occur. Yet it is particularly challenging to tie theory to experiment: the experiments are difficult to perform; the parameter regime of interest (large box, moderate viscosity) along with the technical difficulties of solving the free-boundary Navier–Stokes equations make numerical solution of the problem hard; and the fact that the instabilities result in an entire circle of unstable wavevectors presents considerable theoretical difficulties. In principle, weakly nonlinear theory should be able to predict which patterns are stable near pattern onset. In this paper we present the first quantitative comparison between weakly nonlinear theory of the full Navier–Stokes equations and (previously published) experimental results for the Faraday problem with multiple-frequency forcing. We confirm that three-wave interactions sit at the heart of why complex patterns are stabilised, but also highlight some discrepancies between theory and experiment. These suggest the need for further experimental and theoretical work to fully investigate the issues of pattern bistability and the role of bicritical/tricritical points in determining bifurcation structure.

A turbulent lifted slot-jet flame is studied using direct numerical simulation (DNS). A one-step chemistry model is employed with a mixture-fraction-dependent activation energy which can reproduce qualitatively the dependence of the laminar burning rate on the equivalence ratio that is typical of hydrocarbon fuels. The basic structure of the flame base is first examined and discussed in the context of earlier experimental studies of lifted flames. Several features previously observed in experiments are noted and clarified. Some other unobserved features are also noted. Comparison with previous DNS modelling of hydrogen flames reveals significant structural differences. The statistics of flow and relative edge-flame propagation velocity components conditioned on the leading edge locations are then examined. The results show that, on average, the streamwise flame propagation and streamwise flow balance, thus demonstrating that edge-flame propagation is the basic stabilisation mechanism. Fluctuations of the edge locations and net edge velocities are, however, significant. It is demonstrated that the edges tend to move in an essentially two-dimensional (2D) elliptical pattern (laterally outwards towards the oxidiser, then upstream, then inwards towards the fuel, then downstream again). It is proposed that this is due to the passage of large eddies, as outlined in Su et al. (Combust. Flame, vol. 144 (3), 2006, pp. 494–512). However, the mechanism is not entirely 2D, and out-of-plane motion is needed to explain how flames escape the high-velocity inner region of the jet. Finally, the time-averaged structure is examined. A budget of terms in the transport equation for the product mass fraction is used to understand the stabilisation from a time-averaged perspective. The result of this analysis is found to be consistent with the instantaneous perspective. The budget reveals a fundamentally 2D structure, involving transport in both the streamwise and transverse directions, as opposed to possible mechanisms involving a dominance of either one direction of transport. It features upstream transport balanced by entrainment into richer conditions, while on the rich side, upstream turbulent transport and entrainment from leaner conditions balance the streamwise convection.

When a ball is dropped in fine very loose sand, a splash and subsequently a jet are observed above the bed, followed by a granular eruption. To directly and quantitatively determine what happens inside the sand bed, high-speed X-ray tomography measurements are carried out in a custom-made set-up that allows for imaging of a large sand bed at atmospheric pressures. Herewith, we show that the jet originates from the pinch-off point created by the collapse of the air cavity formed behind the penetrating ball. Subsequently, we measure how the entrapped air bubble rises through the sand, and show that this is consistent with bubbles rising in continuously fluidized beds. Finally, we measure the packing fraction variation throughout the bed. From this we show that there is (i) a compressed area of sand in front of and next to the ball while the ball is moving down, (ii) a strongly compacted region at the pinch-off height after the cavity collapse and (iii) a relatively loosely packed centre in the wake of the rising bubble.

The modulation of small-scale velocity and velocity gradient quantities by concurrent large-scale velocity fluctuations is observed by consideration of the Kullback–Leibler divergence. This is a measure that quantifies the loss of information in modelling a statistical distribution of small-scale quantities conditioned on concurrent positive large-scale fluctuations by that conditioned on negative large-scale fluctuations. It is observed that the small-scale turbulence is appreciably ‘rougher’ when the concurrent large-scale fluctuation is positive in the low-speed side of a fully developed turbulent mixing layer, which gives further evidence to the convective scale modulation argument of Buxton & Ganapathisubramani (Phys. Fluids, vol. 26, 2014, 125106, 1–19). The definition of the small scales is varied, and regardless of whether the small-scale fluctuations are dominated by dissipation or have the characteristic features of inertial range turbulence they are shown to be modulated by the concurrent large-scale fluctuations. The modulation is observed to persist even when there is a large gap in wavenumber space between the small and large scales, although local maxima are observed at intermediate length scales that are significantly larger than the predefined small scales. Finally, it is observed that the modulation of small-scale dissipation is greater than that for enstrophy with the modulation of the vortex stretching term, indicative of the interaction between strain rate and rotation, being intermediate between the two.

In the current literature, the dispersion relation of parametrically forced surface waves is often identified with that of free unforced waves. We revisit here the theoretical description of Faraday waves, showing that forcing and dissipation play a significant role in the dispersion relation, rendering it bi-valued. We then determine the instability thresholds and the wavenumber selection in cases of both short and long waves. We show that the bifurcation can be either supercritical or subcritical, depending on the depth.

A theoretical model of water wave overwash of a thin floating plate is proposed. The nonlinear shallow-water equations are used to model the overwash, and the linear potential-flow/thin-plate model to force it. Model predictions are compared with overwash depths measured during a series of laboratory wave basin experiments. The model is shown to be accurate for incident waves of low steepness or short length.