Abstract
Abstracts of papers
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 290-302
-
- Article
-
- You have access
- Export citation
Research Article
A minimal pair of recursively enumerable degrees
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 159-168
-
- Article
- Export citation
Decidability and undecidability of extensions of second (first) order theory of (generalized) successor1
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 169-181
-
- Article
- Export citation
Decision problems for multiple successor arithmetics1
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 182-190
-
- Article
- Export citation
Algebraic semantics for modal logics II
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 191-218
-
- Article
- Export citation
The undecidability of the Turing machine immortality problem1
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 219-234
-
- Article
- Export citation
Une Généralisation de l'ultraproduit
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 235-244
-
- Article
- Export citation
Reviews
Reviews - Noam Chomsky. Syntactic structures. Janua linguarum, Studia memoriae Nicolai van Wijk dedicata, series minor no. 4. Mouton & Co., ‘s-Gravenhage1957, 116 pp. - Noam Chomsky. Three models for the description of language. A reprint of XXIII 71. Readings in mathematical psychology, volume II, edited by R. Duncan Luce, Robert R. Bush, and Eugene Galanter, John Wiley and Sons, Inc., New York, London, and Sydney, 1965, pp. 105–124. - Noam Chomsky. Logical structures in language. American documentation, vol. 8 (1957), pp. 284–291. - Noam Chomsky and George A. Miller. Finite state languages. Information and control, vol. 1 (1958), pp. 91–112. Reprinted in Readings in mathematical psychology, volume II, edited by R. Duncan Luce, Robert R. Bush, and Eugene Galanter, John Wiley and Sons, Inc., New York, London, and Sydney, 1965, pp. 156–171. - Noam Chomsky. On certain formal properties of grammars. Information and control, vol. 2 (1959), pp. 137–167. Reprinted in Readings in mathematical psychology, volume II, edited by R. Duncan Luce, Robert R. Bush, and Eugene Galanter, John Wiley and Sons, Inc., New York, London, and Sydney, 1965, pp. 125–155. - Noam Chomsky. A note on phrase structure grammars. Information and control, vol. 2 (1959), pp. 393–395. - Noam Chomsky. On the notion “rule of grammar.”Structure of language and its mathematical aspects, Proceedings of symposia in applied mathematics, vol. 12, American Mathematical Society, Providence 1961, pp. 6–24. - Arthur Sard, Noam Chomsky, W. P. Livant, A. G. Oettinger, L. M. Court. Comments. Structure of language and its mathematical aspects, Proceedings of symposia in applied mathematics, vol. 12, American Mathematical Society, Providence 1961, pp. 255–257.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 245-251
-
- Article
- Export citation
Tadeusz Batóg. A contribution to axiomatic phonology. English, with Polish and Russian summaries. Studia logica, vol. 13 (1962), pp. 67–80.
-
- Published online by Cambridge University Press:
- 12 March 2014, p. 251
-
- Article
- Export citation
Roman Sikorski. Boolean algebras. Ergebnisse der Mathematik und ihrer Grenzgebiete, n.s. no. 25. Springer-Verlag, Berlin-Göttingen-Heidelberg1960, IX + 176 pp. - Roman Sikorski. Boolean algebras. Second edition. Ergebnisse der Mathematik und ihrer Grenzgebiete, n.s. no. 25. Academic Press, Inc., New York, and Springer-Verlag, Berlin-Göttingen-Heidelberg, 1964, X + 237 pp.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 251-253
-
- Article
- Export citation
Paul R. Halmos. Lectures on Boolean algebras. D. Van Nostrand Company, Inc., Princeton, Toronto, New York, and London, 1963, v + 147 pp.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 253-254
-
- Article
- Export citation
Hans Hermes. Aufzählbarkeit, Entscheidbarkelt, Berechenbarkeit. Einführung in die Theorie der rekursiven Funktionen. Springer-Verlag, Berlin-Göttingen-Heidelberg1961, X + 246 pp.
-
- Published online by Cambridge University Press:
- 12 March 2014, p. 254
-
- Article
- Export citation
Raphael M. Robinson. The undecidability of pure transcendental extensions of real fields. Zeitschrift für mathematische Logik und Grundlagen der Mathematik, vol. 10 (1964), pp. 275–282. - Raphael M. Robinson. Nérazréšimost′ eléméntarnoj téorii polá racional'nyh funkcij ot odnogo péréménnogo s racional′nymi koefficiéntami (The undecidability of the elementary theory of the field of rational functions of one variable with rational coefficients). Russian translation by M. A. Tajclin. Algébra i logika, Séminar, vol. 2 no. 4 (1963), pp. 5–11.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 254-255
-
- Article
- Export citation
N. A. Šanin. О konstruktivnom ponimanii matématičéskih suždénij (On the constructive interpretation of mathematical judgments). Problémy konstruktivnogo napravléniá v matématiké, 1, Sbornik rabot, edited by N. A. Šanin, Trudy Matématičéskogo Instituta imèni V. A. Stéklova, vol. 52, Izdatél′stvo Akadémii Nauk SSSR, Moscow and Leningrad1958, pp. 226–311.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 255-258
-
- Article
- Export citation
A. A. Markov. O konstruktivnyh funkciáh (On constructive functions). Problémy konstruktivnogo napravléniá v matématiké, 1, Sbornik rabot, edited by N. A. Šanin, Trudy Maté-matičéskogo Instituta imèni V. A. Stéklova, vol. 52, Izdatél′stvo Akadémii Nauk SSSR, Moscow and Leningrad1958, pp. 315–348.
-
- Published online by Cambridge University Press:
- 12 March 2014, p. 258
-
- Article
- Export citation
N. A. Šanin. On the constructive interpretation of mathematical judgments. English translation of XXXI 255 by Elliott Mendelson. American Mathematical Society translations, ser. 2 vol. 23 (1963), pp. 109–189. - A. A. Markov. On constructive functions. English translation of XXXI 258(1) by Moshe Machover. American Mathematical Society translations, vol. 29 (1963), pp. 163–195. - S. C. Kleene. A formal system of intuitionistic analysis. The foundations of intuitionistlc mathematics especially in relation to recursive functions, by Stephen Cole Kleene and Richard Eugene Vesley, Studies in logic and the foundations of mathematics, North-Holland Publishing Company, Amsterdam1965, pp. 1–89. - S. C. Kleene. Various notions of realizability:The foundations of intuitionistlc mathematics especially in relation to recursive functions, by Stephen Cole Kleene and Richard Eugene Vesley, Studies in logic and the foundations of mathematics, North-Holland Publishing Company, Amsterdam1965, pp. 90–132. - Richard E. Vesley. The intuitionistic continuum. The foundations of intuitionistlc mathematics especially in relation to recursive functions, by Stephen Cole Kleene and Richard Eugene Vesley, Studies in logic and the foundations of mathematics, North-Holland Publishing Company, Amsterdam1965, pp. 133–173. - S. C. Kleene. On order in the continuum. The foundations of intuitionistlc mathematics especially in relation to recursive functions, by Stephen Cole Kleene and Richard Eugene Vesley, Studies in logic and the foundations of mathematics, North-Holland Publishing Company, Amsterdam1965, pp. 174–186. - S. C. Kleene. Bibliography.The foundations of intuitionistlc mathematics especially in relation to recursive functions, by Stephen Cole Kleene and Richard Eugene Vesley, Studies in logic and the foundations of mathematics, North-Holland Publishing Company, Amsterdam1965, pp. 187–199.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 258-261
-
- Article
- Export citation
G. Kreisel, D. Lacombe, and J. Shoenfield. Effective operations and partial recursive functionals. Summaries of talks presented at the Summer Institute for Symbolic Logic, Cornell University, 1957, 2nd edn., Communications Research Division, Institute for Defense Analyses, Princeton, N.J., 1960, pp. 364–365. - G. Kreisel, D. Lacombe, and J. R. Shoenfield. Partial recursive functionals and effective operations. Constructivity in mathematics, Proceedings of the colloquium held at Amsterdam, 1957, edited by A. Heyting, Studies in logic and the foundations of mathematics, North Holland Publishing Company, Amsterdam1959, pp. 290–297.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 261-262
-
- Article
- Export citation
H. B. Enderton. Hierarchies in recursive function theory. Transactions of the American Mathematical Society, vol. III (1964), pp. 457–471.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 262-263
-
- Article
- Export citation
Herbert Enderton and David Luckham. Hierarchies over recursive well-orderings. The journal of symbolic logic, vol. 29 no. 4 (for 1964, pub. 1965), pp. 183–190.
-
- Published online by Cambridge University Press:
- 12 March 2014, p. 263
-
- Article
- Export citation
V. A. Uspénskij. Lékcii o vyčislimyh funkciáh (Lectures on computable functions). Gosudarstvénnoé Izdatél′stvo Fiziko-matématičéskoj Literatury, Moscow1960, 492 pp.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 263-264
-
- Article
- Export citation

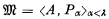 be a
be a 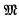 we associate a language
we associate a language