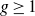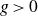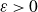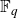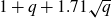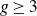Research Article
Prime divisors and the number of conjugacy classes of finite groups
- Part of:
-
- Published online by Cambridge University Press:
- 10 July 2023, pp. 1-16
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Complex multiplication and Noether–Lefschetz loci of the twistor space of a K3 surface
- Part of:
-
- Published online by Cambridge University Press:
- 27 July 2023, pp. 17-38
-
- Article
-
- You have access
- HTML
- Export citation
Weil Sums over Small Subgroups
- Part of:
-
- Published online by Cambridge University Press:
- 15 August 2023, pp. 39-53
-
- Article
-
- You have access
- HTML
- Export citation
On the values taken by slice torus invariants
-
- Published online by Cambridge University Press:
- 23 August 2023, pp. 55-63
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Equidistribution of exponential sums indexed by a subgroup of fixed cardinality
- Part of:
-
- Published online by Cambridge University Press:
- 24 August 2023, pp. 65-94
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Galois groups and prime divisors in random quadratic sequences
- Part of:
-
- Published online by Cambridge University Press:
- 07 September 2023, pp. 95-122
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Non-Orientable Lagrangian Fillings of Legendrian Knots
- Part of:
-
- Published online by Cambridge University Press:
- 27 September 2023, pp. 123-153
-
- Article
-
- You have access
- HTML
- Export citation
Categories of graphs for operadic structures
- Part of:
-
- Published online by Cambridge University Press:
- 28 September 2023, pp. 155-212
-
- Article
-
- You have access
- HTML
- Export citation
Lower bounds on the maximal number of rational points on curves over finite fields
- Part of:
-
- Published online by Cambridge University Press:
- 28 September 2023, pp. 213-238
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Front Cover (OFC, IFC) and matter
PSP volume 176 issue 1 Cover and Front matter
-
- Published online by Cambridge University Press:
- 20 December 2023, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back Cover (OBC, IBC) and matter
PSP volume 176 issue 1 Cover and Back matter
-
- Published online by Cambridge University Press:
- 20 December 2023, pp. b1-b2
-
- Article
-
- You have access
- Export citation