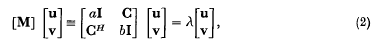Contents
Research Article
On integral operators with kernels involving Bessel functions (Correction and Addendum)
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 583-586
-
- Article
- Export citation
The order-bound topology on Riesz spaces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 587-593
-
- Article
- Export citation
A generalization of the Sα-summation method
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 61-66
-
- Article
- Export citation
Almost hypoelliptic differential operators with variable coefficients
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 287-293
-
- Article
- Export citation
Random Fourier–Stieltjes transforms on partially ordered ǵroups
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 295-306
-
- Article
- Export citation
Some properties of the continued fraction expansion of (m/n) e1/q
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 67-74
-
- Article
- Export citation
Integer points on curves of genus 1
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 595-602
-
- Article
- Export citation
A note on the number of solutions Np of the congruence y2 ≡ x3 − Dx(mod p)
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 603-605
-
- Article
- Export citation
The simultaneous diophantine approximation of certain kth roots*
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 75-86
-
- Article
- Export citation
Summability factors for Riesz loǵarithmic means of order one for a Fourier series†
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 307-320
-
- Article
- Export citation
A Tauberian theorem for absolute summability
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 321-326
-
- Article
- Export citation
Some expansion formulae for Fox's H-function involving exponential functions
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 87-92
-
- Article
- Export citation
Integrals involving classical polynomials
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 607-611
-
- Article
- Export citation
Summability (L) of Fourier series
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 327-331
-
- Article
- Export citation
A theorem on the roots of a certain equation involving Bessel functions
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 93-96
-
- Article
- Export citation
Some formulae for Appell functions
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 613-618
-
- Article
- Export citation
A method for Fourier transforms
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 619-623
-
- Article
- Export citation
On obtaining zero-free regions for the zeta-function from estimates of M(x)
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 333-337
-
- Article
- Export citation
On the shifted eigenvalue problem
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 97-99
-
- Article
- Export citation
On the limiting normality of posterior distributions
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 625-633
-
- Article
- Export citation
 on (
on (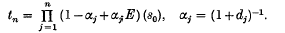
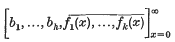
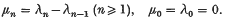
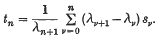
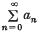 be the given infinite series with {
be the given infinite series with { be the binomial coefficient of
be the binomial coefficient of 
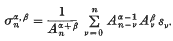


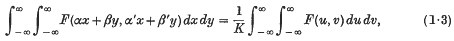
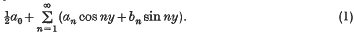

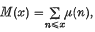 where μ(
where μ(