Barnes and Sloane recently described a “general construction” for lattice packings of equal spheres in Euclidean space. In the present paper we simplify and further generalize their construction, and make it suitable for iteration. As a result we obtain lattice packings in ℝm with density Δ satisfying , as m → ∞ where is the smallest value of k for which the k-th iterated logarithm of m is less than 1. These appear to be the densest lattices that have been explicitly constructed in high-dimensional space. New records are also established in a number of lower dimensions, beginning in dimension 96.

Before turning to the questions to be considered in this paper, we recall two other problems. Let C(a, p) be the class of all convex discs of area not less than a given constant a and perimeter not greater than a given constant p. What is the densest packing and what is the most economical covering of the Euclidean plane with discs from C(a, p)?

Both problems are interesting only if p2/a < 8√3, i.e. if p is less than the perimeter of a regular hexagon of area a. In this case, the densest packing arises from a regular hexagonal tiling by rounding off the corners of the tiles by equal circular arcs so as to obtain smooth hexagons of area a and perimeter p.

Let be k non-overlapping translates of the unit d-ball Bd in euclidean d-space Ed. Let Ck denote the convex hull of their centres and let Sk be a segment of length 2(k– 1). Furthermore, let Vd denote the d-volume. L. Fejes Tóth conjectured in [1], that, for d ≥ 5,

The classical mean value theorem for Dirichlet's polynomials states that

see H. L. Montgomery [7]. This formula is very useful in the theory of the Riemann zeta-function ζ(s). From the approximate functional equation

where | χ(½ + it)| = 1, u, v ≥ 1, 2πuv = t (see E. C. Titchmarsh [8]) it follows that χ(½ + it) can be well approximated by Dirichlet's polynomials of length N< t½.

Let f be a non-zero cusp form of weight k on SL(2, ℤ) with Fourier expansion We assume further that f is normalized (a(1) = 1) and that f is an eigenfunction of the Hecke operators. Define

Let q = pn, p a rational prime, and let be the finite field with q elements. The polynomial ring is considered as an analogue of the ring of rational integers ℤ. Completing the quotient field with respect to the normalized valuation at ∞, and then taking algebraic closure, we obtained the field k∞ whose elements will be called “numbers”.

We consider the Falkner–Skan equation

in the special case β = – 1. It is known that this equation may be integrated twice to get the Riccati equation

If C and Co are two convex bodies in Ed we say that C slides (rolls) freely inside Co if the following condition is satisfied: for each x ∈ ∂C0 (and each rotation R) there is a translation t such that, if gC = C + t (= RC + t), then gC ⊂ Co and x ∈ ∂gC. This work establishes certain topological conditions which ensure the free rolling and sliding of C inside Co. One consequence of these conditions is that, if ∂K ∩ int gK is a topological ball for all rigid motions g, then K is a ball in the geometrical sense.

For a metric space <Ω, ρ> and a ‘measure function’ h, the Hausdorff measure mh on Ω is denned by applying Method II to the premeasure defined by τ(E) = h(d(E)), E ⊆ Ω, where

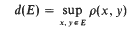
with d(Φ) = 0, is the diameter of E. The set function mh is then a metric outer measure. There are many variations on this definition producing measures also associated with the name Hausdorff. Here we are concerned with those measures which arise when there is a restriction on the sets E for which τ is defined. Such measures arise, for example, as net measures, Rogers [1]. Also we might find it useful to have τ defined only on disks, or only on squares, or only on rectangles with a given relation between vertical and horizontal sides.

Denote by N(t) the number of zeros β + iγ of ζ(s), s = σ + it, with 0 < β < 1 and 0 < γ < t. It is well known that

where

This paper contains estimates of several exponential sums involving Fourier coefficients of certain modular forms. Although the questions which we consider date back, in some cases, to the twenties, our motivation is modern. All of these sums are connected with one approach to the problem of calculating the norm of the Poincaré 0-operator. In this section we state our results (and their antecedents) and then describe their relevance to the θ-operator problem. For simplicity we state and prove all our results for the modular cusp form of weight six, the famous discriminant function

Let

be a cusp form of even integral weight k > 2 for the full modular group. Then the Dirichlet series

is absolutely convergent for σ > ½(k + 1). Hecke showed that LF is an entire function of s satisfying the functional equation

The purpose of this paper is to provide additional evidence to support our view that the modules of generalized fractions introduced in [8] are worth further investigation: we show that, for a module M over a (commutative, Noetherian) local ring A (with identity) having maximal ideal m and dimension n, the n-th local cohomology module may be viewed as a module of generalized fractions of M with respect to a certain triangular subset of An + 1, and we use this work to formulate Hochster's ‘Monomial Conjecture’ [2, Conjecture 1]; in terms of modules of generalized fractions and to make a quick deduction of one of Hochster's results which supports that conjecture.