A space ring R is defined as a domain whose complement in the Moebius space consists of two components. The modulus of R can be defined in variously different but essentially equivalent ways (see e.g. Gehring [3] and Krivov [5]), which is denoted by mod R. Following Gehring [2], we refer to a homeomorphism y(x) of a space domain D as a k-quasiconformal mapping, if the modulus condition
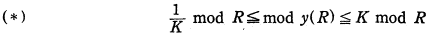
is satisfied for all bounded rings R with their closure 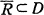 , where y(R) denotes the image of R by y = y(x). Then, it is evident that the inverse of a k-quasi-conformal mapping is itself k-quasiconformal and that a k1-quasiconformal mapping followed by a k2-quasiconformal one is k1k2-quasiconformal. It is also well known that the restriction of a Moebius transformation to a space domain is equivalent to a 1-quasiconformal mapping of its domain.
, where y(R) denotes the image of R by y = y(x). Then, it is evident that the inverse of a k-quasi-conformal mapping is itself k-quasiconformal and that a k1-quasiconformal mapping followed by a k2-quasiconformal one is k1k2-quasiconformal. It is also well known that the restriction of a Moebius transformation to a space domain is equivalent to a 1-quasiconformal mapping of its domain.