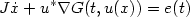We propose a variational model for one of the most importantproblems in traffic networks, namely, the network equilibrium flow that is, traditionallyin the context of operations research, characterized by minimum cost flow. This model has the peculiarity of being formulated by means of a suitable variational inequality (VI) andits solution is called “equilibrium”. This model becomes a minimum cost model when the cost function is separableor, more general, when the Jacobian of the cost operator is symmetric;in such cases a functional representing the total network utility exists.In fact in these cases we can write the first order optimality conditions which turn out to be a VI.In the other situations (i.e., when global utility functional does not exist),which occur much more often in the real problems, we can study the network by looking for equilibrium solutions instead of minimum points.The Lagrangean approach to the study of the VI allows us to introduce dual variables, associated to the constraints of the feasible set, which may receive interesting interpretations in terms of potentials associated to the arcs and the nodes of the network.This interpretation is an extension and generalization of the classic Bellman conditions. Finally, we deepen the analysis of the networks having capacity constraints.


The notion of treewidth is of considerable interest in relation to NP-hard problems.Indeed, several studies have shown that the tree-decomposition method can be used to solve many basic optimization problems in polynomialtime when treewidth is bounded, even if, for arbitrary graphs, computingthe treewidth is NP-hard.Several papers present heuristics with computational experiments.For many graphs the discrepancy between the heuristic resultsand the best lower bounds is still very large. The aim of this paper is to propose two new methodsfor computing the treewidth of graphs: a heuristic and a metaheuristic.The heuristic returns good results in a short computation time,whereas the metaheuristic (a Tabu search method)returns the best results known to have been obtained so far for all the DIMACS vertex coloring / treewidth benchmarks (a well-known collection of graphs used for both vertex coloring and treewidth problems.) Our results actually improve on the previous best results for treewidth problems in 53% of the cases. Moreover, we identify properties of the triangulation processto optimize the computing time of our method.

In this paper we study the existence of subharmonic solutions of the Hamiltonian system $$ J\dot x+ u^* \nabla G(t,u(x)) =e(t)$$ where u is a linear map, G is a C 1-function and e is a continuous function.

Cet article introduit une nouvelle transformation des réseaux de Petri généralisés appelée l'abstraction généralisée. C'est une réduction dont nous montrons qu'elle conserve les invariants du réseau de départ et les propriétés structurelles les plus importantes. Une fonction de transformation de marquages nous permet d'introduire l'étude de la conservation des propriétés comportementales.

Dans cet article, nous proposons une nouvelle méthode de purification pour les problèmes de complémentarité linéaire, monotones. Cette méthode associe à chaque itéré de la suite, générée par une méthode de points intérieurs, une base non nécessairement réalisable. Nous montrons que, sous les hypothèses de complémentarité stricte et de non dégénérescence, la suite des bases converge en un nombre fini d'itérations vers une base optimale qui donne une solution exacte du problème. Le procédé adopté sert également à préconditionner l'algorithme de gradient conjugué lors du calcul de la direction de Newton.