Research Article
A MINIMUM DEGREE CONDITION FOR FRACTIONAL ID-[a,b]-FACTOR-CRITICAL GRAPHS
- Part of:
-
- Published online by Cambridge University Press:
- 07 February 2012, pp. 177-183
-
- Article
-
- You have access
- Export citation
ON LEVI’S THEOREM FOR LEIBNIZ ALGEBRAS
- Part of:
-
- Published online by Cambridge University Press:
- 30 November 2011, pp. 184-185
-
- Article
-
- You have access
- Export citation
POLYNOMIAL ENDOMORPHISMS PRESERVING OUTER RANK IN TWO VARIABLES
- Part of:
-
- Published online by Cambridge University Press:
- 16 February 2012, pp. 186-192
-
- Article
-
- You have access
- Export citation
IMPULSIVE PERIODIC SOLUTIONS FOR SINGULAR PROBLEMS VIA VARIATIONAL METHODS
- Part of:
-
- Published online by Cambridge University Press:
- 16 February 2012, pp. 193-204
-
- Article
-
- You have access
- Export citation
STRONG AND WEAK WEIGHTED NORM INEQUALITIES FOR THE GEOMETRIC FRACTIONAL MAXIMAL OPERATOR
- Part of:
-
- Published online by Cambridge University Press:
- 30 March 2012, pp. 205-215
-
- Article
-
- You have access
- Export citation
SOME REMARKS ON THE RIEMANN ZETA FUNCTION AND PRIME FACTORS OF NUMERATORS OF BERNOULLI NUMBERS
- Part of:
-
- Published online by Cambridge University Press:
- 12 December 2011, pp. 216-223
-
- Article
-
- You have access
- Export citation
CONVOLUTION OF FUNCTIONALS OF DISCRETE-TIME NORMAL MARTINGALES
- Part of:
-
- Published online by Cambridge University Press:
- 16 December 2011, pp. 224-231
-
- Article
-
- You have access
- Export citation
JOINT UNIVERSALITY OF HURWITZ ZETA-FUNCTIONS
- Part of:
-
- Published online by Cambridge University Press:
- 09 July 2012, pp. 232-243
-
- Article
-
- You have access
- Export citation
POSITIVE SOLUTIONS OF A SECOND-ORDER NEUMANN BOUNDARY VALUE PROBLEM WITH A PARAMETER
- Part of:
-
- Published online by Cambridge University Press:
- 16 March 2012, pp. 244-253
-
- Article
-
- You have access
- Export citation
COHOMOLOGY AND PROFINITE TOPOLOGIES FOR SOLVABLE GROUPS OF FINITE RANK
- Part of:
-
- Published online by Cambridge University Press:
- 16 February 2012, pp. 254-265
-
- Article
-
- You have access
- Export citation
GENERALIZED HIGHER DERIVATIONS
- Part of:
-
- Published online by Cambridge University Press:
- 06 January 2012, pp. 266-281
-
- Article
-
- You have access
- Export citation
SPARSE SETS ON THE PLANE AND DENSITY POINTS DEFINED BY FAMILIES OF SEQUENCES
- Part of:
-
- Published online by Cambridge University Press:
- 06 March 2012, pp. 282-290
-
- Article
-
- You have access
- Export citation
c-SECTIONS, SOLVABILITY AND LARGE SUBGROUPS OF FINITE GROUPS
- Part of:
-
- Published online by Cambridge University Press:
- 03 April 2012, pp. 291-302
-
- Article
-
- You have access
- Export citation
ON THE EXPONENTIAL DIOPHANTINE EQUATION x2+p2m=2yn
- Part of:
-
- Published online by Cambridge University Press:
- 21 February 2012, pp. 303-314
-
- Article
-
- You have access
- Export citation
THE MODULUS OF WEAKLY COMPACT MULTIPLIERS ON THE BANACH ALGEBRA L1(𝒢)** OF A LOCALLY COMPACT GROUP
- Part of:
-
- Published online by Cambridge University Press:
- 25 January 2012, pp. 315-321
-
- Article
-
- You have access
- Export citation
ON LOCALLY DEFINED FORMATIONS OF SOLUBLE LIE AND LEIBNIZ ALGEBRAS
- Part of:
-
- Published online by Cambridge University Press:
- 02 February 2012, pp. 322-326
-
- Article
-
- You have access
- Export citation
ON PERIODIC SOLUTIONS FOR FIRST-ORDER DIFFERENTIAL EQUATIONS INVOLVING THE DISTRIBUTIONAL HENSTOCK–KURZWEIL INTEGRAL
- Part of:
-
- Published online by Cambridge University Press:
- 06 February 2012, pp. 327-338
-
- Article
-
- You have access
- Export citation
ON THE DISTRIBUTION OF TORSION POINTS MODULO PRIMES
- Part of:
-
- Published online by Cambridge University Press:
- 16 February 2012, pp. 339-347
-
- Article
-
- You have access
- Export citation
ON THE DIOPHANTINE EQUATION (8n)x+(15n)y=(17n)z
- Part of:
-
- Published online by Cambridge University Press:
- 07 February 2012, pp. 348-352
-
- Article
-
- You have access
- Export citation
Front Cover (OFC, IFC) and matter
BAZ VOLUME 86 ISSUE 2 COVER AND FRONT MATTER
-
- Published online by Cambridge University Press:
- 07 September 2012, pp. f1-f2
-
- Article
-
- You have access
- Export citation


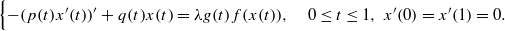
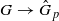 induces an isomorphism
induces an isomorphism  for any discrete
for any discrete 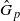 -module
-module  is an isomorphism for any discrete
is an isomorphism for any discrete 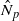 -module
-module 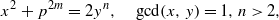
 be a commutative algebraic group defined over a number field
be a commutative algebraic group defined over a number field  , we determine the average values of
, we determine the average values of