Stochastic Geometry and Statistical Applications
On the connectivity and diameter of small-world networks
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 853-863
-
- Article
-
- You have access
- Export citation
On weak stationarity and weak isotropy of processes of convex bodies and cylinders
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 864-882
-
- Article
-
- You have access
- Export citation
On the correlation between the volumes of the typical Poisson-Voronoi cell and the typical Stienen sphere
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 883-892
-
- Article
-
- You have access
- Export citation
Bartlett spectrum and mixing properties of infinitely divisible random measures
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 893-897
-
- Article
-
- You have access
- Export citation
General Applied Probability
Congestion-dependent pricing in a stochastic service system
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 898-921
-
- Article
-
- You have access
- Export citation
Graphs with specified degree distributions, simple epidemics, and local vaccination strategies
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 922-948
-
- Article
-
- You have access
- Export citation
An epidemic model with infector-dependent severity
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 949-972
-
- Article
-
- You have access
- Export citation
Perfectly random sampling of truncated multinormal distributions
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 973-990
-
- Article
-
- You have access
- Export citation
Success run statistics defined on an urn model
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 991-1019
-
- Article
-
- You have access
- Export citation
Wave intensities and slopes in Lagrangian seas
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 1020-1035
-
- Article
-
- You have access
- Export citation
Transformations of Galton-Watson processes and linear fractional reproduction
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 1036-1053
-
- Article
-
- You have access
- Export citation
Functional limit theorems for critical processes with immigration
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 1054-1069
-
- Article
-
- You have access
- Export citation
Uniform renewal theory with applications to expansions of random geometric sums
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 1070-1097
-
- Article
-
- You have access
- Export citation
Optimal boarding policies for thin passengers
- Part of:
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 1098-1114
-
- Article
-
- You have access
- Export citation
Correction
Correction
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 1115-1116
-
- Article
-
- You have access
- Export citation
Other
Advances in Applied Probability Volume 39 (2007): Index: General Applied Probability
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. 1117-1118
-
- Article
-
- You have access
- Export citation
Advances in Applied Probability Volume 39 (2007): Index: Stochastic Geometry and Statistical Applications
-
- Published online by Cambridge University Press:
- 01 July 2016, p. 1119
-
- Article
-
- You have access
- Export citation
Front matter
APR volume 39 issue 4 Cover and Front matter
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. f1-f6
-
- Article
-
- You have access
- Export citation
Back matter
APR volume 39 issue 4 Cover and Back matter
-
- Published online by Cambridge University Press:
- 01 July 2016, pp. b1-b2
-
- Article
-
- You have access
- Export citation

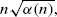 where α(
where α(