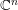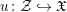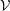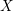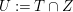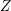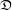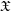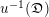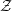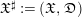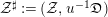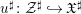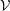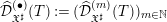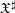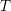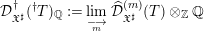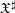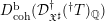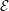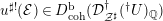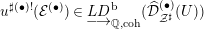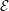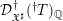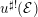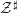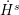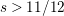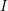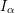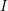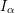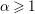The relationship between interpolation and separation properties of hypersurfaces in Bargmann–Fock spaces over \mathbb{C}^{n}$\mathbb{C}^{n}$ is not well understood except for n=1$n=1$. We present four examples of smooth affine algebraic hypersurfaces that are not uniformly flat, and show that exactly two of them are interpolating.

Soit \unicode[STIX]{x1D70B}$\unicode[STIX]{x1D70B}$ un module de plus haut poids unitaire du groupe G=\mathbf{Sp}(2n,\mathbb{R})$G=\mathbf{Sp}(2n,\mathbb{R})$. On s’intéresse aux paquets d’Arthur contenant \unicode[STIX]{x1D70B}$\unicode[STIX]{x1D70B}$. Lorsque le plus haut poids est scalaire, on détermine les paramètres de ces paquets, on établit la propriété de multiplicité 1$1$ de \unicode[STIX]{x1D70B}$\unicode[STIX]{x1D70B}$ dans le paquet, et l’on calcule le caractère \unicode[STIX]{x1D70C}_{\unicode[STIX]{x1D70B}}$\unicode[STIX]{x1D70C}_{\unicode[STIX]{x1D70B}}$ (du groupe des composantes connexes du centralisateur du paramètre dans le groupe dual) associé à \unicode[STIX]{x1D70B}$\unicode[STIX]{x1D70B}$ et qui joue un grand rôle dans la théorie d’Arthur. On fait de même pour certains modules de plus haut poids unitaires unipotents \unicode[STIX]{x1D70E}_{n,k}$\unicode[STIX]{x1D70E}_{n,k}$, ou bien lorsque le caractère infinitésimal est régulier.

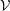Let {\mathcal{V}}${\mathcal{V}}$ be a complete discrete valuation ring of unequal characteristic with perfect residue field, 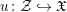u:{\mathcal{Z}}{\hookrightarrow}\mathfrak{X}$u:{\mathcal{Z}}{\hookrightarrow}\mathfrak{X}$ be a closed immersion of smooth, quasi-compact, separated formal schemes over 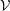{\mathcal{V}}${\mathcal{V}}$, T$T$ be a divisor of 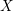X$X$ such tha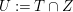t U:=T\cap Z$U:=T\cap Z$ is a divisor of 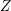Z$Z$, and 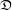\mathfrak{D}$\mathfrak{D}$ a strict normal crossing divisor of 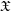\mathfrak{X}$\mathfrak{X}$ such that 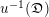u^{-1}(\mathfrak{D})$u^{-1}(\mathfrak{D})$ is a strict normal crossing divisor of 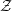{\mathcal{Z}}${\mathcal{Z}}$. We pose 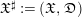\mathfrak{X}^{\sharp }:=(\mathfrak{X},\mathfrak{D})$\mathfrak{X}^{\sharp }:=(\mathfrak{X},\mathfrak{D})$, {\mathcal{Z}}^{\sharp }:=({\mathcal{Z}},u^{-1}\mathfrak{D})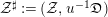${\mathcal{Z}}^{\sharp }:=({\mathcal{Z}},u^{-1}\mathfrak{D})$ and 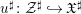u^{\sharp }:{\mathcal{Z}}^{\sharp }{\hookrightarrow}\mathfrak{X}^{\sharp }$u^{\sharp }:{\mathcal{Z}}^{\sharp }{\hookrightarrow}\mathfrak{X}^{\sharp }$ the exact closed immersion of smooth logarithmic formal schemes over 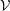{\mathcal{V}}${\mathcal{V}}$. In Berthelot’s theory of arithmetic {\mathcal{D}}${\mathcal{D}}$-modules, we work with the inductive system of sheaves of rings 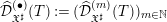\widehat{{\mathcal{D}}}_{\mathfrak{X}^{\sharp }}^{(\bullet )}(T):=(\widehat{{\mathcal{D}}}_{\mathfrak{X}^{\sharp }}^{(m)}(T))_{m\in \mathbb{N}}$\widehat{{\mathcal{D}}}_{\mathfrak{X}^{\sharp }}^{(\bullet )}(T):=(\widehat{{\mathcal{D}}}_{\mathfrak{X}^{\sharp }}^{(m)}(T))_{m\in \mathbb{N}}$, where \widehat{{\mathcal{D}}}_{\mathfrak{X}^{\sharp }}^{(m)}$\widehat{{\mathcal{D}}}_{\mathfrak{X}^{\sharp }}^{(m)}$ is the 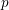p$p$-adic completion of the ring of differential operators of level m$m$ over 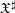\mathfrak{X}^{\sharp }$\mathfrak{X}^{\sharp }$ and where T$T$ means that we add overconvergent singularities along the divisor 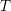T$T$. Moreover, Berthelot introduced the sheaf 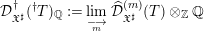{\mathcal{D}}_{\mathfrak{X}^{\sharp }}^{\dagger }(\text{}^{\dagger }T)_{\mathbb{Q}}:=\underset{\underset{m}{\longrightarrow }}{\lim }\,\widehat{{\mathcal{D}}}_{\mathfrak{X}^{\sharp }}^{(m)}(T)\otimes _{\mathbb{Z}}\mathbb{Q}${\mathcal{D}}_{\mathfrak{X}^{\sharp }}^{\dagger }(\text{}^{\dagger }T)_{\mathbb{Q}}:=\underset{\underset{m}{\longrightarrow }}{\lim }\,\widehat{{\mathcal{D}}}_{\mathfrak{X}^{\sharp }}^{(m)}(T)\otimes _{\mathbb{Z}}\mathbb{Q}$ of differential operators over 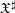\mathfrak{X}^{\sharp }$\mathfrak{X}^{\sharp }$ of finite level with overconvergent singularities along T$T$. Let {\mathcal{E}}^{(\bullet )}\in \underset{\displaystyle \longrightarrow }{LD}\text{}_{\mathbb{Q},\text{coh}}^{\text{b}}(\widehat{{\mathcal{D}}}_{\mathfrak{X}^{\sharp }}^{(\bullet )}(T))${\mathcal{E}}^{(\bullet )}\in \underset{\displaystyle \longrightarrow }{LD}\text{}_{\mathbb{Q},\text{coh}}^{\text{b}}(\widehat{{\mathcal{D}}}_{\mathfrak{X}^{\sharp }}^{(\bullet )}(T))$ and {\mathcal{E}}:=\varinjlim ~({\mathcal{E}}^{(\bullet )})${\mathcal{E}}:=\varinjlim ~({\mathcal{E}}^{(\bullet )})$ be the corresponding object of 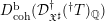D_{\text{coh}}^{\text{b}}({\mathcal{D}}_{\mathfrak{X}^{\sharp }}^{\dagger }(\text{}^{\dagger }T)_{\mathbb{Q}})$D_{\text{coh}}^{\text{b}}({\mathcal{D}}_{\mathfrak{X}^{\sharp }}^{\dagger }(\text{}^{\dagger }T)_{\mathbb{Q}})$. In this paper, we study sufficient conditions on 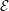{\mathcal{E}}${\mathcal{E}}$ so that if 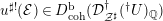u^{\sharp !}({\mathcal{E}})\in D_{\text{coh}}^{\text{b}}({\mathcal{D}}_{{\mathcal{Z}}^{\sharp }}^{\dagger }(\text{}^{\dagger }U)_{\mathbb{Q}})$u^{\sharp !}({\mathcal{E}})\in D_{\text{coh}}^{\text{b}}({\mathcal{D}}_{{\mathcal{Z}}^{\sharp }}^{\dagger }(\text{}^{\dagger }U)_{\mathbb{Q}})$ then 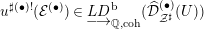u^{\sharp (\bullet )!}({\mathcal{E}}^{(\bullet )})\in \underset{\displaystyle \longrightarrow }{LD}\text{}_{\mathbb{Q},\text{coh}}^{\text{b}}(\widehat{{\mathcal{D}}}_{{\mathcal{Z}}^{\sharp }}^{(\bullet )}(U))$u^{\sharp (\bullet )!}({\mathcal{E}}^{(\bullet )})\in \underset{\displaystyle \longrightarrow }{LD}\text{}_{\mathbb{Q},\text{coh}}^{\text{b}}(\widehat{{\mathcal{D}}}_{{\mathcal{Z}}^{\sharp }}^{(\bullet )}(U))$. For instance, we check that this is the case when 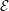{\mathcal{E}}${\mathcal{E}}$ is a coherent 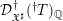{\mathcal{D}}_{\mathfrak{X}^{\sharp }}^{\dagger }(\text{}^{\dagger }T)_{\mathbb{Q}}${\mathcal{D}}_{\mathfrak{X}^{\sharp }}^{\dagger }(\text{}^{\dagger }T)_{\mathbb{Q}}$-module such that the cohomological spaces of 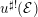u^{\sharp !}({\mathcal{E}})$u^{\sharp !}({\mathcal{E}})$ are isocrystals on 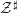{\mathcal{Z}}^{\sharp }${\mathcal{Z}}^{\sharp }$ overconvergent along U$U$.

We prove the existence of the global attractor in {\dot{H}}^{s}${\dot{H}}^{s}$, s>11/12$s>11/12$ for the weakly damped and forced mKdV on the one-dimensional torus. The existence of global attractor below the energy space has not been known, though the global well-posedness below the energy space has been established. We directly apply the I$I$-method to the damped and forced mKdV, because the Miura transformation does not work for the mKdV with damping and forcing terms. We need to make a close investigation into the trilinear estimates involving resonant frequencies, which are different from the bilinear estimates corresponding to the KdV.

We prove that the integral closure of a strongly Golod ideal in a polynomial ring over a field of characteristic zero is strongly Golod, positively answering a question of Huneke. More generally, the rational power I_{\unicode[STIX]{x1D6FC}}$I_{\unicode[STIX]{x1D6FC}}$ of an arbitrary homogeneous ideal is strongly Golod for \unicode[STIX]{x1D6FC}\geqslant 2$\unicode[STIX]{x1D6FC}\geqslant 2$ and, if I$I$ is strongly Golod, then I_{\unicode[STIX]{x1D6FC}}$I_{\unicode[STIX]{x1D6FC}}$ is strongly Golod for \unicode[STIX]{x1D6FC}\geqslant 1$\unicode[STIX]{x1D6FC}\geqslant 1$. We also show that all the coefficient ideals of a strongly Golod ideal are strongly Golod.