For a reduced hyperplane arrangement, we prove the analytic Twisted Logarithmic Comparison Theorem, subject to mild combinatorial arithmetic conditions on the weights defining the twist. This gives a quasi-isomorphism between the twisted logarithmic de Rham complex and the twisted meromorphic de Rham complex. The latter computes the cohomology of the arrangement’s complement with coefficients from the corresponding rank one local system. We also prove the algebraic variant (when the arrangement is central), and the analytic and algebraic (untwisted) Logarithmic Comparison Theorems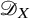. The last item positively resolves an old conjecture of Terao. We also prove that: Every nontrivial rank one local system on the complement can be computed via these Twisted Logarithmic Comparison Theorems; these computations are explicit finite-dimensional linear algebra. Finally, we give some $\mathscr {D}_{X}$-module applications: For example, we give a sharp restriction on the codimension one components of the multivariate Bernstein–Sato ideal attached to an arbitrary factorization of an arrangement. The bound corresponds to (and, in the univariate case, gives an independent proof of) M. Saito’s result that the roots of the Bernstein–Sato polynomial of a non-smooth, central, reduced arrangement live in $(-2 + 1/d, 0).$

We show the thinness of $7$ of the $40$ hypergeometric groups ha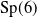ving a maximally unipotent monodromy in $\mathrm{Sp}(6)$.

We develop the theory of relative regular holonomic $\mathcal {D}$-modules with a smooth complex manifold $S$ of arbitrary dimension as parameter space, together with their main functorial properties. In particular, we establish in this general setting the relative Riemann–Hilbert correspondence proved in a previous work in the one-dimensional case.

We define Bernstein–Sato polynomials for meromorphic functions and study their basic properties. In particular, we prove a Kashiwara–Malgrange-type theorem on their geometric monodromies, which would also be useful in relation with the monodromy conjecture. A new feature in the meromorphic setting is that we have several b-functions whose roots yield the same set of the eigenvalues of the Milnor monodromies. We also introduce multiplier ideal sheaves for meromorphic functions and show that their jumping numbers are related to our b-functions.

We study log $\mathscr {D}$-modules on smooth log pairs and construct a comparison theorem of log de Rham complexes. The proof uses Sabbah’s generalized b-functions. As applications, we deduce a log index theorem and a Riemann-Roch type formula for perverse sheaves on smooth quasi-projective varieties. The log index theorem naturally generalizes the Dubson-Kashiwara index theorem on smooth projective varieties.

We introduce the notion of regularity for a relative holonomic ${\mathcal{D}}$-module in the sense of Monteiro Fernandes and Sabbah [Internat. Math. Res. Not. (21) (2013), 4961–4984]. We prove that the solution functor from the bounded derived category of regular relative holonomic modules to that of relative constructible complexes is essentially surjective by constructing a right quasi-inverse functor. 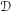When restricted to relative ${\mathcal{D}}$-modules underlying a regular mixed twistor ${\mathcal{D}}$-module, this functor satisfies the left quasi-inverse property.

Given a mixed Hodge module $\mathcal{N}$ and a meromorphic function $f$ on a complex manifold, we associate to these data a filtration (the irregular Hodge filtration) on the exponentially twisted holonomic module $\mathcal{N}\otimes \mathcal{E}^{f}$, which extends the construction of Esnault et al. ($E_{1}$-degeneration of the irregular Hodge filtration (with an appendix by Saito), J. reine angew. Math. (2015), doi:10.1515/crelle-2014-0118). We show the strictness of the push-forward filtered ${\mathcal{D}}$-module through any projective morphism ${\it\pi}:X\rightarrow Y$, by using the theory of mixed twistor ${\mathcal{D}}$-modules of Mochizuki. We consider the example of the rescaling of a regular function $f$, which leads to an expression of the irregular Hodge filtration of the Laplace transform of the Gauss–Manin systems of $f$ in terms of the Harder–Narasimhan filtration of the Kontsevich bundles associated with $f$.

We consider the system F4 (a, b, c) of differential equations annihilating Appell's hypergeometric series F4(a,b,c;x). We find the integral representations for four linearly independent solutions expressed by the hypergeometric series F4. By using the intersection forms of twisted (co)homology groups associated with them, we provide the monodromy representation of F4(a, b, c) and the twisted period relations for the fundamental systems of solutions of F4.

We describe a general setting where the monodromy action on the first cohomology group of the Milnor fiber of a hyperplane arrangement is the identity.

We endow certain GKZ-hypergeometric systems with a natural structure of a mixed Hodge module, which is compatible with the mixed Hodge module structure on the Gauß–Manin system of an associated family of Laurent polynomials. As an application we show that the underlying perverse sheaf of a GKZ-system with rational parameter has quasi-unipotent local monodromy.

We provide certain unusual generalizations of Clausen's and Orr's theorems for solutions of fourth-order and fifth-order generalized hypergeometric equations. As an application, we present several examples of algebraic transformations of Calabi–Yau differential equations.

Let X be a complete, geometrically irreducible, singular, algebraic curve defined over a field of characteristic p big enough. Given a local ring Op,x at a rational singular point P of X, we attached a universal zeta function which is a rational function and admits a functional equation if Op,x is Gorenstein. This universal zeta function specializes to other known zeta functions and Poincaré series attached to singular points of algebraic curves. In particular, for the local ring attached to a complex analytic function in two variables, our universal zeta function specializes to the generalized Poincaré series introduced by Campillo, Delgado, and Gusein-Zade.

We introduce the notion of an alternate product of Frobenius manifolds and we give, after Ciocan-Fontanine et al., an interpretation of the Frobenius manifold structure canonically attached to the quantum cohomology of G(r,n+1) in terms of alternate products. We also investigate the relationship with the alternate Thom–Sebastiani product of Laurent polynomials.

We introduce the notion of the Bernstein–Sato polynomial of an arbitrary variety (which is not necessarily reduced nor irreducible) using the theory of V-filtrations of M. Kashiwara and B. Malgrange. We prove that the decreasing filtration by multiplier ideals coincides essentially with the restriction of the V-filtration. This implies a relation between the roots of the Bernstein–Sato polynomial and the jumping coefficients of the multiplier ideals, and also a criterion for rational singularities in terms of the maximal root of the polynomial in the case of a reduced complete intersection. These are generalizations of the hypersurface case. We can calculate the polynomials explicitly in the case of monomial ideals.

Let $Q\in{\mathbb C}[x_1,\dotsc,x_n]$ be a homogeneous polynomial of degree $k>0$. We establish a connection between the Bernstein–Sato polynomial $b_Q(s)$ and the degrees of the generators for the top cohomology of the associated Milnor fiber. In particular, the integer $u_Q={\rm max}\{i\in{\mathbb Z}:b_Q(-(i+n)/k)=0\}$ bounds the top degree (as differential form) of the elements in $H^{n-1}_{\rm DR}(Q^{-1}(1),{\mathbb C})$. The link is provided by the relative de Rham complex and ${\mathcal D}$-module algorithms for computing integration functors.

As an application we determine the Bernstein–Sato polynomial $b_Q(s)$ of a generic central arrangement $Q=\prod_{i=1}^kH_i$ of hyperplanes. In turn, we obtain information about the cohomology of the Milnor fiber of such arrangements related to results of Orlik and Randell who investigated the monodromy.

We also introduce certain subschemes of the arrangement determined by the roots of $b_Q(s)$. They appear to correspond to iterated singular loci.

In this paper, we give a formal algebraic notion of exponents for linear differential systems at any singularity as eigenvalues of the residue of a regular connection on a maximal lattice (that we call ‘Levelt's lattice’). This allows us to establish upper and lower bounds for the sum of these exponents for differential systems on ${\mathbb P}^{1}(\mathbb{C})$.

We prove a generalization to the context of real geometry of an intersection formula for the vanishing cycle functor, which in the complex context is due to Dubson, Lê, Ginsburg and Sabbah (after a conjecture of Deligne). It is also a generalization of similar results of Kashiwara and Schapira, where these authors work with a suitable assumption about the micro-support of the corresponding constructible complex of sheaves. We only use a similar assumption about the support of the corresponding characteristic cycle so that our result can be formulated in the language of constructible functions and Lagrangian cycles.

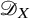
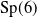
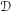
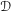
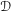
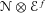
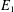
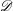
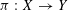
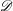
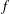
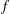
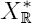Let Xℝ ⊂ ℝN a real analytic set such that its complexification Xℂ ⊂ ℂN is normal with an isolated singularity at 0. Let fℝ : Xℝ → ℝ a real analytic function such that its complexification fℂ : Xℂ → ℂ has an isolated singularity at 0 in Xℂ. Assuming an orientation given on to a connected component A of we associate a compact cycle Γ(A) in the Milnor fiber of fℂ which determines completely the poles of the meromorphic extension of or equivalently the asymptotics when T → ±∞ of the oscillating integrals . 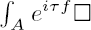A topological construction of Γ(A) is given. This completes the results of [BM] paragraph 6.