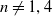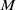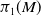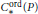7 results
Simplicial volume of manifolds with amenable fundamental group at infinity
- Part of
-
- Journal:
- Glasgow Mathematical Journal / Volume 66 / Issue 3 / September 2024
- Published online by Cambridge University Press:
- 22 April 2024, pp. 563-570
- Print publication:
- September 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Topological volumes of fibrations: a note on open covers
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 152 / Issue 5 / October 2022
- Published online by Cambridge University Press:
- 11 October 2021, pp. 1340-1360
- Print publication:
- October 2022
-
- Article
- Export citation
A NOTE ON p-ADIC SIMPLICIAL VOLUMES
- Part of
-
- Journal:
- Glasgow Mathematical Journal / Volume 63 / Issue 3 / September 2021
- Published online by Cambridge University Press:
- 13 August 2020, pp. 563-583
- Print publication:
- September 2021
-
- Article
-
- You have access
- Open access
- Export citation
EXOTIC FINITE FUNCTORIAL SEMI-NORMS ON SINGULAR HOMOLOGY
- Part of
-
- Journal:
- Glasgow Mathematical Journal / Volume 61 / Issue 2 / May 2019
- Published online by Cambridge University Press:
- 20 June 2018, pp. 287-295
- Print publication:
- May 2019
-
- Article
-
- You have access
- Export citation
ON THE COHOMOLOGY OF CERTAIN QUOTIENTS OF THE SPECTRUM BP
-
- Journal:
- Glasgow Mathematical Journal / Volume 54 / Issue 1 / January 2012
- Published online by Cambridge University Press:
- 02 August 2011, pp. 61-66
- Print publication:
- January 2012
-
- Article
-
- You have access
- Export citation
Monoidal Functors, Acyclic Models and Chain Operads
-
- Journal:
- Canadian Journal of Mathematics / Volume 60 / Issue 2 / 01 April 2008
- Published online by Cambridge University Press:
- 20 November 2018, pp. 348-378
- Print publication:
- 01 April 2008
-
- Article
-
- You have access
- Export citation
Cohomology of Complex Projective Stiefel Manifolds
-
- Journal:
- Canadian Journal of Mathematics / Volume 51 / Issue 5 / 01 October 1999
- Published online by Cambridge University Press:
- 20 November 2018, pp. 897-914
- Print publication:
- 01 October 1999
-
- Article
-
- You have access
- Export citation