We show that self-similar measures on $\mathbb R^d$ satisfying the weak separation condition are uniformly scaling. Our approach combines elementary ergodic theory with geometric analysis of the structure given by the weak separation condition.

Suppose that 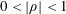$0<|\unicode[STIX]{x1D70C}|<1$ and $m\geqslant 2$ is an integer. Let 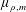$\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D70C},m}$ be the self-similar measure defined by $\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D70C},m}(\cdot )=\frac{1}{m}\sum _{j=0}^{m-1}\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D70C},m}(\unicode[STIX]{x1D70C}^{-1}(\cdot )-j)$. 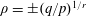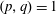Assume that $\unicode[STIX]{x1D70C}=\pm (q/p)^{1/r}$ for some $p,q,r\in \mathbb{N}^{+}$ with $(p,q)=1$ and 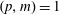$(p,m)=1$. We prove that if 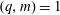$(q,m)=1$, then there are at most $m$ mutually orthogonal exponential functions in 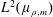$L^{2}(\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D70C},m})$ and $m$ is the best possible. If 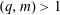$(q,m)>1$, then there are any number of orthogonal exponential functions in $L^{2}(\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D70C},m})$.

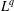For the class of self-similar measures in $\mathbb{R}^{d}$ with overlaps that are essentially of finite type, we set up a framework for deriving a closed formula for the $L^{q}$-spectrum of the measure for $q\geq 0$. This framework allows us to include iterated function systems that have different contraction ratios and those in higher dimension. For self-similar measures with overlaps, closed formulas for the $L^{q}$-spectrum have only been obtained earlier for measures satisfying Strichartz’s second-order identities. We illustrate how to use our results to prove the differentiability of the $L^{q}$-spectrum, obtain the multifractal dimension spectrum, and compute the Hausdorff dimension of the measure.

We set up a framework for computing the spectral dimension of a class of one-dimensional self-similar measures that are defined by iterated function systems with overlaps and satisfy a family of second-order self-similar identities. As applications of our result we obtain the spectral dimension of important measures such as the infinite Bernoulli convolution associated with the golden ratio and convolutions of Cantor-type measures. The main novelty of our result is that the iterated function systems we consider are not post-critically finite and do not satisfy the well-known open set condition.

Start with a compact set K ⊂ Rd. This has a random number of daughter sets, each of which is a (rotated and scaled) copy of K and all of which are inside K. The random mechanism for producing daughter sets is used independently on each of the daughter sets to produce the second generation of sets, and so on, repeatedly. The random fractal set F is the limit, as n goes to ∞, of the union of the nth generation sets. In addition, K has a (suitable, random) mass which is divided randomly between the daughter sets, and this random division of mass is also repeated independently, indefinitely. This division of mass will correspond to a random self-similar measure on F. The multifractal spectrum of this measure is studied here. Our main contributions are dealing with the geometry of realisations in Rd and drawing systematically on known results for general branching processes. In this way we generalise considerably the results of Arbeiter and Patzschke (1996) and Patzschke (1997).

We introduce a class of stochastic processes in discrete time with finite state space by means of a simple matrix product. We show that this class coincides with that of the hidden Markov chains and provides a compact framework for it. We study a measure obtained by a projection on the real line of the uniform measure on the Sierpinski gasket, finding that the dimension of this measure fits with the Shannon entropy of an associated hidden Markov chain.