We consider a viscous drop, loaded with an insoluble surfactant, spreading over an inclined plane that is covered initially with a thin surfactant-free liquid film. Lubrication theory is employed to model the flow using coupled nonlinear evolution equations for the film thickness and surfactant concentration. Exploiting high-resolution numerical simulations, we describe the late-time multi-region asymptotic structure of the spatially one-dimensional spreading flow. A simplified differential–algebraic equation model is derived for key variables characterising the spreading process, using which the late-time spreading and thinning rates are determined. Focusing on the neighbourhood of the drop’s leading-edge effective contact line, we then examine the stability of this region to small-amplitude disturbances with transverse variation. A dispersion relationship is described using long-wavelength asymptotics and numerical simulations, which reveals physical mechanisms and new scaling properties of the instability.

A theoretical and numerical investigation of two classes of models for pressure-driven core–annular flow is presented. Both classes, referred to as ‘long-wave’ and ‘thin-film’ models, may be derived from a unified perspective using long-wave asymptotics, but are distinct from one another in the role played by the curved tube geometry with respect to the planar (limiting) case. Analytical and numerical techniques are used to show and quantify the significant differences between the behaviour of solutions to both model types. Temporal linear stability analysis of the constant solution is carried out first to pinpoint with closed-form mathematical expressions the different dynamical regimes associated with absolute or convective instabilities. Numerical simulations for the models are then performed and qualitative differences in the evolution of the free surface are explored. Mathematically, different levels of asymptotic accuracy are found to result in different regularizing properties affecting the long-time behaviour of generic numerical solutions. Travelling wave solutions are also studied, and qualitative differences in the topology of streamline patterns describing the flow of the film in a moving reference frame are discussed. These topological differences allow for further classification of the models. In particular, a transition from a regime in which waves trap a fluid core to one where waves travel faster than any parcel of the underlying fluid is documented for a variant of the primary model. In the corresponding thin-film model, no such transition is found to occur. The source of these differences is examined, and a comparison of the results with those of related models in the literature is given. A brief discussion of the merits of each class of models concludes this study.

The sedimentation of a rigid particle near a wall in a viscous fluid has been studied numerically by many authors, but analytical solutions have been derived only for special cases such as the motion of spherical particles. In this paper the method of images is used to derive simple ordinary differential equations describing the sedimentation of arbitrarily oriented prolate and oblate spheroids at zero Reynolds number near a vertical or inclined plane wall. The differential equations may be solved analytically in many situations, and full trajectories are predicted which compare favourably with complete numerical simulations. The simulations are performed using a novel double-layer boundary integral formulation, a method of stresslet images. The conditions under which the glancing and reversing trajectories, first observed by Russel et al. (J. Fluid Mech., vol. 83, 1977, pp. 273–287), occur are studied for bodies of arbitrary aspect ratio. Several additional trajectories are also described: a periodic tumbling trajectory for nearly spherical bodies, a linearly stable sliding trajectory which appears when the wall is slightly inclined, and three-dimensional glancing, reversing and wobbling.

When a drop impacts a smooth, dry surface at a velocity above the so-called critical speed for drop splashing, the initial liquid volume loses its integrity, fragmenting into tiny droplets that are violently ejected radially outwards. Here, we make use of the model of Riboux & Gordillo (Phys. Rev. Lett., vol. 113, 2014, 024507), together with a one-dimensional approximation describing the flow in the ejected liquid sheet and of balances of mass and momentum at the border of the sheet, to calculate mean sizes and velocities of the ejected drops. The predictions of the model are in good agreement with experiments.

We study the effects of having an opening in a vertical cylinder of an arbitrary cross-sectional shape when subjected to incident waves from the outside field. Both the diffraction and radiation problems of linear potential-flow theory are addressed. The cylinders considered are bottom-mounted with vanishing thickness and the problem is formulated as a hypersingular boundary-integral method. A simple higher-order procedure is presented to handle the strong singularity. Comparisons are made between the hydrodynamic properties of open and closed cylinders, and the effects of increasing the opening size are discussed and explained. Results for square, circular and elliptical open cylindrical shells, presented as examples, indicate that wave loads on the structures could be dramatically decreased to zero (effectively) at certain frequencies and opening sizes. This leads to the surprising conclusion that directing an open structure into the incident-wave field results in lower loads on the structure. A model of an open harbour with a frontal breakwater in a wave field, inspired by the idea of the Portunus Project, is also analysed.

Since the pioneering work of Richardson in 1926, later refined by Batchelor and Obukhov in 1950, it is predicted that the rate of separation of pairs of fluid elements in turbulent flows with initial separation at inertial scales, grows ballistically first (Batchelor regime), before undergoing a transition towards a super-diffusive regime where the mean-square separation grows as $t^{3}$ (Richardson regime). Richardson empirically interpreted this super-diffusive regime in terms of a non-Fickian process with a scale-dependent diffusion coefficient (the celebrated Richardson’s ‘$4/3$rd’ law). However, the actual physical mechanism at the origin of such a scale dependent diffusion coefficient remains unclear. The present article proposes a simple physical phenomenology for the time evolution of the mean-square relative separation in turbulent flows, based on a scale-dependent ballistic scenario rather than a scale-dependent diffusive. It is shown that this phenomenology accurately retrieves most of the known features of relative dispersion for particles mean-square separation, among others: (i) it is quantitatively consistent with most recent numerical simulations and experiments for mean-square separation between particles (both for the short-term Batchelor regime and the long-term Richardson regime, and for all initial separations at inertial scales); (ii) it gives a simple physical explanation of the origin of the super-diffusive $t^{3}$ Richardson regime which naturally builds itself as an iterative process of elementary short-term scale-dependent ballistic steps; (iii) it shows that the Richardson constant is directly related to the Kolmogorov constant (and eventually to a ballistic persistence parameter); and (iv) in a further extension of the phenomenology, taking into account third-order corrections, it robustly describes the temporal asymmetry between forward and backward dispersion, with an explicit connection to the cascade of energy flux across scales. An important aspect of this phenomenology is that it simply and robustly connects long-term super-diffusive features to elementary short-term mechanisms, and at the same time it connects basic Lagrangian features of turbulent relative dispersion (both at short and long times) to basic Eulerian features of the turbulent field: second-order Eulerian statistics control the growth of separation (both at short and long times) while third-order Eulerian statistics control the temporal asymmetry of the dispersion process, which can then be directly identified as the signature of the energy cascade and associated to well-known exact results as the Karman–Howarth–Monin relation.

The evaporation of non-axisymmetric sessile drops is studied by means of experiments and three-dimensional direct numerical simulations (DNS). The emergence of azimuthal currents and pairs of counter-rotating vortices in the liquid bulk flow is reported in drops with non-circular contact area. These phenomena, especially the latter, which is also observed experimentally, are found to play a critical role in the transient flow dynamics and associated heat transfer. Non-circular drops exhibit variable wettability along the pinned contact line sensitive to the choice of system parameters, and inversely dependent on the local contact-line curvature, providing a simple criterion for estimating the approximate contact-angle distribution. The evaporation rate is found to vary in the same order of magnitude as the liquid–gas interfacial area. Furthermore, the more complex case of drops evaporating with a moving contact line (MCL) in the constant contact-angle mode is addressed. Interestingly, the numerical results demonstrate that the average interface temperature remains essentially constant as the drop evaporates in the constant-angle (CA) mode, while this increases in the constant-radius (CR) mode as the drops become thinner. It is therefore concluded that, for increasing substrate heating, the evaporation rate increases more rapidly in the CR mode than in the CA mode. In other words, the higher the temperature the larger the difference between the lifetimes of an evaporating drop in the CA mode with respect to that evaporating in the CR mode.

A novel similarity-based form is derived of the transport equation for the second-order velocity structure function of $\langle ({\it\delta}q)^{2}\rangle$ along the centreline of a round turbulent jet using an equilibrium similarity analysis. This self-similar equation has the advantage of requiring less extensive measurements to calculate the inhomogeneous (decay and production) terms of the transport equation. It is suggested that the normalised third-order structure function can be uniquely determined when the normalised second-order structure function, the power-law exponent of $\langle q^{2}\rangle$ and the decay rate constants of $\langle u^{2}\rangle$ and $\langle v^{2}\rangle$ are available. In addition, the current analysis demonstrates that the assumption of similarity, combined with an inverse relation between the mean velocity $U$ and the streamwise distance $x-x_{0}$ from the virtual origin (i.e. $U\propto (x-x_{0})^{-1}$), is sufficient to predict a power-law decay for the turbulence kinetic energy ($\langle q^{2}\rangle \propto (x-x_{0})^{m}$), rather than requiring a power-law decay ($m=-2$) as an additional ad hoc assumption. On the basis of the current analysis, it is suggested that the mean kinetic energy dissipation rate, $\langle {\it\epsilon}\rangle$, varies as $(x-x_{0})^{m-2}$. These theoretical results are tested against new experimental data obtained along the centreline of a round turbulent jet as well as previously published data on round jets for $11\,000\leqslant \mathit{Re}_{D}\leqslant 184\,000$ over the range $10\leqslant x/D\leqslant 90$. For the present experiments, $\langle q^{2}\rangle$ exhibits power-law behaviour with $m=-1.83$. The validity of this solution is confirmed using other experimental data where $\langle q^{2}\rangle$ follows a power law with $-1.89\leqslant m\leqslant -1.78$. The present similarity form of the transport equation for $\langle ({\it\delta}q)^{2}\rangle$ is also shown to be closely satisfied by the experimental data.

The present study is an experimental investigation of the relationship between the large- and small-scale motions in the far field of an air jet at high Reynolds number. In the first part of our investigation, the analysis is based on time series of hot-wire anemometry (HWA), which are converted into space series after applying the Taylor hypothesis. By using a spectral filter, two signals are constructed, one representative of the large-scale motions (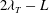$2{\it\lambda}_{T}-L$, where 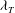${\it\lambda}_{T}$ is the Taylor length scale, and 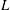$L$ is the integral length scale) and the other representative of the small-scale motions (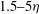$1.5{-}5{\it\eta}$, where 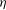${\it\eta}$ is the Kolmogorov length scale). The small-scale signal is found to be modulated both in amplitude and in frequency by the energy-containing scales in an analogous way, both at the centreline and around the centreline. In particular, for positive fluctuations of the large-scale signal, the small-scale signal is locally stronger in amplitude (amplitude modulation), and it locally exhibits a higher number of local maxima and minima (frequency modulation). The extent of this modulation is quantified based on the strength of the large-scale fluctuations. The response of the small-scale motions to amplitude modulation can be considered instantaneous, being on the order of one Kolmogorov time scale. In the second part of our investigation we use long-range 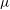${\it\mu}$PIV to study the behaviour of the small-scale motions in relation to their position in either high-speed or low-speed regions of the flow. The spatially resolved velocity vector fields allow us to quantify amplitude modulation directly in physical space. From this direct estimation in physical space, amplitude modulation is only 25 % of the value measured from HWA. The remaining 75 % comes from the fixed spectral band filter used to obtain the large- and small-scale signals, which does not consider the local convection velocity. A very similar overestimation of amplitude modulation when quantified in the time-frame is also obtained analytically. Furthermore, the size of the structures of intense vorticity does not change significantly in relation to the large-scale velocity fluctuation, meaning that there is no significant spatial frequency modulation. The remaining amplitude modulation in space can be explained as a statistical coupling between the strength of the structures of vorticity and their preferential location inside large-scale high-velocity regions. Finally, the implications that the present findings have on amplitude and frequency modulation in turbulent boundary layers (TBLs) are discussed.

Landslides plunging into lakes and reservoirs can result in extreme wave runup at the shores. This phenomenon has claimed lives and caused damage to near-shore properties. Landslide tsunamis in lakes are different from typical earthquake tsunamis in the open ocean in that (i) the affected areas are usually within the near field of the source, (ii) the highest runup occurs within the time period of the geophysical event, and (iii) the enclosed geometry of a lake does not let the tsunami energy escape. To address the problem of transient landslide tsunami runup and to predict the resulting inundation, we utilize a nonlinear model equation in the Lagrangian frame of reference. The motivation for using such a scheme lies in the fact that the runup on an inclined boundary is directly and readily computed in the Lagrangian framework without the need to resort to approximations. In this work, we investigate the inundation patterns due to landslide tsunamis in a lake. We show by numerical computations that Airy’s approximation of an irrotational theory using Lagrangian coordinates can legitimately predict runup of large amplitude. We also demonstrate that in a lake of finite size the highest runup may be magnified by constructive interference between edge waves that are trapped along the shore and multiple reflections of outgoing waves from opposite shores, and may occur somewhat after the first inundation.

Based on a similarity between piston-mode sloshing in a two-dimensional rectangular moonpool and a small-amplitude oscillatory flow through a slot in a slatted screen, a pressure discharge in the moonpool opening is theoretically quantified. This makes it possible, by changing the dynamic free-surface condition inside the moonpool, to account for the vortex-induced damping and thereby to modify the inviscid potential flow statement. The present paper is an important addition to Faltinsen et al. (J. Fluid Mech., vol. 575, 2007, pp. 359–397), where a discrepancy between the potential flow theory and model tests was reported. New calculations are supported by the earlier model tests of the authors as well as numerical data obtained by means of viscous solvers.

We report the first simulations of the Faraday instability using the full three-dimensional Navier–Stokes equations in domains much larger than the characteristic wavelength of the pattern. We use a massively parallel code based on a hybrid front-tracking/level-set algorithm for Lagrangian tracking of arbitrarily deformable phase interfaces. Simulations performed in square and cylindrical domains yield complex patterns. In particular, a superlattice-like pattern similar to those of Douady & Fauve (Europhys. Lett., vol. 6, 1988, pp. 221–226) and Douady (J. Fluid Mech., vol. 221, 1990, pp. 383–409) is observed. The pattern consists of the superposition of two square superlattices. We conjecture that such patterns are widespread if the square container is large compared with the critical wavelength. In the cylinder, pentagonal cells near the outer wall allow a square-wave pattern to be accommodated in the centre.