We examine the steady state of turbulent flows in thin layers using direct numerical simulations. It is shown that when the layer thickness is smaller than a critical height, an inverse cascade arises which leads to the formation of a steady state condensate where most of the energy is concentrated in the largest scale of the system. For layers of thickness smaller than a second critical height, the flow at steady state becomes exactly two-dimensional. The amplitude of the condensate is studied as a function of layer thickness and Reynolds number. Bi-stability and intermittent bursts are found close to the two critical points. The results are interpreted based on a mean-field three-scale model that reproduces some of the basic features of the numerical results.

Motivated by the dynamics within terrestrial bodies, we consider a rotating, strongly thermally stratified fluid within a spherical shell subject to a prescribed laterally inhomogeneous heat-flux condition at the outer boundary. Using a numerical model, we explore a broad range of three key dimensionless numbers: a thermal stratification parameter (the relative size of boundary temperature gradients to imposed vertical temperature gradients), $10^{-3}\leqslant S\leqslant 10^{4}$, a buoyancy parameter (the strength of applied boundary heat-flux anomalies), $10^{-2}\leqslant B\leqslant 10^{6}$, and the Ekman number (ratio of viscous to Coriolis forces), $10^{-6}\leqslant E\leqslant 10^{-4}$. We find both steady and time-dependent solutions and delineate the regime boundaries. We focus on steady-state solutions, for which a clear transition is found between a low $S$ regime, in which buoyancy dominates the dynamics, and a high $S$ regime, in which stratification dominates. For the low-$S$ regime, we find that the characteristic flow speed scales as $B^{2/3}$, whereas for high-$S$, the radial and horizontal velocities scale respectively as $u_{r}\sim S^{-1}$, $u_{h}\sim S^{-3/4}B^{1/4}$ and are confined within a thin layer of depth $(SB)^{-1/4}$ at the outer edge of the domain. For the Earth, if lower mantle heterogeneous structure is due principally to chemical anomalies, we estimate that the core is in the high-$S$ regime and steady flows arising from strong outer boundary thermal anomalies cannot penetrate the stable layer. However, if the mantle heterogeneities are due to thermal anomalies and the heat-flux variation is large, the core will be in a low-$S$ regime in which the stable layer is likely penetrated by boundary-driven flows.

We compute the leading-order inertial corrections to the instantaneous force acting on a rigid body moving with a time-dependent slip velocity in a linear flow field, assuming that the square root of the Reynolds number based on the fluid-velocity gradient is much larger than the Reynolds number based on the slip velocity between the body and the fluid. As a first step towards applications to dilute sheared suspensions and turbulent particle-laden flows, we seek a formulation allowing this force to be determined for an arbitrarily shaped body moving in a general linear flow. We express the equations governing the flow disturbance in a non-orthogonal coordinate system moving with the undisturbed flow and solve the problem using matched asymptotic expansions. The use of the co-moving coordinates enables the leading-order inertial corrections to the force to be obtained at any time in an arbitrary linear flow field. We then specialize this approach to compute the time-dependent force components for a sphere moving in three canonical flows: solid-body rotation, planar elongation, and uniform shear. We discuss the behaviour and physical origin of the different force components in the short-time and quasi-steady limits. Last, we illustrate the influence of time-dependent and quasi-steady inertial effects by examining the sedimentation of prolate and oblate spheroids in a pure shear flow.

The impact of viscoplastic droplets on a free surface of water is studied. The droplet undergoes an elasto-plastic deformation at the early stages of water entry. At large time, the yield stress dominates; therefore, the droplet solidifies and reaches an equilibrium shape. Depending on the impact velocity and the rheology of the droplet, the final morphologies vary from pear-shaped to capsules that contain bubbles. We perform an analysis of the orders of magnitude of the forces and introduce the relevant dimensionless groups. Furthermore, we categorize the final shapes in a phase diagram and analyse their geometrical properties. The process presents a method of making non-spherical beads and capsules with tunable shapes and provides information on the general problem of the impact of highly deformable objects on a liquid surface.

An extension of proper orthogonal decomposition is applied to the wall layer of a turbulent channel flow ($Re_{\unicode[STIX]{x1D70F}}=590$), so that empirical eigenfunctions are defined in both space and time. Due to the statistical symmetries of the flow, the eigenfunctions are associated with individual wavenumbers and frequencies. Self-similarity of the dominant eigenfunctions, consistent with wall-attached structures transferring energy into the core region, is established. The most energetic modes are characterized by a fundamental time scale in the range 200–300 viscous wall units. The full spatio-temporal decomposition provides a natural measure of the convection velocity of structures, with a characteristic value of 12$u_{\unicode[STIX]{x1D70F}}$ in the wall layer. Finally, we show that the energy budget can be split into specific contributions for each mode, which provides a closed-form expression for nonlinear effects.

The multi-point Monin–Obukhov similarity (MMO) was recently proposed (Tong & Nguyen, J. Atmos. Sci., vol. 72, 2015, pp. 4337–4348) to address the issue of incomplete similarity in the framework of the original Monin–Obukhov similarity theory (MOST). MMO hypothesizes the following: (1) The surface-layer turbulence, defined to consist of eddies that are entirely inside the surface layer, has complete similarity, which however can only be represented by multi-point statistics, requiring a horizontal characteristic length scale (absent in MOST). (2) The Obukhov length 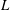$L$ is also the characteristic horizontal length scale; therefore, all surface-layer multi-point statistics, non-dimensionalized using the surface-layer parameters, depend only on the height and separations between the points, non-dimensionalized using $L$. However, similar to MOST, MMO was also proposed as a hypothesis based on phenomenology. In this work we derive MMO analytically for the case of the horizontal Fourier transforms of the velocity and potential temperature fluctuations, which are equivalent to the two-point horizontal differences of these variables, using the spectral forms of the Navier–Stokes and the potential temperature equations. We show that, for the large-scale motions (wavenumber $k<1/z$) in a convective surface layer, the solution is uniformly valid with respect to $z$ (i.e. 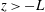as $z$ decreases from $z>-L$ to $z<-L$), where $z$ is the height from the surface. However, for 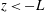$z<-L$ the solution is not uniformly valid with respective to 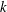$k$ as it increases from $k<-1/L$ to $k>-1/L$, resulting in a singular perturbation problem, which we analyse using the method of matched asymptotic expansions. We show that (1) 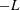$-L$ is the characteristic horizontal length scale, and (2) the Fourier transforms satisfy MMO with the non-dimensional wavenumber 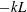$-kL$ as the independent similarity variable. Two scaling ranges, the convective range and the dynamic range, discovered for 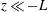$z\ll -L$ in Tong & Nguyen (2015) are obtained. We derive the leading-order spectral scaling exponents for the two scaling ranges and the corrections to the scaling ranges for finite ratios of the length scales. The analysis also reveals the dominant dynamics in each scaling range. The analytical derivations of the characteristic horizontal length scale (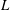$L$) and the validity of MMO for the case of two-point horizontal separations provide strong support to MMO for general multi-point velocity and temperature differences.

Pressure losses and mixing in vibrating channels were analysed. The vibrations in the form of long-wavelength travelling waves were considered. Significant reduction of pressure losses can be achieved using sufficiently fast waves propagating downstream, while significant increase of such losses is generated by waves propagating upstream. The mechanisms responsible for pressure losses were identified and discussed. The interaction of the pressure field with the waves can create a force which assists the fluid movement. A similar force can be created by friction, but only under conditions leading to flow separation. An analysis of particle trajectories was carried out to determine the effect of vibrations on mixing. A significant transverse particle movement takes place, including particle trajectories with back loops. The downstream-propagating out-of-the phase waves provide a large reduction of pressure gradient and significant potential for mixing intensification. Analysis of energy requirements demonstrates that it is possible to identify waves which reduce power requirements, i.e. the cost of actuation is smaller than the energy savings associated with the reduction of pressure gradient. The fast forward moving waves provide an opportunity for the development of alternative propulsion methods which can be more efficient than methods based on the pressure difference.

Three methods are evaluated to estimate the streamwise velocity fluctuations of a zero-pressure-gradient turbulent boundary layer of momentum-thickness-based Reynolds number up to $Re_{\unicode[STIX]{x1D703}}\simeq 8200$, using as input velocity fluctuations at different wall-normal positions. A system identification approach is considered where large-eddy simulation data are used to build single and multiple-input linear and nonlinear transfer functions. Such transfer functions are then treated as convolution kernels and may be used as models for the prediction of the fluctuations. Good agreement between predicted and reference data is observed when the streamwise velocity in the near-wall region is estimated from fluctuations in the outer region. Both the unsteady behaviour of the fluctuations and the spectral content of the data are properly predicted. It is shown that approximately 45 % of the energy in the near-wall peak is linearly correlated with the outer-layer structures, for the reference case $Re_{\unicode[STIX]{x1D703}}=4430$. These identified transfer functions allow insight into the causality between the different wall-normal locations in a turbulent boundary layer along with an estimation of the tilting angle of the large-scale structures. Differences in accuracy of the methods (single- and multiple-input linear and nonlinear) are assessed by evaluating the coherence of the structures between wall-normally separated positions. It is shown that the large-scale fluctuations are coherent between the outer and inner layers, by means of an interactions which strengthens with increasing Reynolds number, whereas the finer-scale fluctuations are only coherent within the near-wall region. This enables the possibility of considering the wall-shear stress as an input measurement, which would more easily allow the implementation of these methods in experimental applications. A parametric study was also performed by evaluating the effect of the Reynolds number, wall-normal positions and input quantities considered in the model. Since the methods vary in terms of their complexity for implementation, computational expense and accuracy, the technique of choice will depend on the application under consideration. We also assessed the possibility of designing and testing the models at different Reynolds numbers, where it is shown that the prediction of the near-wall peak from wall-shear-stress measurements is practically unaffected even for a one order of magnitude change in the corresponding Reynolds number of the design and test, indicating that the interaction between the near-wall peak fluctuations and the wall is approximately Reynolds-number independent. Furthermore, given the performance of such methods in the prediction of flow features in turbulent boundary layers, they have a good potential for implementation in experiments and realistic flow control applications, where the prediction of the near-wall peak led to correlations above 0.80 when wall-shear stress was used in a multiple-input or nonlinear scheme. Errors of the order of 20 % were also observed in the determination of the near-wall spectral peak, depending on the employed method.

In this work, we investigate numerically the perturbative effects of cell aperture in heat transport and thermal dissipation rate for a vertical Hele-Shaw geometry, which is used as an analogue representation of a planar vertical fracture at the laboratory scale. To model the problem, we derive a two-dimensional set of equations valid for this geometry. For Hele-Shaw cells heated from below and above, with periodic boundary conditions in the horizontal direction, the model gives new nonlinear scalings for both the time-averaged Nusselt number $\langle Nu\rangle _{\unicode[STIX]{x1D70F}}$ and dimensionless mean thermal dissipation rate $\langle \unicode[STIX]{x1D717}\rangle _{\unicode[STIX]{x1D70F}}$ in the high-Rayleigh regime. We demonstrate that $\langle Nu\rangle _{\unicode[STIX]{x1D70F}}$ and $\langle \unicode[STIX]{x1D717}\rangle _{\unicode[STIX]{x1D70F}}$ depend upon the cell anisotropy ratio $\unicode[STIX]{x1D716}$, which measures the ratio between the cell gap and height. We show that $\langle Nu\rangle _{\unicode[STIX]{x1D70F}}$ values in the high-Rayleigh regime decrease when $\unicode[STIX]{x1D716}$ grows, supporting the field observations at the fracture scale. When $\unicode[STIX]{x1D716}\ll 1$, our results are in agreement with the scalings found using the Darcy model. The numerical results satisfy the theoretical relation $\langle Nu\rangle _{\unicode[STIX]{x1D70F}}=Ra\langle \unicode[STIX]{x1D717}\rangle _{\unicode[STIX]{x1D70F}}$, which is obtained from the model. This latter relation is valid for all values of Rayleigh number considered. The perturbative effects of cell aperture are observed only in the exponents of the scalings $\langle Nu\rangle _{\unicode[STIX]{x1D70F}}\sim Ra^{\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D716})}$ and $\langle \unicode[STIX]{x1D717}\rangle _{\unicode[STIX]{x1D70F}}\sim Ra^{\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D716})-1}$.

In the microcirculation, a plasma layer forms near the vessel walls that is free of red blood cells (RBCs). This region, often termed as the cell-free layer (CFL), plays important haemorheological and biophysical roles, and has been the subject of extensive research. Many previous studies have considered the CFL development in single, isolated vessels that are straight tubes or channels, as well as in isolated bifurcations and mergers. In the body, blood vessels are typically winding and sequentially bifurcate into smaller vessels or merge to form larger vessels. Because of this geometric complexity, the CFL in vivo is three-dimensional (3D) and asymmetric, unlike in fully developed flow in straight tubes. The three-dimensionality of the CFL as it develops in a vascular network, and the underlying hydrodynamic mechanisms, are not well understood. Using a high-fidelity model of cellular-scale blood flow in microvascular networks with in vivo-like topologies, we present a detailed analysis of the fully 3D and asymmetric nature of the CFL in such networks. We show that the CFL significantly varies over different aspects of the networks. Along the vessel lengths, such variations are predominantly non-monotonic, which indicates that the CFL profiles do not simply become more symmetric over the length as they would in straight vessels. We show that vessel tortuosity causes the CFL to become more asymmetric along the length. We specifically identify a curvature-induced migration of the RBCs as the underlying mechanism of increased asymmetry in curved vessels. The vascular bifurcations and mergers are also seen to change the CFL profiles, and in the majority of them the CFL becomes more asymmetric. For most bifurcations, this is generally observed to occur such that the CFL downstream narrows on the side of the vessel nearest the upstream bifurcation, and widens on the other side. The 3D aspects of such behaviour are elucidated. For many bifurcations, a discrepancy exists between the CFL in the daughter vessels, which arises from a disproportionate partitioning between the flow rate and RBC flux. For most mergers, the downstream CFL narrows in the plane of the merger, but widens away from this plane. The dominant mechanism by which such changes occur is identified as the geometric focusing of the two merging streams. To our knowledge, this work provides the first simulation-based analysis of the 3D CFL structure in complex in vivo-like microvascular networks, including the hydrodynamic origins of the observed behaviour.

From shaping cosmic structures in space to producing intense sounds in aircraft engines, shock waves in fluids ineluctably convert entropy fluctuations into swirling motions and sound waves. Studies of the corresponding conversion from internal energy to kinetic energy have so far been restricted to ideal (or idealised) fluids. Yet, many substances do not obey the ideal-gas law (including those in the above two examples). The present work demonstrates that non-ideal thermodynamic properties provide a remarkable degree of control over the conversion to solenoidal and dilatational kinetic energies. Of particular interest is the ability to suppress much of the emitted acoustic field whilst promoting mixing downstream of the shock. This is made possible by exploiting the convexity (or lack thereof) of the shock adiabats. Whilst illustrated here using dense vapours near the thermodynamic critical point, this ability to design and control specific shock-induced energy transfers extends beyond near-critical-point phenomena; e.g. shocked mixtures (high-speed dusty flows on Mars, nanoparticle formation in supersonic expanders for drug manufacturing), reacting fronts (supersonic combustion, rocket propulsion), ionising shocks (reentry systems, inertial confinement fusion) or fronts in active fluids (bacterial and crowd flows). This theoretical work, which demonstrates the predictive capabilities of linear theory, lays the foundation for future experimental investigations ultimately aimed at delivering novel shock-based flow-control strategies exploiting the thermodynamic properties of the fluid.

The asymmetrical Mach reflection configuration is studied analytically in this paper, using an asymmetrical model extended from a recent symmetrical model and accounting for the new features related to asymmetry of the two wedges. It is found that the two sliplines do not turn parallel to the incoming flow at the same horizontal location and the sonic throat locates at the position where the difference of slopes of the two sliplines vanishes. This allows us to define a new sonic throat compatibility condition essential to determine the size of the Mach stem. The present model gives the height of the Mach stem, declined angle of the Mach stem from vertical axis, sonic throat location and shape of all shock waves and sliplines. The accuracy of the model is checked by computational fluid dynamics (CFD) simulation. It is found that the Mach stem height is strongly dependent on asymmetry of the wedge angles and almost linearly dependent on the asymmetry of the wedge lower surface lengths. The Mach stem height is shown to be insensitive to the asymmetry of the horizontal positions of the two wedges. The mechanisms for these observations are explained. For instance, it is demonstrated that the Mach reflection configuration remains closely similar when there is horizontal shift of either wedge.

Water wave attenuation by grease ice is a key mechanism for the polar regions, as waves in ice influence many phenomena such as ice drift, ice breaking and ice formation. However, the models presented so far in the literature are limited in a number of regards, and more insights are required from either laboratory experiments or fieldwork for these models to be validated and improved. Unfortunately, performing detailed measurements of wave propagation in grease ice, either in the field or in the laboratory, is challenging. As a consequence, laboratory data are relatively scarce, and often consist of only a couple of wave elevation measurements along the length of the wave tank. We present combined measurements of wave elevation using an array of ultrasonic probes, and water kinematics using particle image velocimetry (PIV), in a small-scale wave tank experiment. Experiments are performed over a wider frequency range than has been previously investigated. The wave elevation measurements are used to compute the wavenumber and exponential damping coefficient. In contrast to a previous study in grease ice, we find that the wavenumber is consistent with the mass loading model, i.e. it increases compared with the open water case. Wave attenuation is compared with a series of one-layer models, and we show that they satisfactorily describe the viscous damping occurring. PIV data are also consistent with exponential wave amplitude attenuation, and a proper orthogonal decomposition analysis reveals the existence of mean flows under the ice that are a consequence of the displacement and packing of the ice induced by the gradient in the wave-induced stress. Finally, we show that the dynamics of grease ice can generate eddy structures that inject eddy viscosity into the water under the grease ice, which would lead to enhanced mixing and participating in energy dissipation.

We develop a model for predicting the flow resulting from the relaxation of pre-strained, fluid-filled, elastic network structures. This model may be useful for understanding relaxation processes in various systems, e.g. deformable microfluidic systems or by-products from hydraulic fracturing operations. The analysis is aimed at elucidating features that may provide insight on the rate of fluid drainage from fracturing operations. The model structure is a bifurcating network made of fractures with uniform length and elastic modulus, which allows for general self-similar branching and variation in fracture length and rigidity between fractures along the flow path. A late-time $t^{-1/3}$ power law is attained and the physical behaviour can be classified into four distinct regimes that describe the late-time dynamics based on the location of the bulk of the fluid volume (which shifts away from the outlet as branching is increased) and pressure drop (which shifts away from the outlet as rigidity is increased upstream) along the network. We develop asymptotic solutions for each of the regimes, predicting the late-time flux and evolution of the pressure distribution. The effects of the various parameters on the outlet flux and the network’s drainage efficiency are investigated and show that added branching and a decrease in rigidity upstream tend to increase drainage time.

We study experimentally the spatial distribution, settling and interaction of sub-Kolmogorov inertial particles with homogeneous turbulence. Utilizing a zero-mean-flow air turbulence chamber, we drop size-selected solid particles and study their dynamics with particle imaging and tracking velocimetry at multiple resolutions. The carrier flow is simultaneously measured by particle image velocimetry of suspended tracers, allowing the characterization of the interplay between both the dispersed and continuous phases. The turbulence Reynolds number based on the Taylor microscale ranges from $Re_{\unicode[STIX]{x1D706}}\approx 200{-}500$, while the particle Stokes number based on the Kolmogorov scale varies between $St_{\unicode[STIX]{x1D702}}=O(1)$ and $O(10)$. Clustering is confirmed to be most intense for $St_{\unicode[STIX]{x1D702}}\approx 1$, but it extends over larger scales for heavier particles. Individual clusters form a hierarchy of self-similar, fractal-like objects, preferentially aligned with gravity and with sizes that can reach the integral scale of the turbulence. Remarkably, the settling velocity of $St_{\unicode[STIX]{x1D702}}\approx 1$ particles can be several times larger than the still-air terminal velocity, and the clusters can fall even faster. This is caused by downward fluid fluctuations preferentially sweeping the particles, and we propose that this mechanism is influenced by both large and small scales of the turbulence. The particle–fluid slip velocities show large variance, and both the instantaneous particle Reynolds number and drag coefficient can greatly differ from their nominal values. Finally, for sufficient loadings, the particles generally augment the small-scale fluid velocity fluctuations, which however may account for a limited fraction of the turbulent kinetic energy.

We present analytical examples of fluid dynamos that saturate through the action of the Coriolis and inertial terms of the Navier–Stokes equation. The flow is driven by a body force and is subject to global rotation and uniform sweeping velocity. The model can be studied down to arbitrarily low viscosity and naturally leads to the strong-field scaling regime for the magnetic energy produced above threshold: the magnetic energy is proportional to the global rotation rate and independent of the viscosity $\unicode[STIX]{x1D708}$. Depending on the relative orientations of global rotation and large-scale sweeping, the dynamo bifurcation is either supercritical or subcritical. In the supercritical case, the magnetic energy follows the scaling law for supercritical strong-field dynamos predicted on dimensional grounds by Pétrélis & Fauve (Eur. Phys. J. B, vol. 22, 2001, pp. 271–276). In the subcritical case, the system jumps to a finite-amplitude dynamo branch. The magnetic energy obeys a magneto-geostrophic scaling law (Roberts & Soward, Annu. Rev. Fluid Mech., vol. 4, 1972, pp. 117–154), with a turbulent Elsasser number of the order of unity, where the magnetic diffusivity of the standard Elsasser number appears to be replaced by an eddy diffusivity. In the absence of global rotation, the dynamo bifurcation is subcritical and the saturated magnetic energy obeys the equipartition scaling regime. We consider both the vicinity of the dynamo threshold and the limit of large distance from threshold to put these various scaling behaviours on firm analytical ground.

Thermal transport in rarefied flows far removed from thermodynamic equilibrium is investigated using kinetic-theory-based numerical simulations. Two numerical schemes – unified gas kinetic scheme (UGKS) and direct simulation Monte Carlo (DSMC) – are employed to simulate transport at different degrees of rarefaction. Lid-driven cavity flow simulations of argon gas are performed over a range of Knudsen numbers, Mach numbers and cavity shapes. Thermal transport is then characterized as a function of lid Mach number and Knudsen number for different cavity shapes. Vast deviations from the Fourier law – including thermal transport aligned along the direction of temperature gradient – are observed. Entropy implications are examined using Sackur–Tetrode and Boltzmann $H$-theorem formulations. At low Knudsen and Mach numbers, thermal transport is shown to be amenable to both entropy formulations. However, beyond moderate Knudsen and Mach numbers, thermal transport complies only with the Boltzmann $H$-theorem entropy statement. Two extended thermodynamic models are compared against simulation data and found to account for some of the observed non-equilibrium behaviour.

We consider the instability properties of dense granular flow in inclined plane and plane shear geometries as tests for the compressible inertial-dependent rheology. The model, which is a recent generalisation of the incompressible $\unicode[STIX]{x1D707}(I)$ rheology, constitutes a hydrodynamical description of dense granular flow which allows for variability in the solids volume fraction. We perform a full linear stability analysis of the model and compare its predictions to existing experimental data for glass beads on an inclined plane and discrete element simulations of plane shear in the absence of gravity. In the case of the former, we demonstrate that the compressible model can quantitatively predict the instability properties observed experimentally, and, in particular, we find that it performs better than its incompressible counterpart. For the latter, the qualitative behaviour of the plane shear instability is also well captured by the compressible model.

The high-speed impingement of hollow droplets embedded with a cavity has fundamental applications in various scenarios, such as in spray coating and biomedical engineering. The impingement dynamics is modulated by the wrapping medium, different from that of denser solid droplets. With air and vapour cavities, the impingement of two kinds of hollow cylindrical droplets is simulated in the present study to investigate the morphology and physical mechanisms regarding droplet and cavity dynamics. The compressible two-phase Eulerian model is used to couple with the phase transition procedure. The results detail the evolution of droplets and collapsing dynamics of the two kinds of cavities. Processes are captured in which the impinging water-hammer shock wave interacts with the cavity, and vertical liquid jets are induced to impact the embedded cavity. For the case of the air cavity, a transmitted shock wave is formed and propagates inside the cavity. The air cavities are compressively deformed and broken into a series of small cavities. Subsequently, a range of intermittent collapsing compression wavelets are generated due to the interface collapse driven by local jets. As for the vapour cavity in the saturated state, initially, once it is impacted by the impinging shock wave, it gradually shrinks accompanied by local condensation but without generation of transmitted waves. Following the first interaction between the lower and upper surfaces of the cavity, the vapour cavity undergoes continuous condensation and collapse with repeated interface fusion. The vapour cavity finally turns into liquid water blended into the surroundings, and the strong collapsing shock waves are expanded inside the droplet. The radius ratios and initial impinging speeds are chosen to analyse the variation of the collapsing time, maximum collapsing pressure and mean pressure on the rigid wall. The pressure withstood by the wall due to the collapsing cavity increases with the initial size of the cavity and initial impinging speed. The maximum local pressures in the entire fluids and the mean pressure on the wall during the collapsing of the vapour cavities are higher than those for the air cavities.

We investigate three-dimensional flagellar swimming in a fluid with a sparse network of stationary obstacles or fibres. The Brinkman equation is used to model the average fluid flow where a flow-dependent term, including a resistance parameter that is inversely proportional to the permeability, captures the effects of the fibres on the fluid. To solve for the local linear and angular velocities that are coupled to the flagellar motion, we extend the method of regularized Brinkmanlets to incorporate a Kirchhoff rod, discretized as point forces and torques along a centreline. Representing a flagellum as a Kirchhoff rod, we investigate emergent waveforms for different preferred strain and twist functions. Since the Kirchhoff rod formulation allows for out-of-plane motion, in addition to studying a preferred planar sine wave configuration, we also study a preferred helical configuration. Our numerical method is validated by comparing results to asymptotic swimming speeds derived for an infinite-length cylinder propagating planar or helical waves. Similar to the asymptotic analysis for both planar and helical bending, we observe that with small amplitude bending, swimming speed is always enhanced relative to the case with no fibres in the fluid (Stokes) as the resistance parameter is increased. For regimes not accounted for with asymptotic analysis, i.e. large amplitude planar and helical bending, our model results show a non-monotonic change in swimming speed with respect to the resistance parameter; a maximum swimming speed is observed when the resistance parameter is near one. The non-monotonic behaviour is due to the emergent waveforms; as the resistance parameter increases, the swimmer becomes incapable of achieving the amplitude of its preferred configuration. We also show how simulation results of slower swimming speeds for larger resistance parameters are actually consistent with the asymptotic swimming speeds if work in the system is fixed.