Hydrodynamic signatures at the Stokes regime, pertinent to motility of micro-swimmers, have a long-range nature. This implies that movements of an object in such a viscosity-dominated regime can be felt tens of body-lengths away and significantly alter dynamics of the surrounding environment. Here, we devise a systematic methodology to actively cloak swimming objects within any arbitrarily crowded suspension of micro-swimmers. Specifically, our approach is to conceal the target swimmer throughout its motion using cooperative flocks of swimming agents equipped with adaptive decision-making intelligence. Through a reinforcement learning algorithm, the cloaking agents experientially learn an optimal adaptive behaviour policy in the presence of flow-mediated interactions. This artificial intelligence enables them to dynamically adjust their swimming actions, so as to optimally form and robustly retain any desired arrangement around the moving object without disturbing it from its original path. Therefore, the presented active cloaking approach not only is robust against disturbances, but also is non-invasive to motion of the cloaked object. We then further generalize the proposed approach and demonstrate how our cloaking agents can be readily used, in any region of interest, to realize hydrodynamic invisibility cloaks around any number of arbitrary intruders.

A rigorous derivation and validation for linear fluid–structure-interaction (FSI) equations for a rigid-body motion problem is performed in an Eulerian framework. We show that the ‘added stiffness’ terms arising in the formulation of Fanion et al. (Revue Européenne des Éléments Finis, vol. 9, issue 6–7, 2000, pp. 681–708) vanish at the FSI interface in a first-order approximation and can be neglected when considering the growth of infinitesimal disturbances. Several numerical tests with rigid-body motion are performed to show the validity of the derived formulation by comparing the time evolution between the linear and nonlinear equations when the base flow is perturbed by identical small-amplitude perturbations. In all cases both the growth rate and angular frequency of the instability matches within $0.1\,\%$ accuracy. The derived formulation is used to investigate the phenomenon of symmetry breaking for a rotating cylinder with an attached splitter plate. The results show that the onset of symmetry breaking can be explained by the existence of a zero frequency linearly unstable mode of the coupled FSI system. Finally, the structural sensitivity of the least stable eigenvalue is studied for an oscillating cylinder, which is found to change significantly when the fluid and structural frequencies are close to resonance.

A number of recent results have shown that within the shallow water, rigid-lid approximation, alongshore variations in the bathymetry, i.e. a submerged ridge, can lead to continental shelf waves (CSWs) that are localised geographically at the ridge and then decay both along and away from the coast. Removing the rigid-lid assumption, introduces the superinertial Poincaré waves and a Kelvin wave that is present at all frequencies. This implies that the spectrum of the associated wave operator, which is bounded for the rigid lid case, is continuous and unbounded for the free-surface case. In the rigid-lid case the localisation of modes are isolated eigenvalues lying above the continuous spectrum whereas any localised modes for the free-surface problem must necessarily be embedded in the continuous spectrum. The purpose of this work is to construct trapped CSWs analytically and numerically for a non-rectilinear shelf. A regular asymptotic method is employed by considering a slowly varying, non-rectilinear shelf with an approximate boundary condition at the shelf–ocean boundary. It is shown that even with the free-surface present, trapped CSWs do indeed exist for the submerged ridge topography. Comparison with highly accurate numerical results demonstrates the accuracy of the asymptotic method and also allows the consideration of shelves that abut an open ocean so avoiding the approximate boundary condition at the shelf–ocean boundary.

In this study, we connect concepts that have been recently developed in thermoacoustics, specifically (i) high-order spectral perturbation theory, (ii) symmetry-induced degenerate thermoacoustic modes, (iii) intrinsic thermoacoustic modes and (iv) exceptional points. Their connection helps gain physical insight into the behaviour of the thermoacoustic spectrum when parameters of the system are varied. First, we extend high-order adjoint-based perturbation theory of thermoacoustic modes to the degenerate case. We provide explicit formulae for the calculation of the eigenvalue corrections to any order. These formulae are valid for self-adjoint, non-self-adjoint or even non-normal systems; therefore, they can be applied to a large range of problems, including fluid dynamics. Second, by analysing the expansion coefficients of the eigenvalue corrections as a function of a parameter of interest, we accurately estimate the radius of convergence of the power series. Third, we connect the existence of a finite radius of convergence to the existence of singularities in parameter space. We identify these singularities as exceptional points, which correspond to defective thermoacoustic eigenvalues, with infinite sensitivity to infinitesimal changes in the parameters. At an exceptional point, two eigenvalues and their associated eigenvectors coalesce. Close to an exceptional point, strong veering of the eigenvalue trajectories is observed. As demonstrated in recent work, exceptional points naturally arise in thermoacoustic systems due to the interaction between modes of acoustic and intrinsic origin. The role of exceptional points in thermoacoustic systems sheds new light on the physics and sensitivity of thermoacoustic stability, which can be leveraged for passive control by small design modifications.

A fibre withdrawn from a bath of a dilute particulate suspension exhibits different coating regimes depending on the physical properties of the fluid, the withdrawal speed, the particle sizes and the radius of the fibre. Our experiments indicate that only the liquid without particles is entrained for thin coating films. Beyond a threshold capillary number, the fibre is coated by a liquid film with entrained particles. We systematically characterize the role of the capillary number, the particle size and the fibre radius on the threshold speed for particle entrainment. We discuss the boundary between these two regimes and show that the thickness of the liquid film at the stagnation point controls the entrainment process. The radius of the fibre provides a new degree of control in capillary filtering, allowing greater control over the size of the particles entrained in the film.

We numerically simulate three-dimensional Rayleigh–Bénard convection, the flow in a fluid layer heated from below and cooled from above, with inhomogeneous temperature boundary conditions, to explore two distinct regimes described in recent literature. We fix the non-dimensional temperature difference, i.e. the Rayleigh number, to $Ra=10^8$, and vary the Prandtl number between $1$ and 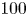$100$. By introducing stripes of adiabatic boundary conditions on the top plate, and making the surface of the top plate only $50\,\%$ conducting, we modify the heat transfer, the average temperature profiles and the underlying flow properties. We find two regimes: when the pattern wavelength is small, the flow is barely affected by the stripes. The heat transfer is reduced, but remains a large fraction of that of the unmodified case, and the underlying flow is only slightly modified. When the pattern wavelength is large, the heat transfer saturates to approximately two-thirds of the value of the unmodified problem, the temperature in the bulk increases substantially, and velocity fluctuations in the directions normal to the stripes are enhanced. The transition between the two regimes happens at pattern wavelength around the distance between the two plates, with different quantities transitioning at slightly different wavelength values. This transition is approximately Prandtl-number-independent, even if the statistics in the long-wavelength regime slightly vary.

In a three-layer system, weakly nonlinear theory predicts that breathers exist under certain conditions which, under the Boussinesq approximation, include symmetric stratifications in which the density jump across each interface is the same and the upper and lower layer thicknesses are equal and less than 9/26 of the total water depth. The existence and characteristics of fully nonlinear breathers in this symmetric stratification are poorly understood. Therefore, this study investigates fully nonlinear breathers in a three-layer symmetric stratification in order to clarify their characteristics by making direct comparisons between numerical simulation results and theoretical solutions. A normalization of the breather profiles is introduced using theoretical solutions of a breather and a new energy scale is proposed to evaluate their potential and kinetic energy. We apply fully nonlinear and strongly dispersive internal wave equations in a three-layer system using a variational principle. The computational results show that the larger the amplitude, the shorter the length of the envelope of breathers, which agrees with the theoretical solution. However, breathers based on the theoretical solutions cannot progress without deformation and decay due to the emission of short small-amplitude internal waves. Furthermore we demonstrate that the shedding of larger amplitude waves occurs, and the amplitude of the envelope decays more strongly when the density interface crosses the critical depth where the ratio of the upper layer thickness and the total water depth is 9/26 suggesting a limiting amplitude for fully nonlinear breathers.

We investigate the effects of magnetisation on the two-fluid plasma Richtmyer–Meshkov instability of a single-mode thermal interface using a computational approach. The initial magnetic field is normal to the mean interface location. Results are presented for a magnetic interaction parameter of 0.1 and plasma skin depths ranging from 0.1 to 10 perturbation wavelengths. These are compared to initially unmagnetised and neutral fluid cases. The electron flow is found to be constrained to lie along the magnetic field lines resulting in significant longitudinal flow features that interact strongly with the ion fluid. The presence of an initial magnetic field is shown to suppress the growth of the initial interface perturbation with effectiveness determined by plasma length scale. Suppression of the instability is attributed to the magnetic field's contribution to the Lorentz force. This acts to rotate the vorticity vector in each fluid about the local magnetic-field vector leading to cyclic inversion and transport of the out-of-plane vorticity that drives perturbation growth. The transport of vorticity along field lines increases with decreasing plasma length scales and the wave packets responsible for vorticity transport begin to coalesce. In general, the two-fluid plasma Richtmyer–Meshkov instability is found to be suppressed through the action of the imposed magnetic field with increasing effectiveness as plasma length scale is decreased. For the conditions investigated, a critical skin depth for instability suppression is estimated.

An experimental investigation using stereo particle image velocimetry (SPIV) was performed to study the vortex dynamics of an aspect ratio 13 rectangular orifice synthetic jet issuing into quiescent fluid. Data were obtained on the orifice centreline planes for four Reynolds numbers and five Strouhal numbers, ranging from 298 to 731 and 0.039 to 0.100, respectively. At one condition, SPIV data obtained on parallel planes distributed across the width of the jet were used to reconstruct the three-dimensional, three-component velocity field. The results reveal that the axis switching deformations of the non-circular vortex rings give rise to several types of secondary structures. Before the primary vortex ring completed its first axis switch, the anti-parallel sides of the ring collided, resulting in vorticity reconnection. Consequently, the elongated vortex ring bifurcated into two circular vortex rings which propagated off along independent paths. The development and structure of vortex ring bifurcation in addition to the bifurcation's effect on the jet shape, momentum and entrainment are presented. The bifurcation process captured in the synthetic jet experiment is similar in many ways to computational simulations of isolated vortex rings. Despite these similarities and the fact that vortex ring bifurcation was detected for all conditions tested, it is shown that the bifurcation process is sensitive to the unique conditions in synthetic jets, specifically the proximity of other vortex rings as indicated by the Strouhal number. Finally, the results at different jet conditions are discussed in relation to prior studies of synthetic jets.

We show a method, for direct numerical simulations, to trigger and maintain turbulent bands directly at low Reynolds numbers in channel flow. The key is to impose a moving localised force which induces a local flow with sufficiently strong inflectional instability. With the method, we can trigger and maintain turbulent bands at Reynolds numbers down to $Re\simeq 500$. More importantly, we can generate any band patterns with desired relative position and orientation. The usual perturbation approach resorts to turbulent fields simulated at higher Reynolds numbers, random noise or localised vortical perturbation, which neither assures a successful generation of bands at low Reynolds numbers nor offers a control on the orientation of the generated bands. A precise control on the position and orientation of turbulent bands is important for the investigation of all possible types of band interaction at low Reynolds numbers and for understanding the subcritical transition in channel flow.

Mach reflection subjected to the influence of an upstream shock wave from the same side is studied here. This situation occurs when two incident shock waves induced by a double wedge reflect at the same point of the reflecting surface and when the downstream incident shock wave is stronger than the upstream one. A shock polar analysis is used to show that this configuration produces an inverted Mach stem and a type IV shock interference between the Mach stem and the upstream shock wave. This shock interference produces a jet that divides the flow stream downstream of the Mach stem into two ducts with different sonic throats, thus complicating the mechanism by which the Mach stem size is determined. A transition analysis shows that the Mach reflection of the downstream shock wave is promoted by the upstream one. Computational fluid dynamics is used to assess the flow pattern anticipated by shock polar analysis and demonstrates how the heights of Mach stem and jet depend on the inflow Mach number and wedge turning angle.

We report on the experimental investigation of the large-scale instantaneous flow structures in turbulent Taylor–Couette flow using tomographic particle image velocimetry. The results indicate three distinct regimes for counter-rotating flow within a shear Reynolds number range of $11\,000 < Re_S < 47\,000$. Close to only inner cylinder rotation, large-scale structures are aligned in the azimuthal direction, similar to Taylor vortices. Near the point of only outer cylinder rotation, we observe columnar vortical structures in the axial direction, which are associated with small Rossby numbers. This is the first time such columnar structures are reported in a fully turbulent Taylor–Couette flow. A transition between these two regimes is observed around the point of exact counter-rotation, where the instantaneous azimuthal structures are inclined with respect to the walls. Furthermore, it is shown that the reported transitions in the turbulent flow structure modify the angular momentum transport, thereby affecting the torque scaling.

We apply conformal mapping to find the evolving shapes of a dissolving cylinder in a potential flow. Similar equations can be used to describe melting in a flowing liquid phase. Results are compared with microfluidic experiments and numerical simulations. Shapes predicted by conformal mapping agree almost perfectly with experimental observations, after a modest (20 %) rescaling of the time. Finite-volume simulations show that the differences with experiment are connected to the underlying assumptions of the analytical model: potential flow and diffusion-limited dissolution. Approximate solutions of the equations describing the evolution of the shape of the undissolved solid can be derived from a Laurent expansion of the mapping function from the unit circle. Asymptotic expressions for the evolution of the area of the disk and the shift in its centre of mass have been derived at low and high Péclet number. Analytic approximations to the leading-order Laurent coefficients provide additional insight into the mechanisms underlying pore-scale dissolution.

We measure the Kelvin–Helmholtz instability in between a layer of a diamagnetic fluid flowing in a channel and a layer of ferrofluid resting on top. When the diamagnetic fluid exceeds a critical flow velocity the interface in between both fluids becomes unstable and waves develop. It has been predicted by Sutyrin & Taktarov (J. Appl. Math. Mech., vol. 39, 1975, pp. 520–524) that a homogeneous magnetic field, oriented horizontally, stabilizes the liquid interface. To test this prediction we apply in a closed flow channel a local periodic perturbation of the interface by magnetic or mechanic means. From the measured growth and decay rates of the interface undulations we determine the critical flow velocity for various driving frequencies and applied magnetic fields. In this way we confirm quantitatively the stabilizing effect of the horizontal field. Moreover we measure the dispersion relation of the interfacial waves.

This article examines numerically the two-dimensional fluid–structure interaction problem of a circular cylinder rolling under gravity along an inclined surface under the assumption of a fixed but small gap. The motion of the cylinder is governed by the ratio of cylinder and fluid densities and the Reynolds number based on a velocity scale derived from the momentum balance in the asymptotic regime. For increasing Reynolds number, the cylinder wake undergoes a transition from steady to periodic flow, causing oscillations of the cylinder motion. The critical Reynolds number increases for light cylinders. Whereas the time-averaged characteristics of the asymptotic rolling states depend only on the Reynolds number, the density ratio has an additional influence on the vibration amplitude and on the cylinder motion during a start-up transient from rest. Light cylinders reach their final state quickly after the initial acceleration; heavier cylinders traverse a series of quasi-steady states, including a temporary velocity overshoot, before settling in the asymptotic regime. The amplitudes of the flow-induced vibrations remain small over the entire parameter range, which can be attributed to the value of the added-mass force associated with a rolling cylinder. Special attention is paid to the influence of the small but finite gap between cylinder and wall, since lubrication theory predicts a diverging pressure drag for a vanishing gap. The variations with gap size of the forces, torque and added mass are explored. The gap also influences the characteristics of the cylinder vibrations in the unsteady wake regime, in particular their amplitude.

We present the results of a combined experimental and theoretical investigation of the stability of rings of millimetric droplets bouncing on the surface of a vibrating liquid bath. As the bath's vibrational acceleration is increased progressively, droplet rings are found to destabilize into a rich variety of dynamical states including steady rotational motion, periodic radial or azimuthal oscillations and azimuthal travelling waves. The instability observed is dependent on the ring's initial radius and drop number, and whether the drops are bouncing in- or out-of-phase relative to their neighbours. As the vibrational acceleration is further increased, more exotic dynamics emerges, including quasi-periodic motion and rearrangement into regular polygonal structures. Linear stability analysis and simulation of the rings based on the theoretical model of Couchman et al. (J. Fluid Mech., vol. 871, 2019, pp. 212–243) largely reproduce the observed behaviour. We demonstrate that the wave amplitude beneath each drop has a significant influence on the stability of the multi-droplet structures: the system seeks to minimize the mean wave amplitude beneath the drops at impact. Our work provides insight into the complex interactions and collective motions that arise in bouncing-droplet aggregates.

In this paper, a semi-analytical model based on linear potential flow theory and an eigenfunction expansion method is developed to study wave scattering by an array of structured cylinders in water of finite depth. Each cylinder is formed by a closely spaced array of thin vertical plates, between which fluid can flow, extending through the depth. In order to consider the wave attenuation and energy dissipation in narrow gaps between the thin vertical plates, a damping mechanism is introduced at the surface of the fluid occupied by the structured cylinders. In addition to a direct calculation of the energy dissipation, an indirect method based on Kochin functions is derived with the employment of energy identities. The present model is shown to be in excellent agreement with both the published data and those obtained by using different methods. The validated model is then applied to study the effect of a pair of structured cylinders on wave focusing/blocking, scattered far-field amplitude and wave power dissipation. Results show that wave focusing/blocking can be achieved by the appropriate choice of plate alignment. The structured cylinders hold profound potential for wave power extraction.

Stability features of two-stream coaxial plasma jet simulations are investigated using numerical solutions to the spatio-temporal one-dimensional linear stability theory problem. The base states obtained from magneto-hydrodynamic simulations consider the flow as a mixture of gases in local thermodynamic equilibrium (LTE) while stability computations are performed assuming both a calorically perfect gas (CPG) model and LTE. Comparisons with solutions considering a simple CPG model show the non-negligible impact of the LTE on the stability attributes of the plasma jet. For all cases studied, a large region of absolute instability is found for the axisymmetric mode, starting at the jet inlet. The streamwise evolution of the absolute growth rate is found to depend both on the baroclinic torque and the displacement of the maximum shear toward low velocity regions of the jet, combining effects described in the literature. The jet is controlled by means of electric power and static pressure at constant mass flow. The former affects mainly the absolute growth rate through changes of the core-to-bypass stream velocity ratio, while the latter influences mostly the absolute frequency. Finally, the full impulse response reveals a competition mechanism between the absolute mixed modes dominating at low group velocities, and convective shear layer modes at higher group velocities, restricted to the first half of the chamber.

We report a series of experiments in which a cylinder, with a vertical axis, is moved back and forth along a long narrow channel containing fresh water at Reynolds numbers $Re=3220\text {--}13\,102$. We examine the mixing of a cloud of dye along the channel by the oscillatory motion of the cylinder. Using light attenuation techniques to measure the time evolution of the concentration of dye along the channel, we find that at early times the concentration profile collapses to a Gaussian profile with dispersivity, $D=(2.4\pm 0.5) fdW$, where $f$ is the frequency of the cylinder oscillation, $d$ is the diameter of the cylinder and $W$ is the width of the channel, respectively. For times much longer than $L^2/D$, with $L$ being the length of the channel, the concentration becomes progressively more uniform over the whole length of the channel, and we show that the long-time non-uniform component decays with time dependence $\exp (- 4{\rm \pi} ^2 Dt / L^2)$. We consider the implications of these experiments for the dispersal of viral aerosols along poorly ventilated corridors, with implications for infection transmission in hospitals and public buildings.