We study the Marangoni propulsion of a spheroidal particle located at a liquid–gas interface. The particle asymmetrically releases an insoluble surface-active agent and so creates and maintains a surface tension gradient leading to the self-propulsion. Assuming that the surface tension has a linear dependence on the concentration of the released agent, we derive closed-form expressions for the translational speed of the particle in the limit of small capillary, Péclet and Reynolds numbers. Our derivations are based on the Lorentz reciprocal theorem, which eliminates the need to develop the detailed flow field.

Experiments on the non-Boussinesq gravity currents generated from an instantaneous buoyancy source propagating on an inclined boundary in the slope angle range 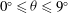$0^{\circ } \le \theta \le 9^{\circ }$ with relative density difference in the range of 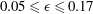$0.05 \le \epsilon \le 0.17$ are reported, where 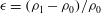$\epsilon = (\rho _1-\rho _0)/\rho _0$, with 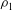$\rho _1$ and 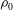$\rho _0$ the densities of the heavy and light ambient fluids, respectively. We showed that a 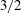$3/2$ power-law, 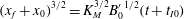${(x_f+x_0)}^{3/2}= K_M^{3/2} {B_0'}^{1/2} (t+t_{I0})$, exists between the front location measured from the virtual origin, $(x_f+x_0)$, and time, $t$, in the early deceleration phase for both the Boussinesq and non-Boussinesq cases, where 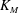$K_M$ is a measured empirical constant, 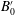$B_0'$ is the total released buoyancy, and 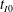$t_{I0}$ is the $t$-intercept. Our results show that 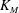$K_M$ not only increases as the relative density difference increases but also assumes its maximum value at 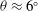$\theta \approx 6^{\circ }$ for sufficiently large relative density differences. In the late deceleration phase, the front location data deviate from the $3/2$ power-law and the flow patterns on $\theta =6^{\circ },9^{\circ }$ slopes are qualitatively different from those on 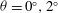$\theta =0^{\circ },2^{\circ }$. In the late deceleration phase, we showed that viscous effects could become more important and another power-law, 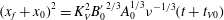${(x_f+x_0)}^{2}= K_{V}^{2} {B_0'}^{2/3} {{A}^{1/3}_0} {\nu }^{-1/3} (t+t_{V0})$, applies for both the Boussinesq and non-Boussinesq cases, where 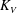$K_V$ is an empirical constant, 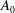$A_0$ is the initial volume of heavy fluid per unit width, $\nu $ is the kinematic viscosity of the fluids, and $t_{V0}$ is the $t$-intercept. Our results also show that 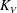$K_V$ increases as the relative density difference increases and 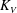$K_V$ assumes its maximum value at 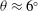$\theta \approx 6^{\circ }$.

The stability of a suspension of chemotactic bacteria confined in an infinitely long channel and subjected to a stationary, linear chemo-attractant gradient is investigated. While swimming, individual bacteria exert force dipoles on the fluid, which at the continuum level lead to a stress depending upon the bacterial orientation and number density fields. The presence of the attractant gradient causes bacteria to tumble less frequently when swimming along the gradient, leading to a mean orientation and a non-zero chemotactic drift velocity $U_0$ in that direction. At long length and time scales compared to those associated with the persistence of bacterial swimming, fluxes due to chemotaxis and the random run–tumble motion of bacteria balance to yield an exponentially varying number density profile across the channel in the base state. The associated bacterial stress field is also exponentially varying and is normal. This spatially non-uniform base state is unstable to fluctuations in the bacterial concentration field when the scaled bacterial concentration $\beta = (3 C/8) \langle n_0\rangle L^2 H$ exceeds a critical value determined by a Péclet number defined as ${\mathit{Pe}} = U_0 H/\kappa $. Here, $C$ is a non-dimensional dipole strength, which depends on the geometry of the bacterium, $\langle n_0\rangle $ is the bacterial concentration averaged across the channel of depth $H$, $L$ is the total length of the bacterium, $\kappa $ is the bacterial diffusivity, and $\beta _{\mathit{crit}}$ is a monotonically decreasing function of ${\mathit{Pe}}$, with $\beta _{\mathit{crit}} \sim 720/{\mathit{Pe}}^3$ for ${\mathit{Pe}} \ll 1$ and $\beta _{\mathit{crit}} \sim 2$ for ${\mathit{Pe}} \gg 1$. The instability is the result of the coupling between the active stress-driven fluid flow and the bacterial concentration, and manifests as rectangular convection patterns. When $\beta $ first exceeds $\beta _{\mathit{crit}}$, the unstable wavelengths are large with $\lambda \gg H$ and the mode of instability is stationary. Although oscillatory modes appear when $\lambda \leq O(H)$ and $\beta > 247$, the most dangerous mode of instability is found to be always stationary with a wavelength $\lambda _m/H \sim {\mathit{Pe}}^{-1}$. To study the coupling between the previously analysed orientation shear instability mechanism of bacterial suspensions and the new chemotaxis-driven instability, a new set of continuum equations that consistently account for weak chemotaxis, rotation of bacteria by weak fluid shear and weak non-continuum effects along with their coupled effects has been derived. The stability analysis of those equations showed that the orientation shear mechanism has only a negligible influence on the critical concentration for the present chemotaxis-induced instability when the suspension depth is large, and it is the latter that has the lowest critical concentration.

Efforts to reduce the noise from turbulent jets at fixed flow conditions, with aircraft noise as the principal technological motivation, have generally involved some degree of parametric empiricism often based upon a series of trial-and-error testing. As a result, it is unclear if the modest reductions found, in rare cases that do not greatly affect the flow field or incur prohibitive losses, are near the limit of what can be accomplished or if there are undiscovered opportunities for more substantive reductions with better designs or active control. We assess this using an adjoint-based optimization procedure in conjunction with an experimentally validated large-eddy simulation of a Mach 1.3 turbulent jet. The adjoint solution provides a definitive direction in which to adjust a model control actuation in order to reduce noise, providing guidance that seems lacking by any other current means. It is found that three conjugate-gradient iterations in the control space provide ∼3.5 dB of reduction, comparable to other reductions found empirically. The control seems to work by disrupting the coherence of acoustically efficient axisymmetric flow structures. The control and noise-reduction mechanisms are informative, but also suggest that any significantly quieter state would not be a simple perturbation from the uncontrolled jet. Additional iterations might reduce noise more significantly, but there might be only modest opportunities to reduce the sound from simple round turbulent jets without radical changes or relatively sophisticated controls. Though it is difficult to prove any behaviour in a global space of actuations, there does not seem to be a direct route based upon a local sensitivity gradient to substantially quieting a jet, even with an unrealistically flexible actuation. More complex jets or other noisy flows may be more amenable to control, in which case the adjoint-based optimization procedure demonstrated here could provide invaluable engineering guidance.

This paper develops a stability analysis for the onset of thermoacoustic oscillations in a gas-filled looped tube with a stack inserted, subject to a temperature gradient. Analysis is carried out based on approximate theories for a thermoviscous diffusion layer derived from the thermoacoustic-wave equation taking account of the temperature dependence of the viscosity and the heat conductivity. Assuming that the stack consists of many pores axially and that the thickness of the diffusion layer is much thicker than the pore radius, the diffusion wave equation with higher-order terms included is applied for the gas in the pores of the stack. For the gas outside of the pores, the theory of a thin diffusion layer is applied. In a section called the buffer tube over which the temperature relaxes from that at the hot end of the stack to room temperature, the effects of the temperature gradient are taken into account. With plausible temperature distributions specified on the walls of the stack and the buffer tube, the solutions to the equations in both theories are obtained and a frequency equation is finally derived analytically by matching the conditions at the junctions between the various sections. Seeking a real solution to the frequency equation, marginal conditions of instability are obtained numerically not only for the one-wave mode but also for the two-wave mode, where the tube length corresponds to one wavelength and two wavelengths, respectively. It is revealed that the marginal conditions depend not only on the thickness of the diffusion layer but also on the porosity of the stack. Although the toroidal geometry allows waves to be propagated in both senses along the tube, it is found that the wave propagating in the sense from the cold to the hot end through the stack is always greater, so that a travelling wave in this sense emerges as a whole. The spatial and temporal variations of excess pressure and mean axial velocity averaged over the cross-section of a flow passage are displayed for the two modes of oscillations at the marginal state. The spatial distribution of mean acoustic energy flux (acoustic intensity) over one period is also shown. It is unveiled that the energy flux is generated only in the stack, and it decays slowly in the other sections by lossy effects due to a boundary layer. Mechanisms for the generation of the acoustic energy flux are also discussed.

The analysis for the physical mechanism of the long-wave instability in liquid film flow is extended to take into account the presence of a surfactant of arbitrary solubility. The Navier–Stokes equations are supplemented by mass balances for the concentrations at the interface and in the bulk, by a Langmuir model for adsorption kinetics at the interface, and are expanded in the limit of long-wave disturbances. The longitudinal flow perturbation, known to result from the perturbation shear stress which develops along the deformed interface, is shown to contribute a convective flux that triggers an interfacial concentration gradient. This gradient is, at leading order, in phase with the interfacial deformation, and as a result produces Marangoni stresses that stabilize the flow. The strength of the interfacial concentration gradient is shown to be maximum for an insoluble surfactant and to decrease with increasing surfactant solubility. The decrease is explained in terms of the spatial phase of mass transfer between interface and bulk, which mitigates the interfacial flux by the flow perturbation and leads to the attenuation of Marangoni stresses. Higher-order terms are derived, which provide corrections for disturbances of finite wavelength.

In the Dagan & Tulin (J. Fluid Mech., vol. 51, 1972, pp. 529–543) model of ship waves, a blunt ship moving at low speeds can be modelled as a two-dimensional semi-infinite body. A central question for these reduced models is whether a particular ship design can minimize, or indeed eliminate, the wave resistance. In the previous part of our work (Trinh et al., J. Fluid Mech., vol. 685, 2011, pp. 413–439), we demonstrated why a single corner can never be made waveless. In this accompanying paper, we continue our investigations with the study of more general piecewise-linear, or multi-cornered ships. By using exponential asymptotics, we demonstrate how the production of waves can be directly ascertained by the positions and angles of the corners. In particular, this theory answers the question raised by Farrow & Tuck (J. Austral. Math. Soc. B, vol. 36, 1995, pp. 424–437) as to why certain bulbous-like obstructions can minimize the production of waves. General results for wavelessness are given for a class of hulls, and numerical computations of the nonlinear ship-wave problem are used to confirm analytical predictions. Finally, we discuss open questions regarding hulls without corners and more general three-dimensional bluff bodies.

In this work, the scaling statistics of the dissipation along Lagrangian trajectories are investigated by using fluid tracer particles obtained from a high-resolution direct numerical simulation with $\mathit{Re}_{\lambda }=400$. Both the energy dissipation rate $\epsilon $ and the local time-averaged $\epsilon _{\tau }$ agree rather well with the lognormal distribution hypothesis. Several statistics are then examined. It is found that the autocorrelation function $\rho (\tau )$ of $\ln (\epsilon (t))$ and variance $\sigma ^2(\tau )$ of $\ln (\epsilon _{\tau }(t))$ obey a log-law with scaling exponent $\beta '=\beta =0.30$ compatible with the intermittency parameter $\mu =0.30$. The $q{\rm th}$-order moment of $\epsilon _{\tau }$ has a clear power law on the inertial range $10<\tau /\tau _{\eta }<100$. The measured scaling exponent $K_L(q)$ agrees remarkably with $q-\zeta _L(2q)$ where $\zeta _L(2q)$ is the scaling exponent estimated using the Hilbert methodology. All of these results suggest that the dissipation along Lagrangian trajectories could be modelled by a multiplicative cascade.

The effects of surface corrugation with small amplitude on the growth of Tollmien–Schlichting (T–S) waves were examined experimentally in a zero-pressure-gradient boundary layer. Two- and three-dimensional corrugations of sinusoidal geometry with wavelengths of the same order as that of the two-dimensional T–S wave were considered. The corrugation amplitudes were one order of magnitude smaller than the boundary-layer displacement thickness. Streamwise growth of T–S waves on the corrugated walls was compared with that in the boundary layer on the smooth surface. A distinct difference was found in the destabilizing effect between the two- and three-dimensional corrugations. The two-dimensional corrugation significantly enhanced the growth of two-dimensional T–S waves even when the corrugation amplitude was only ∼10% of the displacement thickness. On decreasing the corrugation amplitude, the growth rate of two-dimensional T–S waves asymptotically approached that in the smooth-wall case. On the other hand, the three-dimensional corrugation had only a small influence on the growth of two-dimensional T–S waves even when the corrugation amplitude was as large as 20% of the displacement thickness. For three-dimensional corrugations, however, a pair of oblique waves was generated and developed by an interaction between the two-dimensional T–S wave and the corrugation-induced mean-flow distortion for the corrugation wavelength considered. On increasing the corrugation amplitude, the oblique waves generated were increased in amplitude and thus significantly influenced the secondary instability process.

The turbulent energy cascade in an upward or downward bubbly pipe flow with a Reynolds number of 1.5 × 104 was experimentally investigated in order to examine the effects of the flow direction on the turbulence modifications by bubbles. The bubble diameter was approximately 1 mm. The combination of a particle tracking velocimetry (PTV) system with Kolmogorov-order spatial and temporal resolutions and a shape projection imaging (SPI) system was used to simultaneously capture the liquid and bubble motions. The physical mechanisms of turbulence modification at each length scale, or in wavenumber space, were investigated by introducing a filtering-based scaling analysis, in which the filtering techniques derived from large eddy simulation (LES) were applied to the PTV measurements. The analysis can be used to examine the turbulent kinetic energy (TKE) exchange between bubbles and flows at each wavenumber. We observed significant differences in the flow statistics and turbulent energy budget of upward and downward flows, which are due to the sign of the relative velocity of bubbles. A negative relative velocity (downward flow) induces greater modifications in the energy budget than a positive relative velocity (upward flow), which suggests that the bubble-transport term of the turbulent energy is greater when the flow has to push down the bubbles. The flow provides more energy to the bubbles when it pushes them in the downward direction. The flow will also receive and dissipate more energy from the bubbles in a downward flow compared with an upward flow due to the greater transverse motion of the bubbles. The analysis introduced in the present study shows that the energy transfer from large to small scales is decreased in an upward flow and is increased in a downward flow. Similarly, the sign of the bubble term indicates that turbulent flow receives energy from bubbles in an upward flow, while it transfers energy to bubbles in a downward flow. We also observed that this energy transport is approximately 10 times larger in a downward flow than in an upward flow.

We investigate the low-Weber-number flow of a liquid curtain bridged between two vertical edge guides and a pool surface. Three flow patterns, namely, steady vertical flow, steady oblique flow, and oscillatory oblique flow, are observed in our experiment. These patterns are caused by the Coanda effect of the jet around the meniscus that is formed in the matching region common to the liquid curtain and the pool surface. Here, the deflection angle of the jet is greater than $90^\circ $. The equation describing the motion of the liquid curtain applicable for a finite curtain slope is obtained using the intrinsic coordinate system in which the distance along the curtain is selected as one of the coordinates, and the equation of the meniscus motion is derived by considering the conservation of momentum. The curtain deformations of oblique flows are analysed by generating numerical simulations of these equations, and the simulation results are then compared with experimental results. Also, the period of oscillatory oblique flow is discussed and explained via the response analyses of the curtain, and the result shows that the period of the oscillations is close to that of the most amplified mode of the liquid curtain. Further, the detachment angle of the jet is discussed in relation to the Coanda effect of the jet in the meniscus.

This paper discusses the interaction between droplets and entrained turbulent air flow in the far-downstream locations of a confined polydispersed isothermal spray. Simultaneous and planar measurements of droplet and gas velocities in the spray along with droplet size are obtained with the application of a novel experimental technique, developed by Hardalupas et al. (Exp. Fluids, vol. 49, 2010, pp. 417–434), which combines interferometric laser imaging for droplet sizing (ILIDS) with particle image velocimetry (PIV). These measurements quantified the spatial correlation coefficients of droplet–gas velocity fluctuations ($R_{dg}$) and droplet–droplet velocity fluctuations ($R_{dd}$) conditional on droplet size classes, for various separation distances, and for axial and cross-stream velocity components. At the measurement location close to the spray edge, with increasing droplet size, $R_{dg}$ was found to increase in axial direction and decrease in cross-stream direction. This suggests that as the gas-phase turbulence becomes more anisotropic away from the spray axis, the gravitational influence on droplet–gas correlated motion tends to increase. The effective length scales of the correlated droplet–gas motion were evaluated and compared with that for gas and droplet motion. The role of different turbulent eddies of the gas flow on the droplet–gas interaction was examined. The flow structures were extracted using proper orthogonal decomposition (POD) of the instantaneous gas velocity data, and their contribution on the spatial droplet–gas velocity correlation was evaluated, which quantified the momentum transfer between the two phases at different length scales of the gas flow. The droplets were observed to augment turbulence for the first three POD modes (larger scales) and attenuate it for the rest of the modes (smaller scales). It has been realized that apart from droplet Stokes number and mass loading, the dynamic range of length scales of the gas flow and the relative turbulent kinetic energy content of the flow structures (POD modes) must be considered in order to conclude if the droplets enhance or reduce the carrier-phase turbulence especially at the lower wavenumbers.

Triad interactions, involving a set of wave-vectors $\{\pm \boldsymbol {k}, \pm \boldsymbol {p}, \pm \boldsymbol {q}\}$, with $ \boldsymbol {k} + \boldsymbol {p}+ \boldsymbol {q}=0$, are considered, and the results of triad truncation are compared with the results of exact Euler evolution starting from the same initial conditions. The essential two-dimensionality of the triad interaction is used to separate the problem into two parts: a nonlinear two-dimensional flow problem in the triad plane, and a linear problem of ‘passive scalar’ type for the evolution of the component of velocity perpendicular to this plane. Several examples of triad evolution are presented in detail, and the marked contrast with Euler evolution is demonstrated. It is known that energy and helicity are conserved under triad truncation; it is shown that the ‘in-plane’ energy and enstrophy are also conserved. However, it is also shown that, in general, the evolution of the vorticity under triad truncation cannot be represented as transport by any divergence-free velocity field, with the consequence that the detailed topology of the vorticity field is not conserved under this truncation.

The Rotne–Prager–Yamakawa (RPY) approximation is a commonly used approach to model the hydrodynamic interactions between small spherical particles suspended in a viscous fluid at a low Reynolds number. However, when the particles overlap, the RPY tensors lose their positive definiteness, which leads to numerical problems in the Brownian dynamics simulations as well as errors in calculations of the hydrodynamic properties of rigid macromolecules using bead modelling. These problems can be avoided by using regularizing corrections to the RPY tensors; so far, however, these corrections have only been derived for equal-sized particles. Here we show how to generalize the RPY approach to the case of overlapping spherical particles of different radii and present the complete set of mobility matrices for such a system. In contrast to previous ad hoc approaches, our method relies on the direct integration of force densities over the sphere surfaces and thus automatically provides the correct limiting behaviour of the mobilities for the touching spheres and for a complete overlap, with one sphere immersed in the other one. This approach can then be used to calculate hydrodynamic properties of complex macromolecules using bead models with overlapping, different-sized beads, which we illustrate with an example.

In 1880 Lord Kelvin analysed the linearized inviscid oscillations of a Rankine vortex as part of a theory of vortex atoms. These eponymously named neutrally stable modes are, however, exceptional regular oscillations that make up the discrete spectrum of the Rankine vortex. In this paper, we examine the singular oscillations that make up the continuous spectrum (CS) and span the entire base state range of frequencies. In two dimensions, the CS eigenfunctions have a twin-vortex-sheet structure similar to that known from earlier investigations of parallel flows with piecewise linear velocity profiles. The vortex sheets are cylindrical, being threaded by axial lines, with one sheet at the edge of the core and the other at the critical radius in the irrotational exterior; the latter refers to the radial location at which the fluid co-rotates with the eigenmode. In three dimensions, the CS eigenfunctions have core vorticity and may be classified into two families based on the singularity at the critical radius. For the first family, the singularity is a cylindrical vortex sheet threaded by helical vortex lines, while for the second family it has a localized dipole structure with radial vorticity. The presence of perturbation vorticity in the otherwise irrotational exterior implies that the CS modes, unlike the Kelvin modes, offer a modal interpretation for the (linearized) interaction of the Rankine vortex with an external vortical disturbance. It is shown that an arbitrary initial distribution of perturbation vorticity, both in two and three dimensions, may be evolved as a superposition over the discrete and CS modes; this modal representation being equivalent to a solution of the corresponding initial value problem. For the restricted case of an initial axial vorticity distribution in two dimensions, the modal representation may be generalized to a smooth vortex. Finally, for the three-dimensional case, the analogy between rotational flows and stratified shear flows, and the known analytical solution for stratified Couette flow, are used to clarify the singular manner in which the modal superposition for a smooth vortex approaches the Rankine limit.

Data on the internal velocity distribution of flowing sediment–fluid mixtures such as debris flows are rare, but necessary for model development and testing. A probe to measure the mean particle velocity at different depths and different locations within experimental debris flows in a 4 m diameter rotating drum was developed. In addition, the flow depth, basal normal stress and basal pore fluid pressure were also measured. Results show that for a given sediment–fluid mixture the velocity profiles collapse to distinct non-dimensional profiles. Macroscopic flow behaviour shows great similarity, with mean surface slopes weakly dependent on the shear rate for water-saturated gravel, but strongly shear-rate-dependent when pores are filled with mud. Poorly sorted material with a high content of fines produced fluid pressures close to normal stress and sidewall friction had a strong effect on the flow pattern. Our results reveal variability in profile characteristics for flows displaying similar macro-dynamics and provide data for model testing.

The secondary instability of boundary layer streaks is investigated by means of direct stability analysis. The base flow is computed in direct simulations of bypass transition. The random nature of the free-stream perturbations causes the formation of a spectrum of streaks inside the boundary layer, with breakdown to turbulence initiated by the amplification of localized instabilities of individual streaks. The capability of the instability analysis to predict the instabilities which are observed in the direct numerical simulation is established. Furthermore, the analysis is shown to identify the particular streaks that break down to turbulence farther downstream. Two particular configurations of streaks regularly induce the growth of these localized instabilities: low-speed streaks that are lifted towards the edge of the boundary layer, and the local overlap between high-speed and low-speed streaks inside the boundary layer. It is established that the underlying modes can be ascribed to the general classification of inner and outer modes which was introduced by Vaughan & Zaki (J. Fluid Mech., vol. 681, 2011, pp. 116–153). Statistical evaluations show that Blasius boundary layers favour the amplification of outer instabilities. Adverse pressure gradient promotes breakdown to turbulence via the inner mode.

The non-classical gas dynamics of binary mixtures of organic fluids in the vapour phase is investigated for the first time. A predictive thermodynamic model is used to compute the relevant mixture properties, including its critical point coordinates and the local value of the fundamental derivative of gas dynamics $\Gamma $. The considered model is the improved Peng–Robinson Stryjek–Vera cubic equation of state, complemented by the Wong–Sandler mixing rules. A finite thermodynamic region is found where the nonlinearity parameter $\Gamma $ is negative and therefore non-classical gas dynamics phenomena are admissible. A non-monotone dependence of $\Gamma $ on the mixture composition is observed in the case of binary mixtures of siloxane and perfluorocarbon fluids, with the minimum value of $\Gamma $ in the mixture being always larger than that of its more complex component. The observed dependence indicates that non-ideal mixing has a strong influence on the gas dynamics behaviour, either classical or non-classical, of the mixture. Numerical experiments of the supersonic expansion of a mixture flow around a sharp corner show the transition from the classical configuration, exhibiting an isentropic rarefaction fan centred at the expansion corner, to non-classical ones, including mixed expansion waves and rarefaction shock waves, if the mixture composition is changed.

The aim of this investigation was to experimentally examine the surface pressures and resulting forces on an individual sediment grain whose size is comparable to the scales of the turbulent channel flow in an effort to discern details of the flow/grain interaction. This was accomplished by measuring the pressure fluctuations on the surface of a coarse, fully exposed, spherical grain resting upon a bed of identical grains in open channel turbulent flow. This spherical particle was instrumented with low-range, high-frequency-response pressure transducers to measure the individual surface pressures simultaneously on its front, back, top and bottom. The local flow velocity was measured synchronously with a laser Doppler velocimeter. The flow and sediment are near threshold conditions for entrainment with the channel and particle Reynolds numbers varying between 31 000–39 000 and 330–440 respectively. The emphasis was on determining the characteristics of the flow field with the potential to dislodge a spherical grain under uniform flow conditions as well as in the wake of a circular cylinder placed spanwise across the flow in otherwise fully developed open channel flow. It is concluded that the streamwise velocity near the bed is most directly related to those force events (and associated individual surface pressure distributions) crucial for particle entrainment. The lift force was observed to momentarily reach values which can be consequential for particle stability, although it is poorly correlated with the fluctuating normal velocity component. Turbulence intensity near the bed, rather than being the causative factor for increased force fluctuations, was shown to be an indicator of changes in the average lift force experienced by the grain during the application of extreme drag forces, at least for this particular flow condition (the upstream, spanwise-mounted circular cylinder). This effect is known to alter the sediment transport rates significantly. The characteristics of the temporal durations of flow events about the local maxima in the stagnation pressure, drag and lift forces, using a conditional sampling method, revealed the prevalence of sweep-type near-bed flow events in generating favourable conditions for particle dislodgement, although the dominant feature is the positive streamwise velocity fluctuation, not the normal velocity component. The duration of such events was the highest in the fourth and first quadrants in the $u,w$ plane, inducing high impulses on the grain.

Against the common wisdom that wall slip plays only a minor role in global flow characteristics, here we demonstrate theoretically for the displacement of a long bubble in a slippery channel that the well-known Bretherton $2/3$ law can break down due to a fraction of wall slip with the slip length $\lambda $ much smaller than the channel depth $R$. This breakdown occurs when the film thickness $h_{\infty } $ is smaller than $\lambda $, corresponding to the capillary number $Ca$ below the critical value $Ca^{\ast } \sim (\lambda /R)^{3 / 2}$. In this strong slip regime, a new quadratic law $h_{\infty } /R \sim Ca^{2} (R/\lambda )^{2}$ is derived for a film much thinner than that predicted by the Bretherton law. Moreover, both the $2/3$ and the quadratic laws can be unified into the effective $2/3$ law, with the viscosity $\mu $ replaced by an apparent viscosity $\mu _{app}= \mu h_{\infty } /({\lambda } + h_{\infty })$. A similar extension can also be made for coating over textured surfaces where apparent slip lengths are large. Further insights can be gained by making a connection with drop spreading. We find that the new quadratic law can lead to $\theta _{d} \propto Ca^{1 / 2} $ for the apparent dynamic contact angle of a spreading droplet, subsequently making the spreading radius grow with time as $r \propto t^{1 / 8}$. In addition, the precursor film is found to possess $\ell _{f} \propto Ca^{ - 1 / 2}$ in length and therefore spreads as $\ell _{f} \propto t^{1 / 3}$ in an anomalous diffusion manner. All these features are accompanied by no-slip-to-slip transitions sensitive to the amount of slip, markedly different from those on no-slip surfaces. Our findings not only provide plausible accounts for some apparent departures from no-slip predictions seen in experiments, but also offer feasible alternatives for assessing wall slip effects experimentally.