Refine listing
Actions for selected content:
Nanjing Special Issue on K-theory, number theory and geometry
Volume
12 -
Issue 1
-
August 2013
Contents
Editorial
Preface
-
- Published online by Cambridge University Press:
- 30 October 2013, p. 1
-
- Article
-
- You have access
- Export citation
Research Article
Remarks on Murre's conjecture on Chow groups
-
- Published online by Cambridge University Press:
- 30 October 2013, pp. 3-14
-
- Article
- Export citation
A Bloch-Wigner complex for SL2
-
- Published online by Cambridge University Press:
- 22 April 2013, pp. 15-68
-
- Article
- Export citation
K-cycles for twisted K-homology
-
- Published online by Cambridge University Press:
- 23 May 2013, pp. 69-98
-
- Article
- Export citation
Cohomology for Generalized Bredon Coefficient Systems and Higher K-Theory
-
- Published online by Cambridge University Press:
- 19 April 2012, pp. 99-113
-
- Article
- Export citation
Iwasawa Theory for K2n

-
- Published online by Cambridge University Press:
- 02 May 2013, pp. 115-123
-
- Article
- Export citation
Relative reciprocities on Dedekind domains
-
- Published online by Cambridge University Press:
- 03 June 2013, pp. 125-136
-
- Article
- Export citation
Tame kernels and second regulators of number fields and their subfields
-
- Published online by Cambridge University Press:
- 17 July 2013, pp. 137-165
-
- Article
- Export citation
On λ-invariants of number fields and étale cohomology
-
- Published online by Cambridge University Press:
- 05 July 2013, pp. 167-181
-
- Article
- Export citation
On a local to global principle in étale K-groups of curves
-
- Published online by Cambridge University Press:
- 17 May 2013, pp. 183-201
-
- Article
- Export citation
Hermitian periodicity and cohomology of infinite orthogonal groups
-
- Published online by Cambridge University Press:
- 23 May 2013, pp. 203-211
-
- Article
- Export citation
Front matter
KAG volume 12 issue 1 Cover and Front matter
-
- Published online by Cambridge University Press:
- 30 October 2013, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back matter
KAG volume 12 issue 1 Cover and Back matter
-
- Published online by Cambridge University Press:
- 30 October 2013, pp. b1-b2
-
- Article
-
- You have access
- Export citation

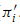 , 0 ≤
, 0 ≤ 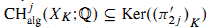 )
) be a generalized based category (see definition 1.2). In this paper, we construct a cohomology theory in the category
be a generalized based category (see definition 1.2). In this paper, we construct a cohomology theory in the category 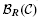 of contravariant functors:
of contravariant functors: 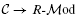 where
where 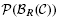 of finitely generated projective objects in
of finitely generated projective objects in 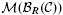 of finitely generated objects in
of finitely generated objects in 
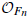 denote its ring of integers. We establish an analogue of the classical Iwasawa theorem for the orders of
denote its ring of integers. We establish an analogue of the classical Iwasawa theorem for the orders of  ) ∈
) ∈ 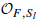 then
then