Definition. For ordinals α ≤ κ, = [X]α = {p ⊆ X ∣ ot (p) = α}. For α ≤, κ, κ a cardinal, holds iff for every partition F: [Κ]κ → A, there is an X ∈ [Κ]κ with F constant on [X]α. X is called homogeneous for F. When A = 2 the subscript is omitted.

It has been known since the sixties that for finite exponents all such properties are equivalent, i.e., κ→ (κ)2 iff for all n < ω, β < κ. The infinite case is much more difficult, though it is not actually known to be different. The usual methods for dealing with partitions fail, and as the weakest of these properties violates the Axiom of Choice, many techniques are unavailable.

It is extremely unlikely that an infinite exponent can be increased, but infinite subscripts are generally possible.

Theorem. If ∥ α ∥ · 2 ≤ α, then κ → (κ)α implies for all λ < κ.

In §1, we show the subscript can be any λ < κ. In §2 we extend this to 2λ. In §3 we discuss the possibilities with limited amounts of well-ordered choice, and apply the theorem to the problem of obliging ordinals. Except in §3, we assume no choice. For the story of finite exponents, see [1]. For the relationship between infinite-exponent partition relations and choice, see [4].

An effective translation of the fact that any infinite ordered set contains an infinite ascending or descending sequence is that any infinite recursive set A ⊆ Q has a recursive subset with order type ω or ω*. Tennenbaum's theorem states that this translation is false, and there is a counterexample A with order type ω + ω*. Tennenbaum suggested that this counterexample is an infinite recursive linearly ordered set which is effectively finite, and that the collection of all such counterexamples could provide a concrete model of nonstandard arithmetic. The purpose of this paper is to determine the collection of order types for which there is a counterexample.

It is readily seen that any counterexample to the effective translation must have order type ω + Z · α + ω* for some α [2], [3]. Let be the collection of order types α for which there is a counterexample. As a test case, we have previously shown that contains the constructive scattered orderings [3], [4]. In this paper we determine exactly which order types are in . We easily show that if ω+ Z · α + ω* is recursive, then α is . The main result is that . Consequently, .

Classical set theory is considered as the framework of contemporary mathematics. But there are aspects of our understanding of the real world about what it is not clear that they are described in Cantor's set theory in the best possible way. For example the formalization of some intuitive concepts such as the description of vague properties (which led to the theory of fuzzy sets) and the interpretation of the notion of infinitely small quantities (modelled by nonstandard models) is not quite simple in the classical set theory. Therefore it is natural to look for an alternative to Cantor's set theory which would enable us to formalize these considerations more naturally and which would be a sufficiently strong framework for mathematics at the same time.

The alternative set theory which was created by P. Vopěnka (cf. [V]) is an attempt to construct a theory that could serve as an alternative to Cantor's set theory. The ideas of the alternative set theory make it possible to constitute a new approach to mathematics. An effort to formalize mathematically our intuitive concepts once more is made, and moreover it seems that one can obtain in the alternative set theory mathematical formalizations of concepts which up to now were not adequately formalized.

In the alternative set theory we can build up all essential parts of mathematics. Development of the classical disciplines is described in [V]. Let us mention a few further results obtained in the alternative set theory: a connection between the discrete and the continuous on the basis of which it is possible to define topology (cf. [V]), investigation of motion (cf. [V]), construction of different types of σ-additive ultrafilters (cf. [S-V4]), classification of classes (measurement of vagueness of properties; cf. [Č-V]), possibility of definition of notions of nonstandard methods (cf. [S-V 1]) and valuations of ideals (cf. [M]). A series of articles has been written developing mathematics in the alternative set theory (for the full list of papers cf. [S]). For some remarks concerning the connection between the alternative set theory and nonstandard methods, the reader is refered to [S 4].

Lachlan's nondiamond theorem [7, Theorem 5] asserts that there is no embedding of the four-element Boolean algebra (diamond) in the recursively enumerable degrees which preserves infima, suprema, and least and greatest elements. Lachlan observed that, essentially by relativization, the theorem can be extended to

Using the Sacks splitting theorem he concluded that there exists a pair of r.e. degrees which does not have an infimum, thus showing that the r.e. degrees do not form a lattice.

We will first prove the following extension of (1):

where an r.e. degree a is non-b-cappable if . From (2) we obtain more information about pairs of r.e. degrees without infima: For every nonzero low r.e. degree there exists an incomparable one such that the two degrees do not have an infimum and there is an r.e. degree which is not half of a pair of incomparable r.e. degrees which has an infimum in the low r.e. degrees. Probably the most interesting corollary of (2) is that the join of any cappable r.e. degree (i.e. half of a minimal pair) and any low r.e. degree is incomplete. Consequently there is an incomplete noncappable degree above every incomplete r.e. degree. Cooper's result [3] that ascending sequences of uniformly r.e. degrees can have minimal upper bounds in the set R of r.e. degrees is another corollary of (2).

In [J] R. Jensen proved that any model of ZFC can be generically extended to a model of ZFC + (∃a ⊆ ω)(V = L[a]). This extension was made via a class P of forcing conditions. One can ask what can be said about the class HOD in such extensions. In particular one can ask whether V = HOD holds in Jensen's model. A full answer to this question is given by the following assertion:

Theorem. Let N = L[a] be given by forcing with Jensen's conditions (the classP).

Then:

1. N ⊨ V ≠ HOD,

2. N ⊨ (∀n < ω)(HODn + 1 ⊈ HODω).

3. N ⊨ (∀α ≥ ω)(HODα = HODω), or equivalently HODω + 1 = HODω.

The result is established by investigating homogeneity properties of certain complete Boolean algebras that are associated with the partial orderings of forcing conditions for coding using almost disjoint sets.

We explain shortly the first of our two technical results: It is rather well known that if we have a subset then by performing threefold almost disjoint coding we come to a set a0 ⊆ ω such that ; cf. [JS]. This is achieved by iterating over almost disjoint forcing . That means we first code by a subset , of [ω1ω2) using the almost disjoint forcing conditions . Then we code by aω, contained in [ω, ω1), using forcing with over .

A hundred years ago, Frege proposed a logical definition of the natural numbers based on the following idea:

He replaced this circular definition by the following one:

He tried afterwards to found his theory over a notion of class satisfying a general comprehension principle:

Russell quickly derived a contradiction from this principle (the famous Russell's paradox) but saved Frege's arithmetic with his theory of types based on the following comprehension principle:

In 1979, talking at the Claude Bernard University in Lyon, I remarked that 3 types suffice to provide Frege's arithmetic, showing in fact that PA2 (second order Peano arithmetic) holds in TT3 + AI (theory of types 0, 1, 2 plus a suitable axiom of infinity). I asked whether TT3 + AI was a conservative extension of PA2. Pabion [3] gave a positive answer by a subtle use of the Fraenkel-Moskowski method. This result will be improved in the present paper, with a view to getting models of NF3 + AI in which Frege's arithmetic forms a model isomorphic to a given countable model of PA2.

(1.1) A well-known example of a theory with built-in Skolem functions is (first-order) Peano arithmetic (or rather a certain definitional extension of it). See [C-K, pp. 143, 162] for the notion of a theory with built-in Skolem functions, and for a treatment of the example just mentioned. This property of Peano arithmetic obviously comes from the fact that in each nonempty definable subset of a model we can definably choose an element, namely, its least member.

(1.2) Consider now a real closed field R and a nonempty subset D of R which is definable (with parameters) in R. Again we can definably choose an element of D, as follows: D is a union of finitely many singletons and intervals (a, b) where – ∞ ≤ a < b ≤ + ∞; if D has a least element we choose that element; if not, D contains an interval (a, b) for which a ∈ R ∪ { − ∞}is minimal; for this a we choose b ∈ R ∪ {∞} maximal such that (a, b) ⊂ D. Four cases have to be distinguished:

(i) a = − ∞ and b = + ∞; then we choose 0;

(ii) a = − ∞ and b ∈ R; then we choose b − 1;

(iii) a ∈ R and b ∈ = + ∞; then we choose a + 1;

(iv) a ∈ R and b ∈ R; then we choose the midpoint (a + b)/2.

It follows as in the case of Peano arithmetic that the theory RCF of real closed fields has a definitional extension with built-in Skolem functions.

Under the assumption that all “rules” are recursive (ECT) the statement Cont(NN, N) that all functions from NN to N are continuous becomes equivalent to a statement KLS in the language of arithmetic about “effective operations”. Our main result is that KLS is underivable in intuitionistic Zermelo-Fraenkel set theory + ECT. Similar results apply for functions from R to R and from 2N to N. Such results were known for weaker theories, e.g. HA and HAS. We extend not only the theorem but the method, fp-realizability, to intuitionistic ZF.

If L is a first-order language, then an L-structure A is called quantifier-eliminable (QE) if every L-formula is equivalent in A to a formula without quantifiers.

The classification problem for QE groups and rings has received attention in work by Berline, Boffa, Cherlin, Feigner, Macintyre, Point, Rose, the present authors, and others. In [1], Berline and Cherlin reduced the problem for rings of prime characteristic p to that for nilrings, but also constructed 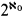countable QE nilrings of characteristic p. Likewise, in [3], we constructed 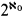countable QE nil-2 groups. Both results can be viewed as “nonstructure theorems”, in that they provide negative evidence for any attempt at classification. In the present paper we show that the situation is equally bad (or rich, depending on one's point of view) for commutative rings:

Theorem 1. For any odd prime p, there exist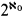countable QE commutative nilrings of characteristic p.

This solves a problem posed in [1]. We remark that the examples we produce are uniformly locally finite, hence ℵ0-categorical. A more algebraic description is that each of our rings R is uniformly locally finite (in fact, R3 = 0) and homogeneous, in the sense that any isomorphism of finitely generated subrings extends to an automorphism of R.

Theorem 1 does not cover the case p = 2, and we show that for commutative rings this case is in fact exceptional:

Theorem 2. There exist exactly two nonisomorphic countably infinite QE commutative nilrings of characteristic 2.