David Pagni drew attention to a result which is ascribed by Dickson [2, p. 286] to Liouville (1857), that the sum of the cubes of the number of divisors of each of the divisors of an integer, is equal to the square of their sum. For example, the divisors of 6 are 1, 2, 3, and 6, which have 1, 2, 2, and 4 divisors respectively, and 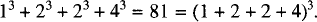
Pagni observed, as have others, including Mason et al. [4, p. 179], that the reason Liouville’s result works is because the numbers generated are element-by-element products of sequences of the form {1, 2, ..., t}, which are well known to have the sum of their cubes equal to the square of their sum.