Let 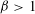$\unicode[STIX]{x1D6FD}>1$ be a real number and define the 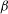$\unicode[STIX]{x1D6FD}$ -transformation on 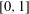$[0,1]$ by 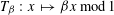$T_{\unicode[STIX]{x1D6FD}}:x\mapsto \unicode[STIX]{x1D6FD}x\,\text{mod}\,1$ . Further, define

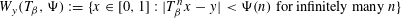$$\begin{eqnarray}W_{y}(T_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D6F9}):=\{x\in [0,1]:|T_{\unicode[STIX]{x1D6FD}}^{n}x-y|<\unicode[STIX]{x1D6F9}(n)\text{ for infinitely many }n\}\end{eqnarray}$$

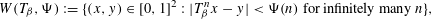$$\begin{eqnarray}W(T_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D6F9}):=\{(x,y)\in [0,1]^{2}:|T_{\unicode[STIX]{x1D6FD}}^{n}x-y|<\unicode[STIX]{x1D6F9}(n)\text{ for infinitely many }n\},\end{eqnarray}$$

$\unicode[STIX]{x1D6F9}:\mathbb{N}\rightarrow \mathbb{R}_{{>}0}$

$\unicode[STIX]{x1D6F9}(n)\rightarrow 0$

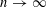$n\rightarrow \infty$

We study the average value of the divisor function $\unicode[STIX]{x1D70F}(n)$ for $n\leqslant x$ with $n\equiv a~\text{mod}~q$ . The divisor function is known to be evenly distributed over arithmetic progressions for all $q$ that are a little smaller than $x^{2/3}$ . We show how to go past this barrier when $q=p^{k}$ for odd primes $p$ and any fixed integer $k\geqslant 7$ .

In this paper we show that sieve methods used previously to investigate primes in short intervals and corresponding Goldbach-type problems can be modified to obtain results on primes in Beatty sequences in short intervals.

We prove that among all flag triangulations of manifolds of odd dimension $2r-1$ , with a sufficient number of vertices, the unique maximizer of the entries of the $f$ -, $h$ -, $g$ - and $\unicode[STIX]{x1D6FE}$ -vector is the balanced join of $r$ cycles. Our proof uses methods from extremal graph theory.

We show that if a finite, large enough subset $A$ of an arbitrary abelian group $G$ satisfies the small doubling condition $|A+A|\leqslant (\log |A|)^{1-{\it\varepsilon}}|A|$, then $A$ must contain a three-term arithmetic progression whose terms are not all equal, and $A+A$ must contain an arithmetic progression or a coset of a subgroup, either of which is of size at least $\exp [c(\log |A|)^{{\it\delta}}]$. This extends analogous results obtained by Sanders, and by Croot, Łaba and Sisask in the case where $G=\mathbb{Z}$.

We present a construction of a measure-zero Kakeya-type set in a finite-dimensional space $K^{n}$ over a local field with finite residue field. The construction is an adaptation of the ideas appearing in works by Sawyer [Mathematika34(1) (1987), 69–76] and Wisewell [Mathematika51(1–2) (2004), 155–162]. The existence of measure-zero Kakeya-type sets over discrete valuation rings is also discussed, giving an alternative construction to the one over $\mathbb{F}_{\ell }\unicode[STIX]{x27E6}t\unicode[STIX]{x27E7}$ presented by Dummit and Hablicsek [Mathematika59(2) (2013), 257–266].

We study ultrasymmetric spaces in the case in which the fundamental function belongs to a limiting class of quasiconcave functions. In the process, we study limiting cases of $J$ interpolation spaces and establish new $J$ – $K$ identities as well as a reiteration theorem for these limiting interpolation methods.

The illumination problem may be phrased as the problem of covering a convex body in Euclidean $n$-space by a minimum number of translates of its interior. By a probabilistic argument, we show that, arbitrarily close to the Euclidean ball, there is a centrally symmetric convex body of illumination number exponentially large in the dimension.

In a $d$ -dimensional convex body $K$ random points $X_{0},\ldots ,X_{d}$ are chosen. Their convex hull is a random simplex. The expected volume of a random simplex is monotone under set inclusion if $K\subset L$ implies that the expected volume of a random simplex in $K$ is smaller than the expected volume of a random simplex in $L$ . Continuing work of Rademacher [On the monotonicity of the expected volume of a random simplex. Mathematika58 (2012), 77–91], it is shown that moments of the volume of random simplices are, in general, not monotone under set inclusion.