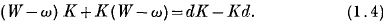Let N be a natural number, and let be a non-empty subset of the set of integers

Let Z be the number of elements of . We denote by Z(p, h) the number of elements of falling into the congruence class h modulo p, so that

In a recent paper I. J. Schoenberg [1] considered relations

where the av are rational integers and the ζv are roots of unity. We may in (1) replace all negative coefficients av by −av replacing at the same time ζv by −ζv so that we may, if it is convenient, assume that all av are positive. If we do this and arrange the ζr so that their arguments do not decrease with v then (1) can, as suggested by Schoenberg (oral communication) be interpreted as a convex polygon with integral sides whose angles are rational when measured in degrees. Accordingly we shall call a relation (1) a polygon if all av are non-negative. We shall call a polygon (1) k-sided if all av are positive. The polygon is called degenerate if two of the ζv are equal. Schoenberg calls these polygons polar rational polygons (abbreviated prp) because the vectors composing them have rational coordinates in their polar representations. Schoenberg showed that every prp can be obtained as a linear combination with integral positive or negative coefficients of regular p-gons where p is a prime.

Our purpose in this note is to present a natural geometrical definition of the dimension of a graph and to explore some of its ramifications. In §1 we determine the dimension of some special graphs. We observe in §2 that several results in the literature are unified by the concept of the dimension of a graph, and state some related unsolved problems.

Write ‖θ‖ for the distance from the real number θ to the nearest integer. An n-tuple of real numbers (β1, …, βn) will be called badly approximable, if there is constant C > 0 such that

for all positive integers q. As is well known, a single number β is badly approximable if and only if the partial quotients in its continued fraction are bounded.

If an is a sequence of numbers between 0 and 1, then

has infinitely many integral solutions n, l either for almost all real x or for almost no real x[1,4]. Duffin and Schaeffer [2], improving on an earlier theorem of Khintchine [7], proved that for decreasing sequences an, (1) has infinitely many solutions a.e. if and only if Σan diverges. They also gave an example of a sequence an for which Σan diverges, but for which (1) has only finitely many solutions a.e. No general necessary and sufficient condition for (1) to have infinitely many solutions a.e. is known.

A general theory of an elastic-plastic continuum which is valid for non-isothermal deformation and which includes explicit restrictions derived from thermodynamics has been given recently by Green and Naghdi [2]. In the development of this theory, the analysis was carried out for a symmetric plastic strain tensor, although it was noted that it is possible to use instead a plastic strain tensor which is nonsymmetric and this would require only a slight modification of the results.

Green [8] has shown that a constitutive relation of the form

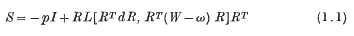
arises as a special case of an incompressible anisotropic simple fluid, where S is the stress tensor or matrix,

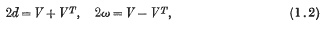
and V is the velocity gradient matrix at time t, all measured in a fixed rectangular cartesian coordinate system. Also, if F is the displacement gradient measured with respect to some curvilinear reference system θi, then

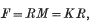
where R is a proper orthogonal matrix, and M and K are positive definite symmetric matrices. In addition

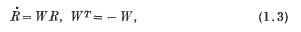
and

This paper is concerned with marginal convection in a self-gravitating sphere of uniform incompressible fluid containing a uniform distribution of heat sources. Its purpose is twofold. The first aim is to present the mathematical argument in a form which, the author believes, is more succinct than that which has been given heretofore. The second aim is to determine the effect of the convective motions upon the moments of inertia of the body and, in the light of the results obtained, examine briefly the hypothesis that the moon is in a state of convection.

1. Let p be a prime, t a positive integer, and ϕ a cubic polynomial with integral coefficients, and an integral constant term. We study the congruence

because of its obvious connection with the equation

In an unpublished, dissertation Cleaver [1] proved the following

Theorem 1. If L is a lattice in euclidean four-space R4 of determinant d(L) = 1 and with no pair of its points within unit distance apart then any four-sphere of radius 1 contains a point of L.

For the solubility of an inhomogeneous polynomial Diophantine equation, there is one well-known necessary, but not sufficient condition; namely the necessary congruence condition (NCC) explained in §2, below. Till recently, no progress had been made with the general cubic equation, because no one knew what else to assume. Examples given here, see (4.3), (5.4), indicate that some rather subtle hypothesis is needed. The first such hypothesis, see Davenport and Lewis [1], was very far from being necessary for the solubility of the equation. It would seem that any supplementary hypothesis which (loosely) is somewhere near necessary and also (together with the NCC) somewhere near sufficient deserves separate detailed investigation before one proceeds to use it.

Let L be a lattice in euclidean n-space Rn of determinant d(L). A well-known problem in the geometry of numbers is whether

(1) if d(L) = l and there exists a sphere centred at the origin containing n linearly independent points of L on its boundary and none other than the origin inside, then any sphere in Rn of radius ½√ contains a point of L.

A solution is obtained of the problem of diffusion from an elevated point source into a turbulent atmospheric flow over a horizontal ground z = 0. The mechanism of the turbulence is the one considered by D. R. Davies [1[ when he obtained a solution of the same problem in the case when the source is at ground level, the specifications for the mean wind velocity V, and for the across-wind diffusivity Ky, and for the vertical diffusivity Kz, being V αzm, Ky αzm, Kz αz1−m, where m is a constant. Predictions of the solution are that the maximum concentration at ground level of the diffusing matter varies inversely as h1·8 in adiabatic conditions, where h is source height, and that the distance downwind from the source to the point where this maximum concentration is attained varies, in these conditions, as h1·3.

Let p be a prime, t a positive integer, and P = P(x1, …, xn) a polynomial over the rational field K, in any number n of variables, of degree k = 2, 3, or 4. We shall consider the congruence

The flows induced by an oscillating cylinder and by a torsionally oscillating disk are considered. For the case of the cylinder attention is restricted to the high frequency case whilst for the disk both the high and low frequency cases are discussed.

The paper discusses the advantages of solving boundaryvalue problems by the use of eigen-function expansions of suitable fourth order differential equations instead of those of second order equations. Some such expansions are constructed, their convergence properties studied and their use in different types of boundary-value problems are discussed.

Let p be an odd prime and denote by [p], the finite field of residue classes, mod p. In Euclidean n-space, let n denote the lattice of points x = (x1, …, xn) with integral coordinates and C = C(n, p), the set of points of n satisfying

Throughout this paper a ring will mean a commutative ring with identity element. If A is an ideal of the ring R and P is a minimal prime ideal of A, then the intersection Q of all P-primary ideals which contain A is called the isolated primary component of A belonging to P. The ideal Q can also be described as the set of all elements x∈R such that xr∈A for some r∈R\P. If {Pα} is the collection of all minimal prime ideals of A and Qα is the isolated primary component of A belonging to Pα, then is called the kernel of A.

This paper is concerned with (a) a new simple method of solution of a wide variety of problems of elastic strips by means of Fourier transforms in the complex plane and (b) a direct solution of the elastic annulus. Continuation of functions into adjacent regions of the plane and the solution of differential-difference equations are seen to be unnecessary complications.

In many investigations into the properties of convex bodies, authors have made use of distance functions ρ(K1K2) which give a measure of the “nearness” of two convex bodies K1 and K2. Sometimes they have introduced new functions to deal with particular problems. The purpose of this paper is to compare and contrast the properties of four of these functions, namely all those (so far as we are aware) which occur in the literature and have the property that they are metrics on the set of all convex bodies of some given dimension.