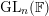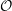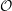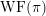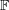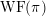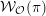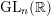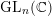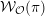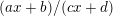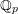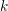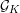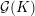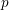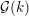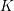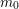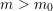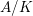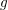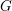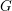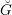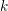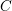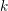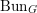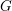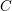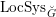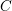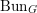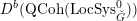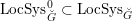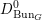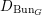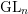We study generalized and degenerate Whittaker models for reductive groups over local fields of characteristic zero (archimedean or non-archimedean). Our main result is the construction of epimorphisms from the generalized Whittaker model corresponding to a nilpotent orbit to any degenerate Whittaker model corresponding to the same orbit, and to certain degenerate Whittaker models corresponding to bigger orbits. We also give choice-free definitions of generalized and degenerate Whittaker models. Finally, we explain how our methods imply analogous results for Whittaker–Fourier coefficients of automorphic representations. For 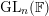 \text{GL}_{n}(\mathbb{F}) this implies that a smooth admissible representation
\text{GL}_{n}(\mathbb{F}) this implies that a smooth admissible representation  \unicode[STIX]{x1D70B} has a generalized Whittaker model
\unicode[STIX]{x1D70B} has a generalized Whittaker model  {\mathcal{W}}_{{\mathcal{O}}}(\unicode[STIX]{x1D70B}) corresponding to a nilpotent coadjoint orbit
{\mathcal{W}}_{{\mathcal{O}}}(\unicode[STIX]{x1D70B}) corresponding to a nilpotent coadjoint orbit 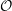 {\mathcal{O}} if and only if
{\mathcal{O}} if and only if 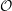 {\mathcal{O}} lies in the (closure of) the wave-front set
{\mathcal{O}} lies in the (closure of) the wave-front set 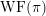 \operatorname{WF}(\unicode[STIX]{x1D70B}). Previously this was only known to hold for
\operatorname{WF}(\unicode[STIX]{x1D70B}). Previously this was only known to hold for 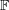 \mathbb{F} non-archimedean and
\mathbb{F} non-archimedean and  {\mathcal{O}} maximal in
{\mathcal{O}} maximal in 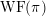 \operatorname{WF}(\unicode[STIX]{x1D70B}), see Moeglin and Waldspurger [Modeles de Whittaker degeneres pour des groupes p-adiques, Math. Z. 196 (1987), 427–452]. We also express
\operatorname{WF}(\unicode[STIX]{x1D70B}), see Moeglin and Waldspurger [Modeles de Whittaker degeneres pour des groupes p-adiques, Math. Z. 196 (1987), 427–452]. We also express 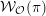 {\mathcal{W}}_{{\mathcal{O}}}(\unicode[STIX]{x1D70B}) as an iteration of a version of the Bernstein–Zelevinsky derivatives [Bernstein and Zelevinsky, Induced representations of reductive p-adic groups. I., Ann. Sci. Éc. Norm. Supér. (4) 10 (1977), 441–472; Aizenbud et al., Derivatives for representations of
{\mathcal{W}}_{{\mathcal{O}}}(\unicode[STIX]{x1D70B}) as an iteration of a version of the Bernstein–Zelevinsky derivatives [Bernstein and Zelevinsky, Induced representations of reductive p-adic groups. I., Ann. Sci. Éc. Norm. Supér. (4) 10 (1977), 441–472; Aizenbud et al., Derivatives for representations of \text{GL}(n,\mathbb{R})and
\text{GL}(n,\mathbb{R})and \text{GL}(n,\mathbb{C}), Israel J. Math. 206 (2015), 1–38]. This enables us to extend to
\text{GL}(n,\mathbb{C}), Israel J. Math. 206 (2015), 1–38]. This enables us to extend to 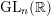 \text{GL}_{n}(\mathbb{R}) and
\text{GL}_{n}(\mathbb{R}) and 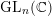 \text{GL}_{n}(\mathbb{C}) several further results by Moeglin and Waldspurger on the dimension of
\text{GL}_{n}(\mathbb{C}) several further results by Moeglin and Waldspurger on the dimension of 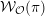 {\mathcal{W}}_{{\mathcal{O}}}(\unicode[STIX]{x1D70B}) and on the exactness of the generalized Whittaker functor.
{\mathcal{W}}_{{\mathcal{O}}}(\unicode[STIX]{x1D70B}) and on the exactness of the generalized Whittaker functor.