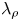Most cited
This page lists all time most cited articles for this title. Please use the publication date filters on the left if you would like to restrict this list to recently published content, for example to articles published in the last three years. The number of times each article was cited is displayed to the right of its title and can be clicked to access a list of all titles this article has been cited by.
- Cited by 2
Axisymmetric expansion of a gas from a nozzle into a vacuum
-
- Published online by Cambridge University Press:
- 29 March 2006, pp. 107-113
-
- Article
- Export citation
- Cited by 2
Transverse dam-break waves
-
- Published online by Cambridge University Press:
- 07 October 2014, R2
-
- Article
- Export citation
- Cited by 2
Hypersonic viscous interaction with strong blowing
-
- Published online by Cambridge University Press:
- 26 April 2006, pp. 291-311
-
- Article
- Export citation
- Cited by 2
Viscous irrotational analysis of the deformation and break-up time of a bubble or drop in uniaxial straining flow*
-
- Published online by Cambridge University Press:
- 09 November 2011, pp. 390-421
-
- Article
- Export citation
- Cited by 2
The pressure distribution on dihedral wings at supersonic speed
-
- Published online by Cambridge University Press:
- 28 March 2006, pp. 302-312
-
- Article
- Export citation
- Cited by 2
Laminar and transitional flow of drilling muds and various suspensions in circular tubes
-
- Published online by Cambridge University Press:
- 28 March 2006, pp. 449-464
-
- Article
- Export citation
- Cited by 2
Taylor dispersion in arbitrarily shaped axisymmetric channels
- Part of:
-
- Published online by Cambridge University Press:
- 12 December 2023, A30
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Global stability analysis of elastic aircraft in edge-of-the-envelope flow
-
- Published online by Cambridge University Press:
- 12 July 2023, A4
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Thermoelectrohydrodynamic convection in a finite cylindrical annulus under microgravity
-
- Published online by Cambridge University Press:
- 20 August 2024, A7
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Mixing in Inland and Coastal Waters. By HUGO B. FISCHER, E. JOHN LIST, ROBERT C. Y. KOH, JORO IMBEROER and NORMAN H. BROOKS. Academic Press, 1979. 483 pp. $35.00.
-
- Published online by Cambridge University Press:
- 20 April 2006, pp. 532-533
-
- Article
- Export citation
- Cited by 2
Görtler-number-based scaling of boundary-layer transition on rotating cones in axial inflow
-
- Published online by Cambridge University Press:
- 16 May 2024, R3
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Vorticity dynamics in transcritical liquid jet breakup
-
- Published online by Cambridge University Press:
- 27 December 2023, A6
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
A study of turbulent flow over patchy roughness
-
- Published online by Cambridge University Press:
- 11 March 2025, A28
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Direct calculation of the eddy viscosity operator in turbulent channel flow at Reτ = 180
-
- Published online by Cambridge University Press:
- 31 October 2024, A33
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Extension of Kelvin’s minimumenergy theorem to incompressiblefluid domains with open regions
-
- Published online by Cambridge University Press:
- 20 July 2017, pp. 208-212
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Excitation and evolution of radiating modes in supersonic boundary layers. Part 1. Fundamental resonance with impinging sound waves
-
- Published online by Cambridge University Press:
- 18 April 2024, A13
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Influence of three-dimensionality on wake synchronisation of an oscillatory cylinder
-
- Published online by Cambridge University Press:
- 11 December 2024, A24
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Nonlinear dynamics of a buoyant pendulum submerged under surface gravity waves
-
- Published online by Cambridge University Press:
- 21 April 2025, A45
-
- Article
- Export citation
- Cited by 2
New scaling laws for turbulent Poiseuille flow with wall transpiration – ERRATUM
-
- Published online by Cambridge University Press:
- 30 June 2014, p. 746
-
- Article
-
- You have access
- Export citation
- Cited by 2
Capillary lubrication of a spherical particle near a fluid interface
-
- Published online by Cambridge University Press:
- 19 December 2024, A58
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
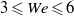 , predictions from viscous potential flow shows good agreement with the results from the Navier–Stokes equations for the bubble break-up time, whereas for larger
, predictions from viscous potential flow shows good agreement with the results from the Navier–Stokes equations for the bubble break-up time, whereas for larger  , the former underpredicts the results given by the latter. When viscosity is included, larger break-up times are predicted with respect to the inviscid case for the same
, the former underpredicts the results given by the latter. When viscosity is included, larger break-up times are predicted with respect to the inviscid case for the same  . For the drop, and considering moderate Reynolds numbers,
. For the drop, and considering moderate Reynolds numbers, 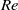 , increasing the viscous effects of the irrotational motion produces large, elongated drops that take longer to break up in comparison with results for inviscid fluids. For larger
, increasing the viscous effects of the irrotational motion produces large, elongated drops that take longer to break up in comparison with results for inviscid fluids. For larger  , it comes as a surprise that break-up times smaller than the inviscid limit are obtained. Unfortunately, results from numerical analyses of the incompressible, unsteady Navier–Stokes equations for the case of a drop have not been presented in the literature, to the best of the authors’ knowledge; hence, comparison with the viscous irrotational analysis is not possible.
, it comes as a surprise that break-up times smaller than the inviscid limit are obtained. Unfortunately, results from numerical analyses of the incompressible, unsteady Navier–Stokes equations for the case of a drop have not been presented in the literature, to the best of the authors’ knowledge; hence, comparison with the viscous irrotational analysis is not possible.