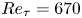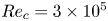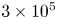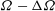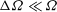Most cited
This page lists all time most cited articles for this title. Please use the publication date filters on the left if you would like to restrict this list to recently published content, for example to articles published in the last three years. The number of times each article was cited is displayed to the right of its title and can be clicked to access a list of all titles this article has been cited by.
- Cited by 2
Improved calculations of waterfalls and weir flows
-
- Published online by Cambridge University Press:
- 27 April 2022, A27
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
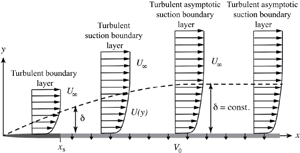
Laboratory realization of an asymptotic wall flow
-
- Published online by Cambridge University Press:
- 06 May 2021, F1
-
- Article
-
- You have access
- HTML
- Export citation
- Cited by 2
Two-way wave–vortex interactions in a Lagrangian-mean shallow water model
-
- Published online by Cambridge University Press:
- 20 December 2022, A1
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
On the non-uniqueness of the kernel of the Zakharov equation in intermediate and shallow water: the connection with the Davey–Stewartson equation
-
- Published online by Cambridge University Press:
- 02 February 2024, A32
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Immersed body motion: near-bottom added mass effects
-
- Published online by Cambridge University Press:
- 30 March 2022, A18
-
- Article
- Export citation
- Cited by 2
Effect of fluid motions on finite spheres released in turbulent boundary layers
-
- Published online by Cambridge University Press:
- 23 April 2024, A28
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Global stability analysis of elastic aircraft in edge-of-the-envelope flow
-
- Published online by Cambridge University Press:
- 12 July 2023, A4
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Intensification of non-uniformity in convergent near-conical hypersonic flow
-
- Published online by Cambridge University Press:
- 22 November 2021, A8
-
- Article
- Export citation
- Cited by 2
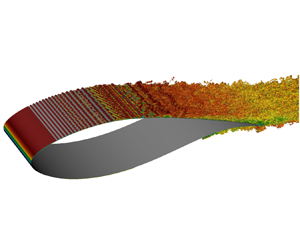
Transition to turbulence on a rotating wind turbine blade at
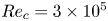 $Re_c=3 \times 10^5$
$Re_c=3 \times 10^5$
-
- Published online by Cambridge University Press:
- 14 November 2024, A54
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Numerical simulations of attachment-line boundary layer in hypersonic flow. Part 1. Roughness-induced subcritical transitions
-
- Published online by Cambridge University Press:
- 30 April 2025, A63
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
The role of polymer molecular weight distribution in drag-reducing turbulent flows
-
- Published online by Cambridge University Press:
- 14 March 2025, A10
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
On the efficacy of surface-attached air bubbles as thermal insulators for pressure-driven internal flow
-
- Published online by Cambridge University Press:
- 13 May 2024, A6
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Capillary lubrication of a spherical particle near a fluid interface
-
- Published online by Cambridge University Press:
- 19 December 2024, A58
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
New scaling laws for turbulent Poiseuille flow with wall transpiration – ERRATUM
-
- Published online by Cambridge University Press:
- 30 June 2014, p. 746
-
- Article
-
- You have access
- Export citation
- Cited by 2
Direct numerical simulations of an airfoil undergoing dynamic stall at different background disturbance levels
-
- Published online by Cambridge University Press:
- 30 April 2024, A3
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
Darcy–Forchheimer gravity currents in porous media
-
- Published online by Cambridge University Press:
- 02 December 2024, A89
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
The spin-up of a linearly stratified fluid in a sliced, circular cylinder
-
- Published online by Cambridge University Press:
- 30 September 2016, pp. 254-303
-
- Article
- Export citation
- Cited by 2
The pressure distribution on dihedral wings at supersonic speed
-
- Published online by Cambridge University Press:
- 28 March 2006, pp. 302-312
-
- Article
- Export citation
- Cited by 2
Particle capture by expanding droplets: effects of inner diffusion
-
- Published online by Cambridge University Press:
- 13 September 2022, A36
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 2
New insights into head-on bouncing of unequal-size droplets on a wetting surface
-
- Published online by Cambridge University Press:
- 18 March 2024, A25
-
- Article
-
- You have access
- Open access
- HTML
- Export citation