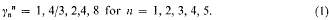Let Z(Γ) be the integral groupring of a finite Abelian group Γ. There is some interest in the study of its class group (Picard group) C(Z(Γ)) (cf. e.g. [1] and [5]). One knows that this group is mapped surjectively onto the class group of the maximal order in the rational groupring Q(Γ). Now is known in the sense that it is the product of the classgroups of the algebraic integer rings, whose quotient fields appear in the decomposition of Q(Γ). One is thus also interested in the kernel D(Z(Γ)) of the map , and it is this which concerns us here. I shall show that it can become very big.

In general the terminology and notation of [1] is used throughout. A correspondence for topological spaces is a triple f: P → Q where P and Q are topological spaces and f is a subset of P × Q, the graph of f: P → Q. A correspondence f: P → Q will be called graph-compact, or graph-closed, or graph-Souslin, or graph-analytic if f is, respectively, compact or closed or Souslin or analytic in P × Q.

Let G be a plane domain with ∞ ∊ G. Let E be the compact complement of G and cap E the logarithmic capacity. We shall assume that cap E = 1 and E ⊂ {|Z| ≤ R. Then R ≥ 1, with equality if and only if E is a closed disc.

An old theorem of Pólya and Carlson [2] states that, if the power series has rational integer coefficients, positive radius of convergence, and can be continued analytically to a region that contains points outside the closed unit disc, then the function that the power series represents is rational. This result has been extended in a number of ways (cf. e.g. Petersson [4]). The present note gives a new extension based on a recent theorem of Güting [3]. My thanks to Professors Henry Helson and Raphael Robinson for introducing me to this subject.

The object of this paper is to prove the following:

Theorem. Every perfect septenary quadratic form assumes its minimum value at a set of 7 points with integer co-ordinates whose determinant is 1.

This is true also, as shown by Rankin [1], with n ≤ 6 in place of 7. The proof will be shortened considerably by using the weaker result obtained in [1] for n = 7, and we shall also use the following classical results, see, e.g., [2], for Hermite's constant γn:

Artin, in 1927, conjectured that for any given non-zero integer a other than — 1 or a perfect square there exist infinitely many primes for which a is a primitive root. He also conjectured that the number of primes not exceeding x, denoted by Na(x), for which a is a primitive root is given by the asymptotic formula

where A(a) is a constant depending on a.

The objectjof this paper is to extend to algebraic number fields some of the recent results proved by the large sieve method. In particular we prove generalizations of Bombieri's form of the large sieve inequality [1; Theorem 1] and of the theorems of Davenport and Halberstam [2] and Bombieri [1, 4], on the average distribution of primes in arithmetic progressions.

In this paper the response of an Euler-Bernoulli viscoelastic beam to impulsive excitation is obtained using Volterra's model for the stress-strain relationship. In order to achieve a better approximation of actual materials a series of parallel connections of one or more basic models must be used. However, the analytic solutions to most problems then become very difficult. Therefore an alternative approach is to formulate such a problem in terms of the hereditary integral as proposed by Vito Volterra [1].

One of the earliest investigations of the acoustic disturbance created in a fluid of semi-infinite extent by the movement of a piston surrounded by an infinite plane rigid baffle was undertaken in 1878 by Rayleigh [1], who studied the harmonic oscillations of a piston of arbitrary shape. In subsequent years, a number of authors have presented methods for studying the “baffled piston problem”. A large portion of the literature has been concerned with a circular piston and particular attention has been devoted to the case in which the small amplitude oscillations of the piston are harmonic. References to these earlier works are given by Chadwick and Tupholme [2]. A solution to the more general problem in which the normal velocity of a circular piston is an arbitrary function of time was derived from the corresponding time-harmonic solution by Oberhettinger [3] and obtained more directly by Miles [4, see also 5]. Kozina and Makarov [6] considered the acoustic fields produced by the time-dependent motion of an arbitrarily-shaped piston.

We present here the eigenfunction expansions of the bi-harmonic Love strain function for a cylinder whose plane faces are stress-free. The convergence for the expansions of arbitrary functions in terms of these eigenfunctions is studied. As an example, the symmetrical deformations in a cylinder whose plane faces are stress-free and on whose curved edge arbitrary radial displacement and transverse shear force are prescribed is worked out.

A hemisphere, resting on a horizontal plane, is initially at rest relative to an incompressible, inviscid, non-diffusive fluid whose density is vertically stratified. The hemisphere is then given, impulsively, a small constant horizontal velocity which is maintained thereafter. Assuming that the Froude number is small, and using the Boussinesq approximation, the equations of motion are linearised and solved using a Laplace transform. The disturbance in the fluid is analysed for large times and is found to contain a steady component of purely horizontal flow, an internal wave field and internal oscillations at the Brunt-Väisälä frequency, together with their various interactions. The effects of viscosity and diffusivity are discussed qualitatively by considering their effects on an internal wave.

We investigate the steady pressure driven flow of conducting fluid through an insulating circular pipe in the presence of a transverse applied magnetic field. It is well known that an exact solution is available, and the series expressions for the fluid velocity and induced magnetic field have often appeared in the literature, Uflyand (1960), Uhlenbusch and Fischer (1961), Gold (1962); however, no progress has been made in deriving asymptotic expansions for large Hartmann number directly from these series. Boundary layer methods have been used by Shercliff (1953. 1962) to determine the core structure, the Hartmann layer structure, the current distribution and the flow rate; however, these analyses do not describe the tangential layers (called obscure layers by Shercliff), which are located in the vicinity of the points at which the applied magnetic field is tangential to the pipe.

The model of the complete system of triangles lying in a plane is a sixfold, whose nature of course depends on the chosen definition of the geometric variable. An earlier paper [1] concentrated on the variety associated with the Schubert triangle, although it contained brief descriptions of the corresponding models for other types. We now study the relation between these sixfolds, paying particular attention to the partial dilatation which transforms the model of ordered triangles into the Schubert variety.

Let X be a finite set of points in Ed. Then a partition of X into two non-empty subsets X1 and X2 (X1 ∪ X2 = X, X1 ∩ X2 = ∅) will be called a Radon partition if

.

The object of the present note is to prove the following theorem. †

Theorem. Let P be a convex d-polytope in d-dimensional real vector space Rd (d ≥ 2). If each d-polytope which is the intersection of two translates of P has the same number of vertices as P, then P is a direct linear sum ‡ of simplices.

Weber proves in §§114-124 of his Algebra [19] that if w is complex quadratic and ℤ[ω] is the ring of integers of the field ℚ(ω) then the absolute class field of ℚ(ω) is generated by the modular invariant j(w); he calls j(w) a class invariant. He goes on in §§125-144 to consider the values f(w) of other modular functions f(z); he shows that in certain cases the degree of the extension ℚ(ω, f(ω)) of ℚ(ω, j(ω)) is much less than that of ℚ(f(z)) over ℚ(f(z)); indeed, f(ω) is often in ℚ(ω, j(ω)), and in such circumstances Weber calls f(ω) a class invariant too. Using such results, Weber computes many class invariants—an end in itself, since the numbers are so beautiful. More recently, results of this type have been applied to determine all the complex quadratic fields with class number 1, and to prove that elliptic curves of certain families always have infinitely many rational points-see [9, 2, 5].

In an earlier paper [Shail, 1] one of the present authors considered the effect of a magnetic field on the frictional couple experienced by an axisymmetric solid insulator which rotates slowly in a bounded viscous conducting fluid, the applied magnetic field being parallel to the axis of rotation. Results for the couple in various geometrical configurations were obtained in the form of power series expansions in the Hartmann number M, and hence are valid only for M ≪ 1. However, because of the increased rigidity given to the fluid by the applied field, it is physically evident that the magnetic field will have a more dramatic effect on the flow pattern for large values of M. Thus one object of this paper is to investigate the frictional couple on a solid insulator rotating in an unbounded fluid for values of M ≫ 1.

In this note we shall show that the assumptions made by Murray [1] on the initial state of the gas and the initial magnetic field are inconsistent with the steady state momentum equation and the energy balance equation (20). The form of second term in equation (20) requires

Irrotational gravity waves of finite height: a numerical study. The sentence on page 146 in which equation (30) occurs should read:

The method consists of expanding the equation as

An analogue of a problem of Littlewood. The arguments of this paper are not wholly correct; a detailed corrigendum will be published in due course.