In 1973, Montgomery [12] introduced, in order to study the vertical distribution of the zeros of the Riemann zeta function, the pair correlation function
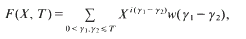
where w(u) = 4/(4 + u2) and γjj = 1, 2, run over the imaginary part of the nontrivial zeros of ζ(s). It is easy to see that, for T → ∞,
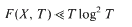
uniformly in X, and Montgomery [12], see also Goldston-Montgomery [7], proved that under the Riemann Hypothesis (RH)
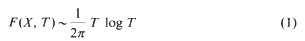
uniformly for X ≤ T ≤ XA, for any fixed A > 1. He also conjectured, under RH, that (1) holds uniformly for Xε ≤ T ≤ X, for every fixed ε > 0. We denote by MC the above conjecture.