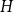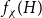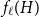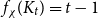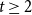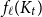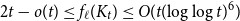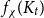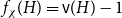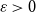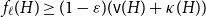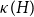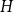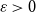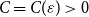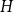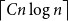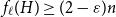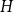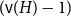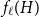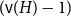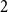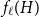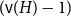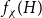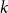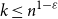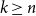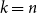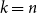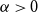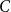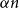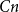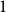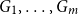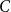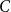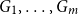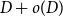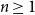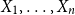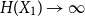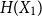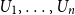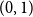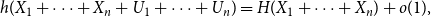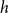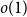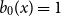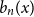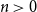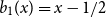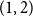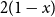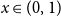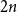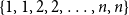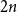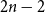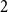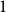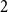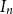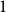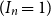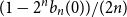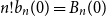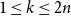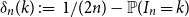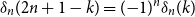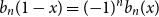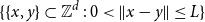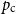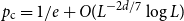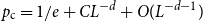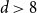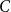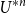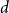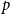Given a graph H$H$, let us denote by f_\chi (H)$f_\chi (H)$ and f_\ell (H)$f_\ell (H)$, respectively, the maximum chromatic number and the maximum list chromatic number of H$H$-minor-free graphs. Hadwiger’s famous colouring conjecture from 1943 states that f_\chi (K_t)=t-1$f_\chi (K_t)=t-1$ for every t \ge 2$t \ge 2$. A closely related problem that has received significant attention in the past concerns f_\ell (K_t)$f_\ell (K_t)$, for which it is known that 2t-o(t) \le f_\ell (K_t) \le O(t (\!\log \log t)^6)$2t-o(t) \le f_\ell (K_t) \le O(t (\!\log \log t)^6)$. Thus, f_\ell (K_t)$f_\ell (K_t)$ is bounded away from the conjectured value t-1$t-1$ for f_\chi (K_t)$f_\chi (K_t)$ by at least a constant factor. The so-called H$H$-Hadwiger’s conjecture, proposed by Seymour, asks to prove that f_\chi (H)={\textrm{v}}(H)-1$f_\chi (H)={\textrm{v}}(H)-1$ for a given graph H$H$ (which would be implied by Hadwiger’s conjecture).

In this paper, we prove several new lower bounds on f_\ell (H)$f_\ell (H)$, thus exploring the limits of a list colouring extension of H$H$-Hadwiger’s conjecture. Our main results are:

• For every \varepsilon \gt 0$\varepsilon \gt 0$ and all sufficiently large graphs H$H$ we have f_\ell (H)\ge (1-\varepsilon )({\textrm{v}}(H)+\kappa (H))$f_\ell (H)\ge (1-\varepsilon )({\textrm{v}}(H)+\kappa (H))$, where \kappa (H)$\kappa (H)$ denotes the vertex-connectivity of H$H$.

• For every \varepsilon \gt 0$\varepsilon \gt 0$ there exists C=C(\varepsilon )\gt 0$C=C(\varepsilon )\gt 0$ such that asymptotically almost every n$n$-vertex graph H$H$ with \left \lceil C n\log n\right \rceil$\left \lceil C n\log n\right \rceil$ edges satisfies f_\ell (H)\ge (2-\varepsilon )n$f_\ell (H)\ge (2-\varepsilon )n$.

The first result generalizes recent results on complete and complete bipartite graphs and shows that the list chromatic number of H$H$-minor-free graphs is separated from the desired value of ({\textrm{v}}(H)-1)$({\textrm{v}}(H)-1)$ by a constant factor for all large graphs H$H$ of linear connectivity. The second result tells us that for almost all graphs H$H$ with superlogarithmic average degree f_\ell (H)$f_\ell (H)$ is separated from ({\textrm{v}}(H)-1)$({\textrm{v}}(H)-1)$ by a constant factor arbitrarily close to 2$2$. Conceptually these results indicate that the graphs H$H$ for which f_\ell (H)$f_\ell (H)$ is close to the conjectured value ({\textrm{v}}(H)-1)$({\textrm{v}}(H)-1)$ for f_\chi (H)$f_\chi (H)$ are typically rather sparse.

Since the 1960s Mastermind has been studied for the combinatorial and information-theoretical interest the game has to offer. Many results have been discovered starting with Erdős and Rényi determining the optimal number of queries needed for two colours. For k$k$ colours and n$n$ positions, Chvátal found asymptotically optimal bounds when k \le n^{1-\varepsilon }$k \le n^{1-\varepsilon }$. Following a sequence of gradual improvements for k\geq n$k\geq n$ colours, the central open question is to resolve the gap between \Omega (n)$\Omega (n)$ and \mathcal{O}(n\log \log n)$\mathcal{O}(n\log \log n)$ for k=n$k=n$. In this paper, we resolve this gap by presenting the first algorithm for solving k=n$k=n$ Mastermind with a linear number of queries. As a consequence, we are able to determine the query complexity of Mastermind for any parameters k$k$ and n$n$.

In 2003, Bohman, Frieze, and Martin initiated the study of randomly perturbed graphs and digraphs. For digraphs, they showed that for every \alpha \gt 0$\alpha \gt 0$, there exists a constant C$C$ such that for every n$n$-vertex digraph of minimum semi-degree at least \alpha n$\alpha n$, if one adds Cn$Cn$ random edges then asymptotically almost surely the resulting digraph contains a consistently oriented Hamilton cycle. We generalize their result, showing that the hypothesis of this theorem actually asymptotically almost surely ensures the existence of every orientation of a cycle of every possible length, simultaneously. Moreover, we prove that we can relax the minimum semi-degree condition to a minimum total degree condition when considering orientations of a cycle that do not contain a large number of vertices of indegree 1$1$. Our proofs make use of a variant of an absorbing method of Montgomery.

A collection of graphs is nearly disjoint if every pair of them intersects in at most one vertex. We prove that if G_1, \dots, G_m$G_1, \dots, G_m$ are nearly disjoint graphs of maximum degree at most D$D$, then the following holds. For every fixed C$C$, if each vertex v \in \bigcup _{i=1}^m V(G_i)$v \in \bigcup _{i=1}^m V(G_i)$ is contained in at most C$C$ of the graphs G_1, \dots, G_m$G_1, \dots, G_m$, then the (list) chromatic number of \bigcup _{i=1}^m G_i$\bigcup _{i=1}^m G_i$ is at most D + o(D)$D + o(D)$. This result confirms a special case of a conjecture of Vu and generalizes Kahn’s bound on the list chromatic index of linear uniform hypergraphs of bounded maximum degree. In fact, this result holds for the correspondence (or DP) chromatic number and thus implies a recent result of Molloy and Postle, and we derive this result from a more general list colouring result in the setting of ‘colour degrees’ that also implies a result of Reed and Sudakov.

It is proven that a conjecture of Tao (2010) holds true for log-concave random variables on the integers: For every n \geq 1$n \geq 1$, if X_1,\ldots,X_n$X_1,\ldots,X_n$ are i.i.d. integer-valued, log-concave random variables, then

\begin{equation*} H(X_1+\cdots +X_{n+1}) \geq H(X_1+\cdots +X_{n}) + \frac {1}{2}\log {\Bigl (\frac {n+1}{n}\Bigr )} - o(1) \end{equation*} $$\begin{equation*} H(X_1+\cdots +X_{n+1}) \geq H(X_1+\cdots +X_{n}) + \frac {1}{2}\log {\Bigl (\frac {n+1}{n}\Bigr )} - o(1) \end{equation*}$$

H(X_1) \to \infty$H(X_1) \to \infty$

H(X_1)$H(X_1)$

U_1,\ldots,U_n$U_1,\ldots,U_n$

(0,1)$(0,1)$

\begin{equation*} h(X_1+\cdots +X_n + U_1+\cdots +U_n) = H(X_1+\cdots +X_n) + o(1), \end{equation*} $$\begin{equation*} h(X_1+\cdots +X_n + U_1+\cdots +U_n) = H(X_1+\cdots +X_n) + o(1), \end{equation*}$$

H(X_1) \to \infty$H(X_1) \to \infty$

h$h$

o(1)$o(1)$

The factorially normalized Bernoulli polynomials b_n(x) = B_n(x)/n!$b_n(x) = B_n(x)/n!$ are known to be characterized by 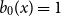b_0(x) = 1$b_0(x) = 1$ and 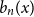b_n(x)$b_n(x)$ for 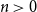n \gt 0$n \gt 0$ is the anti-derivative of b_{n-1}(x)$b_{n-1}(x)$ subject to \int _0^1 b_n(x) dx = 0$\int _0^1 b_n(x) dx = 0$. We offer a related characterization: 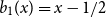b_1(x) = x - 1/2$b_1(x) = x - 1/2$ and ({-}1)^{n-1} b_n(x)$({-}1)^{n-1} b_n(x)$ for n \gt 0$n \gt 0$ is the n$n$-fold circular convolution of b_1(x)$b_1(x)$ with itself. Equivalently, 1 - 2^n b_n(x)$1 - 2^n b_n(x)$ is the probability density at x \in (0,1)$x \in (0,1)$ of the fractional part of a sum of n$n$ independent random variables, each with the beta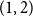(1,2)$(1,2)$ probability density 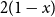2(1-x)$2(1-x)$ at 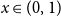x \in (0,1)$x \in (0,1)$. This result has a novel combinatorial analog, the Bernoulli clock: mark the hours of a 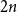2 n$2 n$ hour clock by a uniformly random permutation of the multiset 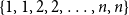\{1,1, 2,2, \ldots, n,n\}$\{1,1, 2,2, \ldots, n,n\}$, meaning pick two different hours uniformly at random from the 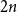2 n$2 n$ hours and mark them 1$1$, then pick two different hours uniformly at random from the remaining 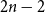2 n - 2$2 n - 2$ hours and mark them 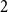2$2$, and so on. Starting from hour 0 = 2n$0 = 2n$, move clockwise to the first hour marked 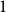1$1$, continue clockwise to the first hour marked 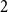2$2$, and so on, continuing clockwise around the Bernoulli clock until the first of the two hours marked n$n$ is encountered, at a random hour 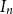I_n$I_n$ between 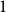1$1$ and 2n$2n$. We show that for each positive integer n$n$, the event 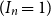( I_n = 1)$( I_n = 1)$ has probability 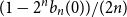(1 - 2^n b_n(0))/(2n)$(1 - 2^n b_n(0))/(2n)$, where 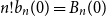n! b_n(0) = B_n(0)$n! b_n(0) = B_n(0)$ is the n$n$th Bernoulli number. For 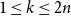1 \le k \le 2 n$1 \le k \le 2 n$, the difference 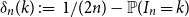\delta _n(k)\,:\!=\, 1/(2n) -{\mathbb{P}}( I_n = k)$\delta _n(k)\,:\!=\, 1/(2n) -{\mathbb{P}}( I_n = k)$ is a polynomial function of k$k$ with the surprising symmetry 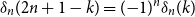\delta _n( 2 n + 1 - k) = ({-}1)^n \delta _n(k)$\delta _n( 2 n + 1 - k) = ({-}1)^n \delta _n(k)$, which is a combinatorial analog of the well-known symmetry of Bernoulli polynomials 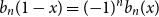b_n(1-x) = ({-}1)^n b_n(x)$b_n(1-x) = ({-}1)^n b_n(x)$.

A spread-out lattice animal is a finite connected set of edges in \{\{x,y\}\subset \mathbb{Z}^d\;:\;0\lt \|x-y\|\le L\}$\{\{x,y\}\subset \mathbb{Z}^d\;:\;0\lt \|x-y\|\le L\}$. A lattice tree is a lattice animal with no loops. The best estimate on the critical point p_{\textrm{c}}$p_{\textrm{c}}$ so far was achieved by Penrose (J. Stat. Phys. 77, 3–15, 1994) : p_{\textrm{c}}=1/e+O(L^{-2d/7}\log L)$p_{\textrm{c}}=1/e+O(L^{-2d/7}\log L)$ for both models for all d\ge 1$d\ge 1$. In this paper, we show that p_{\textrm{c}}=1/e+CL^{-d}+O(L^{-d-1})$p_{\textrm{c}}=1/e+CL^{-d}+O(L^{-d-1})$ for all d\gt 8$d\gt 8$, where the model-dependent constant C$C$ has the random-walk representation

\begin{align*} C_{\textrm{LT}}=\sum _{n=2}^\infty \frac{n+1}{2e}U^{*n}(o),&& C_{\textrm{LA}}=C_{\textrm{LT}}-\frac 1{2e^2}\sum _{n=3}^\infty U^{*n}(o), \end{align*} $$\begin{align*} C_{\textrm{LT}}=\sum _{n=2}^\infty \frac{n+1}{2e}U^{*n}(o),&& C_{\textrm{LA}}=C_{\textrm{LT}}-\frac 1{2e^2}\sum _{n=3}^\infty U^{*n}(o), \end{align*}$$

U^{*n}$U^{*n}$

n$n$

d$d$

\{x\in{\mathbb R}^d\;: \|x\|\le 1\}$\{x\in{\mathbb R}^d\;: \|x\|\le 1\}$

p$p$