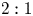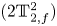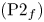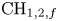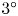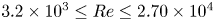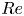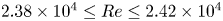130 results
18 - The Kennedy Paradox: Games of Conflict and Escalation
- from Part IV - Society
-
- Book:
- Behavioral Network Science
- Published online:
- 08 November 2024
- Print publication:
- 19 December 2024, pp 296-319
-
- Chapter
- Export citation
Large-eddy simulation-based shape optimization for mitigating turbulent wakes of a bluff body using the regularized ensemble Kalman method
-
- Journal:
- Journal of Fluid Mechanics / Volume 1001 / 25 December 2024
- Published online by Cambridge University Press:
- 12 December 2024, A31
-
- Article
-
- You have access
- HTML
- Export citation
Representing turbulent statistics with partitions of state space. Part 1. Theory and methodology
-
- Journal:
- Journal of Fluid Mechanics / Volume 997 / 25 October 2024
- Published online by Cambridge University Press:
- 21 October 2024, A1
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Relative fluid stretching and rotation for sparse trajectory observations
-
- Journal:
- Journal of Fluid Mechanics / Volume 996 / 10 October 2024
- Published online by Cambridge University Press:
- 03 October 2024, A40
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Fake turbulence
-
- Journal:
- Journal of Fluid Mechanics / Volume 990 / 10 July 2024
- Published online by Cambridge University Press:
- 12 August 2024, R2
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Bacterial barriers
-
- Journal:
- Journal of Fluid Mechanics / Volume 988 / 10 June 2024
- Published online by Cambridge University Press:
- 25 July 2024, F1
-
- Article
-
- You have access
- HTML
- Export citation
Enhancing transport barriers with swimming micro-organisms in chaotic flows
-
- Journal:
- Journal of Fluid Mechanics / Volume 988 / 10 June 2024
- Published online by Cambridge University Press:
- 31 May 2024, A25
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
15 - Modelling Weather and Climate
- from Part IV - The Climate System
-
- Book:
- The Science of Our Changing Climate
- Published online:
- 05 April 2024
- Print publication:
- 02 May 2024, pp 251-270
-
- Chapter
- Export citation
Large-scale patterns set the predictability limit of extreme events in Kolmogorov flow
-
- Journal:
- Journal of Fluid Mechanics / Volume 986 / 10 May 2024
- Published online by Cambridge University Press:
- 30 April 2024, A2
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Chaos via type-II intermittency in a forced globally unstable jet
-
- Journal:
- Journal of Fluid Mechanics / Volume 984 / 10 April 2024
- Published online by Cambridge University Press:
- 04 April 2024, R8
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
The conditional Lyapunov exponents and synchronisation of rotating turbulent flows
-
- Journal:
- Journal of Fluid Mechanics / Volume 983 / 25 March 2024
- Published online by Cambridge University Press:
- 12 March 2024, A1
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Forced synchronization of self-excited chaotic thermoacoustic oscillations
-
- Journal:
- Journal of Fluid Mechanics / Volume 982 / 10 March 2024
- Published online by Cambridge University Press:
- 01 March 2024, A9
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
C - Strategist Should Find Advantage
-
- Book:
- Three Faces of Sun Tzu
- Published online:
- 07 March 2024
- Print publication:
- 15 February 2024, pp 171-234
-
- Chapter
- Export citation
C - Strategist Should Find Advantage
-
- Book:
- Three Faces of Sun Tzu
- Published online:
- 07 March 2024
- Print publication:
- 15 February 2024, pp 171-234
-
- Chapter
- Export citation
The production of uncertainty in three-dimensional Navier–Stokes turbulence
- Part of
-
- Journal:
- Journal of Fluid Mechanics / Volume 977 / 25 December 2023
- Published online by Cambridge University Press:
- 13 December 2023, A17
-
- Article
-
- You have access
- HTML
- Export citation
13 - Orchestrating Chaos
- from Part IV - Literary Genres
-
-
- Book:
- Jazz and American Culture
- Published online:
- 09 November 2023
- Print publication:
- 30 November 2023, pp 203-218
-
- Chapter
- Export citation
Predicting turbulent dynamics with the convolutional autoencoder echo state network
-
- Journal:
- Journal of Fluid Mechanics / Volume 975 / 25 November 2023
- Published online by Cambridge University Press:
- 15 November 2023, A2
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
On the emergence of secondary tones in airfoil noise
-
- Journal:
- Journal of Fluid Mechanics / Volume 966 / 10 July 2023
- Published online by Cambridge University Press:
- 27 June 2023, A8
-
- Article
- Export citation
Dynamics of iteration operators on self-maps of locally compact Hausdorff spaces
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 44 / Issue 3 / March 2024
- Published online by Cambridge University Press:
- 03 May 2023, pp. 749-768
- Print publication:
- March 2024
-
- Article
-
- You have access
- HTML
- Export citation