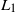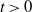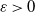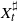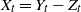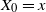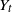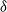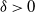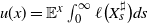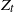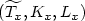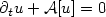25 results
On stochastic control under poissonian intervention: optimality of a barrier strategy in a general Lévy model
- Part of
-
- Journal:
- Journal of Applied Probability , First View
- Published online by Cambridge University Press:
- 09 January 2025, pp. 1-24
-
- Article
- Export citation
The impact of pinning points on memorylessness in Lévy random bridges
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 62 / Issue 1 / March 2025
- Published online by Cambridge University Press:
- 15 October 2024, pp. 172-187
- Print publication:
- March 2025
-
- Article
-
- You have access
- HTML
- Export citation
Generalised shot-noise representations of stochastic systems driven by non-Gaussian Lévy processes
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 56 / Issue 4 / December 2024
- Published online by Cambridge University Press:
- 21 March 2024, pp. 1215-1250
- Print publication:
- December 2024
-
- Article
-
- You have access
- HTML
- Export citation
Predicting the last zero before an exponential time of a spectrally negative Lévy process
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 55 / Issue 2 / June 2023
- Published online by Cambridge University Press:
- 16 January 2023, pp. 611-642
- Print publication:
- June 2023
-
- Article
- Export citation
Non-asymptotic control of the cumulative distribution function of Lévy processes
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 54 / Issue 3 / September 2022
- Published online by Cambridge University Press:
- 15 June 2022, pp. 913-944
- Print publication:
- September 2022
-
- Article
- Export citation
Probability of total domination for transient reflecting processes in a quadrant
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 54 / Issue 4 / December 2022
- Published online by Cambridge University Press:
- 14 June 2022, pp. 1094-1138
- Print publication:
- December 2022
-
- Article
- Export citation
Subexponential potential asymptotics with applications
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 54 / Issue 3 / September 2022
- Published online by Cambridge University Press:
- 13 June 2022, pp. 783-807
- Print publication:
- September 2022
-
- Article
- Export citation
Unified signature cumulants and generalized Magnus expansions
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 10 / 2022
- Published online by Cambridge University Press:
- 09 June 2022, e42
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
On operator fractional Lévy motion: integral representations and time-reversibility
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 54 / Issue 2 / June 2022
- Published online by Cambridge University Press:
- 06 June 2022, pp. 493-535
- Print publication:
- June 2022
-
- Article
- Export citation
Valuing vulnerable Asian options with liquidity risk under Lévy processes
-
- Journal:
- Probability in the Engineering and Informational Sciences / Volume 37 / Issue 3 / July 2023
- Published online by Cambridge University Press:
- 07 February 2022, pp. 653-673
-
- Article
- Export citation
Non-Gaussian fluctuations of randomly trapped random walks
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 53 / Issue 3 / September 2021
- Published online by Cambridge University Press:
- 08 October 2021, pp. 801-838
- Print publication:
- September 2021
-
- Article
- Export citation
Double hypergeometric Lévy processes and self-similarity
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 58 / Issue 1 / March 2021
- Published online by Cambridge University Press:
- 25 February 2021, pp. 254-273
- Print publication:
- March 2021
-
- Article
- Export citation
A note on the optimal dividends paid in a foreign currency
-
- Journal:
- Annals of Actuarial Science / Volume 11 / Issue 1 / March 2017
- Published online by Cambridge University Press:
- 10 November 2016, pp. 67-73
-
- Article
- Export citation
Simulation and approximationof Lévy-driven stochastic differential equations
-
- Journal:
- ESAIM: Probability and Statistics / Volume 15 / 2011
- Published online by Cambridge University Press:
- 05 January 2012, pp. 233-248
- Print publication:
- 2011
-
- Article
- Export citation
Asymptotic behavior of the hitting time, overshootandundershoot for some Lévy processes
-
- Journal:
- ESAIM: Probability and Statistics / Volume 12 / 2008
- Published online by Cambridge University Press:
- 13 November 2007, pp. 58-93
- Print publication:
- 2008
-
- Article
- Export citation
Convex rearrangements of Lévy processes
-
- Journal:
- ESAIM: Probability and Statistics / Volume 11 / June 2007
- Published online by Cambridge University Press:
- 31 March 2007, pp. 161-172
- Print publication:
- June 2007
-
- Article
- Export citation
Convergence to infinitely divisible distributions with finite variance for someweakly dependent sequences
-
- Journal:
- ESAIM: Probability and Statistics / Volume 9 / February 2005
- Published online by Cambridge University Press:
- 15 November 2005, pp. 38-73
- Print publication:
- February 2005
-
- Article
- Export citation
Fast deterministic pricing of optionson Lévy driven assets
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 38 / Issue 1 / January 2004
- Published online by Cambridge University Press:
- 15 February 2004, pp. 37-71
- Print publication:
- January 2004
-
- Article
- Export citation
Periodic Ornstein-Uhlenbeck processes driven by Lévy processes
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 39 / Issue 4 / December 2002
- Published online by Cambridge University Press:
- 14 July 2016, pp. 748-763
- Print publication:
- December 2002
-
- Article
- Export citation