We derive analytically the angular velocity of a spheroid, of an arbitrary aspect ratio $\kappa$, sedimenting in a linearly stratified fluid. The analysis demarcates regions in parameter space corresponding to broadside-on and edgewise settling in the limit $Re, Ri_v \ll 1$, where $Re = \rho _0UL/\mu$ and $Ri_v =\gamma L^3\,g/\mu U$, the Reynolds and viscous Richardson numbers, respectively, are dimensionless measures of the importance of inertial and buoyancy forces relative to viscous ones. Here, $L$ is the spheroid semi-major axis, $U$ an appropriate settling velocity scale, $\mu$ the fluid viscosity and $\gamma \ (>0)$ the (constant) density gradient characterizing the stably stratified ambient, with the fluid density $\rho_0$ taken to be a constant within the Boussinesq framework. A reciprocal theorem formulation identifies three contributions to the angular velocity: (1) an $O(Re)$ inertial contribution that already exists in a homogeneous ambient, and orients the spheroid broadside-on; (2) an $O(Ri_v)$ hydrostatic contribution due to the ambient stratification that also orients the spheroid broadside-on; and (3) a hydrodynamic contribution arising from the perturbation of the ambient stratification whose nature depends on $Pe$; $Pe = UL/D$ being the Péclet number with $D$ the diffusivity of the stratifying agent. For $Pe \ll 1$, this contribution is $O(Ri_v)$ and orients prolate spheroids edgewise for all $\kappa \ (>1)$. For oblate spheroids, it changes sign across a critical aspect ratio $\kappa _c \approx 0.41$, orienting oblate spheroids with $\kappa _c < \kappa < 1$ edgewise and those with $\kappa < \kappa _c$ broadside-on. For $Pe \ll 1$, the hydrodynamic component is always smaller in magnitude than the hydrostatic one, so a sedimenting spheroid in this limit always orients broadside-on. For $Pe \gg 1$, the hydrodynamic contribution is dominant, being $O(Ri_v^{{2}/{3}}$) in the Stokes stratification regime characterized by $Re \ll Ri_v^{{1}/{3}}$, and orients the spheroid edgewise regardless of $\kappa$. Consideration of the inertial and large-$Pe$ stratification-induced angular velocities leads to two critical curves which separate the broadside-on and edgewise settling regimes in the $Ri_v/Re^{{3}/{2}}$–$\kappa$ plane, with the region between the curves corresponding to stable intermediate equilibrium orientations. The predictions for large $Pe$ are broadly consistent with observations.

Recent developments in neural networks have shown the potential of estimating drag on irregular rough surfaces. Nevertheless, the difficulty of obtaining a large high-fidelity dataset to train neural networks is deterring their use in practical applications. In this study, we propose a transfer learning framework to model the drag on irregular rough surfaces even with a limited amount of direct numerical simulations. We show that transfer learning of empirical correlations, reported in the literature, can significantly improve the performance of neural networks for drag prediction. This is because empirical correlations include ‘approximate knowledge’ of the drag dependency in high-fidelity physics. The ‘approximate knowledge’ allows neural networks to learn the surface statistics known to affect drag more efficiently. The developed framework can be applied to applications where acquiring a large dataset is difficult but empirical correlations have been reported.

This combined numerical/laboratory study investigates the effect of stratification form on the shoaling characteristics of internal solitary waves propagating over a smooth, linear topographic slope. Three stratification types are investigated, namely (i) thin tanh (homogeneous upper and lower layers separated by a thin pycnocline), (ii) surface stratification (linearly stratified layer overlaying a homogeneous lower layer) and (iii) broad tanh (continuous density gradient throughout the water column). It is found that the form of stratification affects the breaking type associated with the shoaling wave. In the thin tanh stratification, good agreement is seen with past studies. Waves over the shallowest slopes undergo fission. Over steeper slopes, the breaking type changes from surging, through collapsing to plunging with increasing wave steepness $A_w/L_w$ for a given topographic slope, where $A_w$ and $L_w$ are incident wave amplitude and wavelength, respectively. In the surface stratification regime, the breaking classification differs from the thin tanh stratification. Plunging dynamics is inhibited by the density gradient throughout the upper layer, instead collapsing-type breakers form for the equivalent location in parameter space in the thin tanh stratification. In the broad tanh profile regime, plunging dynamics is likewise inhibited and the near-bottom density gradient prevents the collapsing dynamics. Instead, all waves either fission or form surging breakers. As wave steepness in the broad tanh stratification increases, the bolus formed by surging exhibits evidence of Kelvin–Helmholtz instabilities on its upper boundary. In both two- and three-dimensional simulations, billow size grows with increasing wave steepness, dynamics not previously observed in the literature.

The dominant paradigm in turbulent wall flows is that the mean velocity near the wall, when scaled on wall variables, is independent of the friction Reynolds number 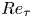$Re_\tau$. This paradigm faces challenges when applied to fluctuations but has received serious attention only recently. Here, by extending our earlier work (Chen & Sreenivasan, J. Fluid Mech., vol. 908, 2021, p. R3) we present a promising perspective, and support it with data, that fluctuations displaying non-zero wall values, or near-wall peaks, are bounded for large values of 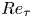$Re_\tau$, owing to the natural constraint that the dissipation rate is bounded. Specifically, 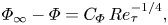$\varPhi _\infty - \varPhi = C_\varPhi \,Re_\tau ^{-1/4},$ where $\varPhi$ represents the maximum value of any of the following quantities: energy dissipation rate, turbulent diffusion, fluctuations of pressure, streamwise and spanwise velocities, squares of vorticity components, and the wall values of pressure and shear stresses; the subscript 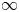$\infty$ denotes the bounded asymptotic value of 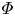$\varPhi$, and the coefficient 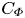$C_\varPhi$ depends on $\varPhi$ but not on 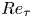$Re_\tau$. Moreover, there exists a scaling law for the maximum value in the wall-normal direction of high-order moments, of the form 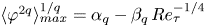$\langle \varphi ^{2q}\rangle ^{{1}/{q}}_{max}= \alpha _q-\beta _q\,Re^{-1/4}_\tau$, where $\varphi$ represents the streamwise or spanwise velocity fluctuation, and 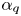$\alpha _q$ and 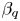$\beta _q$ are independent of $Re_\tau$. Excellent agreement with available data is observed. A stochastic process for which the random variable has the form just mentioned, referred to here as the ‘linear 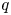$q$-norm Gaussian’, is proposed to explain the observed linear dependence of 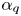$\alpha _q$ on 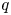$q$.

We use computational methods to determine the minimal yield stress required in order to hold static a buoyant bubble in a yield-stress liquid. The static limit is governed by the bubble shape, the dimensionless surface tension ($\gamma$) and the ratio of the yield stress to the buoyancy stress ($Y$). For a given geometry, bubbles are static for $Y > Y_c$, which we determine for a range of shapes. Given that surface tension is negligible, long prolate bubbles require larger yield stress to hold static compared with oblate bubbles. Non-zero $\gamma$ increases $Y_c$ and for large $\gamma$ the yield-capillary number ($Y/\gamma$) determines the static boundary. In this limit, although bubble shape is important, bubble orientation is not. Two-dimensional planar and axisymmetric bubbles are studied.

A multiscale reduced description of turbulent free shear flows in the presence of strong stabilizing density stratification is derived via asymptotic analysis of the Boussinesq equations in the simultaneous limits of small Froude and large Reynolds numbers. The analysis explicitly recognizes the occurrence of dynamics on disparate spatiotemporal scales, yielding simplified partial differential equations governing the coupled evolution of slow large-scale hydrostatic flows and fast small-scale isotropic instabilities and internal waves. The dynamics captured by the coupled reduced equations is illustrated in the context of two-dimensional strongly stratified Kolmogorov flow. A noteworthy feature of the reduced model is that the fluctuations are constrained to satisfy quasilinear (QL) dynamics about the comparably slowly varying large-scale fields. Crucially, this QL reduction is not invoked as an ad hoc closure approximation, but rather is derived in a physically relevant and mathematically consistent distinguished limit. Further analysis of the resulting slow–fast QL system shows how the amplitude of the fast stratified-shear instabilities is slaved to the slowly evolving mean fields to ensure the marginal stability of the latter. Physically, this marginal stability condition appears to be compatible with recent evidence of self-organized criticality in both observations and simulations of stratified turbulence. Algorithmically, the slaving of the fluctuation fields enables numerical simulations to be time-evolved strictly on the slow time scale of the hydrostatic flow. The reduced equations thus provide a solid mathematical foundation for future studies of three-dimensional strongly stratified turbulence in extreme parameter regimes of geophysical relevance and suggest avenues for new sub-grid-scale parametrizations.

The motion of an object within a viscous fluid and in the vicinity of a soft surface induces a hydrodynamic stress field that deforms the latter, thus modifying the boundary conditions of the flow. This results in elastohydrodynamic interactions experienced by the particle. Here, we derive a soft-lubrication model, in order to compute all the forces and torque applied on a rigid sphere that is free to translate and rotate near an elastic wall. We focus on the limit of small deformations of the surface with respect to the fluid-gap thickness, and perform a perturbation analysis in dimensionless compliance. The response is computed in the framework of linear elasticity, for planar elastic substrates in the limiting cases of thick and thin layers. The EHD forces are also obtained analytically using the Lorentz reciprocal theorem.

The analogy between rotating shear flow and thermal convection suggests the existence of plumes, inertial waves and plume currents in plane Poiseuille flow under spanwise rotation. The existence of these flow structures is examined with the results of three-dimensional and two-dimensional three-component direct numerical simulations. The dynamics of plumes near the unstable side is embodied in a truncated exponential distribution of turbulent fluctuations. For large rotation numbers, inertial waves are identified near the stable side, and these can be used to explain the abnormal flow statistics, such as the large root-mean-square of the streamwise velocity fluctuation and the nearly negligible Reynolds shear stress. For small or medium rotation numbers, plumes generated from the unstable side form large-scale plume currents and the patterns of the plume currents show different capabilities in scalar transport.

A blunt obstacle in the path of a rapid granular avalanche generates a bow shock (a jump in the avalanche thickness and velocity), a region of static grains upstream of the obstacle, and a grain-free region downstream. Here, it is shown that this interaction is qualitatively altered if the incline on which the avalanche is flowing is changed from smooth to rough. On a rough incline, the friction between the grains and the incline depends on the flow thickness and speed, which allows both rapid (supercritical) and slow (subcritical) steady uniform avalanches. For supercritical experimental flows, the material is diverted around a blunt obstacle by the formation of a bow shock and a static dead zone upstream of the obstacle. Downslope, a grain-free vacuum region forms, but, in contrast to flows on smooth beds, static levees form at the boundary between the vacuum region and the flow. In slower, subcritical, flows the flow is diverted smoothly around the dead zone and the obstacle without forming a bow shock. After the avalanche stops, signatures of the dead zone, levees and (in subcritical flows) a deeper region upslope of the obstacle are frozen into the deposit. To capture this behaviour, numerical simulations are performed with a depth-averaged avalanche model that includes frictional hysteresis and depth-averaged viscous terms, which are needed to accurately model the flowing and deposited regions. These results may be directly relevant to geophysical mass flows and snow avalanches, which flow over rough terrain and may impact barriers or other infrastructure.

Theoretical analysis of attachment-line instabilities is performed for supersonic swept flows using the compressible Hiemenz approximation for the mean flow and the successive approximation procedures for disturbances. The theoretical model captures the dominant attachment-line modes in wide ranges of the sweep Mach number ${M_e}$ and the wall temperature ratio. It is shown that these modes behave similar to the first and second Mack modes in the boundary layer flow. This similarity allows us to extrapolate the knowledge gained for Mack modes to the attachment-line instabilities. In particular, we find that at sufficiently large ${M_e}$, the dominant attachment-line instability is associated with the synchronisation of slow and fast modes of acoustic nature. Point-by-point comparisons of the theoretical predictions with the experiments of Gaillard et al. (Exp. Fluids, vol. 26, 1999, pp. 169–176) demonstrate that at ${M_e} > 4$, the theory captures a significant drop of the transition onset Reynolds number, which is below the contamination criterion of Poll $({R_\mathrm{\ast }} = 250)$ at ${M_e} > 6$. This contradicts the generally accepted assumption that the attachment-line flow is stable for ${R_\mathrm{\ast }} \le 250$. The theoretical critical Reynolds numbers lie well below the experimental transition-onset Reynolds numbers. Stability computations using the Navier–Stokes mean flow and accounting for the leading-edge curvature effect do not eliminate this discrepancy. Most likely, in the experiments of Gaillard et al., we face with an unknown effect that does not fit to the concept of transition arising from linear instability.

To spontaneously break their intrinsic symmetry and self-propel at the micron scale, isotropic active colloidal particles and droplets exploit the nonlinear convective transport of chemical solutes emitted/consumed at their surface by the surface-driven fluid flows generated by these solutes. Significant progress was recently made to understand the onset of self-propulsion and nonlinear dynamics. Yet, most models ignore a fundamental experimental feature, namely the spatial confinement of the colloid, and its effect on propulsion. In this work the self-propulsion of an isotropic colloid inside a capillary tube is investigated numerically. A flexible computational framework is proposed based on a finite-volume approach on adaptative octree grids and embedded boundary methods. This method is able to account for complex geometric confinement, the nonlinear coupling of chemical transport and flow fields, and the precise resolution of the surface boundary conditions, that drive the system's dynamics. Somewhat counterintuitively, spatial confinement promotes the colloid's spontaneous motion by reducing the minimum advection-to-diffusion ratio or Péclet number, ${Pe}$, required to self-propel; furthermore, self-propulsion velocities are significantly modified as the colloid-to-capillary size ratio $\kappa$ is increased, reaching a maximum at fixed ${Pe}$ for an optimal confinement $0<\kappa <1$. These properties stem from a fundamental change in the dominant chemical transport mechanism with respect to the unbounded problem: with diffusion now restricted in most directions by the confining walls, the excess solute is predominantly convected away downstream from the colloid, enhancing front-back concentration contrasts. These results are confirmed quantitatively using conservation arguments and lubrication analysis of the tightly confined limit, $\kappa \rightarrow 1$.

The asymptotic behaviour of Reynolds stresses close to walls is well established in incompressible flows owing to the constraint imposed by the solenoidal nature of the velocity field. For compressible flows, thus, one may expect a different asymptotic behaviour, which has indeed been noted in the literature. However, the transition from incompressible to compressible scaling, as well as the limiting behaviour for the latter, is largely unknown. Thus, we investigate the effects of compressibility on the near-wall, asymptotic behaviour of turbulent fluxes using a large direct numerical simulation (DNS) database of turbulent channel flow at higher than usual wall-normal resolutions. We vary the Mach number at a constant friction Reynolds number to directly assess compressibility effects. We observe that the near-wall asymptotic behaviour for compressible turbulent flow is different from the corresponding incompressible flow even if the mean density variations are taken into account and semi-local scalings are used. For Mach numbers near the incompressible regimes, the near-wall asymptotic behaviour follows the well-known theoretical behaviour. When the Mach number is increased, turbulent fluxes containing wall-normal components show a decrease in the slope owing to increased dilatation effects. We observe that $R_{vv}$ approaches its high-Mach-number asymptote at a lower Mach number than that required for the other fluxes. We also introduce a transition distance from the wall at which turbulent fluxes exhibit a change in scaling exponents. Implications for wall models are briefly presented.

Planar partial coalescence is a phenomenon in which a droplet at a free surface or interface between two fluids coalesces into the plane surface producing a smaller droplet rather than coalescing completely. This smaller, ‘daughter’ droplet will be driven towards the interface by gravity and capillary forces resulting in a cascade effect of progressively small daughter droplets until the Ohnesorge Number approaches $\sim$1 and the cascade terminates with a full coalescence event. This paper utilizes a room temperature liquid metal alloy composed of gallium, indium and tin to study partial coalescence in a viscous quiescent medium and observed bouncing of the coalescing droplets on the interface. We observed the event using high speed videography measuring effects such as the droplet to daughter droplet ratio, droplet velocities, droplet bounce heights and coefficients of restitution for the bouncing event. An existing model (Honey & Kavehpour, Phys. Rev. E, vol. 73, 2006) from our group was used, validated and expanded upon to include buoyancy effects to estimate the initial velocity of the droplet and we developed two new models for the droplet travel and maximum bounce height. The first utilizes the Stokes model for drag to moderate success while the second utilizes a model from Beard & Pruppacher (J. Atmos. Sci., vol. 26, 1969, pp. 1066–1072) and a fourth-order Runge–Kutta numerical integration scheme to predict the droplet velocity and position as functions of time. Additionally the coefficient of restitution was determined from the model using a shooting method technique in tandem with measured data to find a coefficient of restitution value of $A = 0.27 \pm 0.06$. This ‘bouncing drop’ phenomenon continues in a quiescent viscous fluid to the sub-micron scale and was facilitated by the material properties of the liquid metal including the high density, moderate viscosity and particularly high interfacial tension.

A sequence of two- and three-dimensional simulations are conducted for the double-diffusive convection (DDC) flows in the diffusive regime subjected to an imposed shear. For a wide range of control parameters, and for sufficiently strong perturbation of the conductive initial state, staircase-like structures spontaneously develop, with relatively well-mixed layers separated by sharp interfaces of enhanced scalar gradient. Such staircases appear to be robust even in the presence of strong shear over very long times, with early-time coarsening of the observed layers. For the same set of control parameters, different asymptotic layered states, with markedly different vertical scalar fluxes, can arise for different initial perturbation structures. The imposed shear significantly spatio-temporally modifies the vertical transport of the various scalars. The flux ratio $\gamma ^*$ (i.e. the ratio between the density fluxes due to the total salt flux and the total heat flux) is found, at steady state, to be essentially equal to the square root of the ratio of the salt diffusivity to the thermal diffusivity, consistent with the physical model proposed by Linden & Shirtcliffe (J. Fluid Mech., vol. 87, 1978, pp. 417–432) and the variational arguments presented by Stern (J. Fluid Mech., vol. 114, 1982, pp. 105–121) for unsheared double-diffusive convection.

Expanding recent observations by Hammond & Meng (J. Fluid Mech., vol. 921, 2021, A16), we present a range of detailed experimental data of the radial distribution function (r.d.f.) of inertial particles in isotropic turbulence for different Stokes number, $St$, showing that the r.d.f. grows explosively with decreasing separation r, exhibiting $r^{-6}$ scaling as the collision radius is approached, regardless of $St$ or particle radius $a$. To understand such explosive clustering, we correct a number of errors in the theory by Yavuz et al. (Phys. Rev. Lett., vol. 120, 2018, 244504) based on hydrodynamic interactions between pairs of small, weakly inertial particles. A comparison between the corrected theory and the experiment shows that the theory by Yavuz et al. underpredicts the r.d.f. by orders of magnitude. To explain this discrepancy, we explore several alternative mechanisms for this discrepancy that were not included in the theory and show that none of them are likely the explanation. This suggests new, yet-to-be-identified physical mechanisms are at play, requiring further investigation and new theories.

This work reports experimental observation and theoretical explanation of the dynamics and morphology of a droplet passing through a soap film. During the process, the film undergoes four sequential responses: (1) film deformation upon droplet impact; (2) drop–film detachment; (3) coalescence of the film shell with the drop; (4) peel-off of the film shell. Physical models and the corresponding analytical expressions are developed to reveal the underlying physics for the observed four responses. It is identified that the film is an elongated catenoid under continuous stretch by the droplet, and that they separate at the fixed height of 5.8 times of the droplet radius while the detach point is located at the centre of the height. After separation, the droplet is wrapped with a film shell, which is then punctured by the ring tip of the converging surface wave at the impacting Weber number range of [45, 225]. The film shell then coalesces with the droplet, falls off with a fixed velocity and is eventually ejected as a bubble leaving the droplet with a transplanted surface of the soap solution.

This study examines the precursors and consequences of rare backflow events at the wall using direct numerical simulation of turbulent pipe flow with a high spatiotemporal resolution. The results obtained from conditionally averaged fields reveal that the precursor of a backflow event is the asymmetric collision between a high- and a low-speed streak (LSS) associated with the sinuous mode of the streaks. As the collision occurs, a lifted shear layer with high local azimuthal enstrophy is formed at the trailing end of the LSS. Subsequently, a spanwise or an oblique vortex spontaneously arises. The dominant nonlinear mechanism by which this vortex is engendered is enstrophy intensification due to direct stretching of the lifted vorticity lines in the azimuthal direction. As time progresses, this vortex tilts and orientates towards the streamwise direction and, as its enstrophy increases, it induces the breakdown of the LSS located below it. Subsequently, this vortical structure advects as a quasi-streamwise vortex, as it tilts and stretches with time. As a result, it is shown that reverse flow events at the wall are the signature of the nonlinear mechanism of the self-sustaining process occurring at the near-wall region. Additionally, each backflow event has been tracked in space and time, showing that approximately 50 % of these events are followed by at least one additional vortex generation that gives rise to new backflow events. It is also found that up to a maximum of seven regenerations occur after a backflow event has appeared for the first time.

We study the sound generation mechanism of initially subsonic viscous vortex reconnection at vortex Reynolds number $Re~(\equiv \text {circulation}/\text {kinematic viscosity})=1500$ through decomposition of Lighthill's acoustic source term. The Laplacian of the kinetic energy, flexion product, enstrophy and deviation from the isentropic condition provide the dominant contributions to the acoustic source term. The overall (all time) extrema of the total source term and its dominant hydrodynamic components scale linearly with the reference Mach number $M_o$; the deviation from the isentropic condition shows a quadratic scaling. The significant sound arising from the flexion product occurs due to the coiling and uncoiling of the twisted vortex filaments wrapping around the bridges, when a rapid strain is induced on the filaments by the repulsion of the bridges. The spatial distributions of the various acoustic source terms reveal the importance of mutual cancellations among most of the terms; this also highlights the importance of symmetry breaking in the sound generation during reconnection. Compressibility acts to delay the start of the sequence of reconnection events, as long as shocklets, if formed, are sufficiently weak to not affect the reconnection. The delayed onset has direct ramifications for the sound generation by enhancing the velocity of the entrained jet between the vortices and increasing the spatial gradients of the acoustic source terms. Consistent with the near-field pressure, the overall maximum instantaneous sound pressure level in the far field has a quadratic dependence on $M_o$. Thus, reconnection becomes an even more dominant sound-generating event at higher $M_o$.

Phase-reduction analysis captures the linear phase dynamics with respect to a limit cycle subjected to weak external forcing. We apply this technique to study the phase dynamics of the self-sustained oscillations produced by a Rijke tube undergoing thermoacoustic instability. Through the phase-reduction formulation, we are able to reduce these dynamics to a scalar equation for the phase, which allows us to efficiently determine the synchronisation properties of the system. For the thermoacoustic system, we find the conditions for which $m:n$ frequency locking occurs, which sheds light on the mechanisms behind asynchronous and synchronous quenching. We also reveal the optimal placement of pressure actuators that provide the most efficient route to synchronisation.

Precipitation in the forms of snow, hail, and rain plays a critical role in the exchange of mass, momentum and heat at the surfaces of lakes and seas. However, the microphysics of these interactions are not well understood. Motivated by recent observations, we study the physics of the impact of a single frozen canonical particle, such as snow and hail, onto the surface of a liquid bath using a numerical model. The descent, melting, bubble formation and thermal transport characteristics of this system are examined. Three distinct response regimes, namely particle impact, ice melting and vortex ring descent, have been identified and characterized. The melting rate and air content of the snow particle are found to be leading factors affecting the formation of a coherent vortex ring, the vertical descent of melted liquid and the vortex-induced transportation of the released gas bubble to lower depths. It is found that the water temperature can substantially alter the rate of phase change and subsequent flow and thermal transport, while the particle temperature has minimal effect on the process. Finally, the effects of the Reynolds, Weber and Stefan numbers are examined and it is shown that the Reynolds number modifies the strength of the vortex ring and induces the most significant effect on the flow dynamics of the snow particle. Also, the change of Weber number primarily alters the initial phases of snow–bath interaction while modifying the Stefan number of the snow particle essentially determines the system response in its later stages.