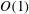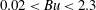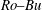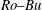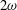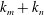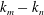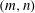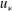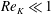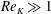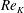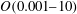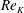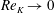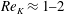Contents
Focus on Fluids
When is high Reynolds number shear flow not turbulent?
-
- Published online by Cambridge University Press:
- 03 July 2017, pp. 1-4
-
- Article
-
- You have access
- HTML
- Export citation
Papers
Two-stage autoignition and edge flames in a high pressure turbulent jet
-
- Published online by Cambridge University Press:
- 04 July 2017, pp. 5-41
-
- Article
- Export citation
Motion of a model swimmer near a weakly deforming interface
-
- Published online by Cambridge University Press:
- 04 July 2017, pp. 42-73
-
- Article
- Export citation
Sensitivity of internal wave energy distribution over seabed corrugations to adjacent seabed features
-
- Published online by Cambridge University Press:
- 04 July 2017, pp. 74-96
-
- Article
- Export citation
Stability of three-dimensional Gaussian vortices in an unbounded, rotating, vertically stratified, Boussinesq flow: linear analysis
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 97-134
-
- Article
- Export citation
Statistics and tensor analysis of polymer coil–stretch mechanism in turbulent drag reducing channel flow
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 135-173
-
- Article
- Export citation
Unsteady flow dynamics reconstruction from mean flow and point sensors: an experimental study
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 174-201
-
- Article
- Export citation
Experimental characterisation of the screech feedback loop in underexpanded round jets
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 202-229
-
- Article
- Export citation
On the instabilities of a potential vortex with a free surface
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 230-264
-
- Article
- Export citation
On the swirling Trkalian mean flow field in solid rocket motors
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 265-285
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Internal wave resonant triads in finite-depth non-uniform stratifications
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 286-311
-
- Article
- Export citation
Active control of transonic buffet flow
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 312-351
-
- Article
- Export citation
The K-type and H-type transitions of natural convection boundary layers
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 352-387
-
- Article
- Export citation
Transitional flows with the entropic lattice Boltzmann method
-
- Published online by Cambridge University Press:
- 05 July 2017, pp. 388-412
-
- Article
- Export citation
The variation of flow and turbulence across the sediment–water interface
-
- Published online by Cambridge University Press:
- 06 July 2017, pp. 413-437
-
- Article
-
- You have access
- HTML
- Export citation
Linear and weakly nonlinear analyses of cylindrical Couette flow with axial and radial flows
-
- Published online by Cambridge University Press:
- 06 July 2017, pp. 438-476
-
- Article
- Export citation
Generalized rapid-distortion theory on transversely sheared mean flows with physically realizable upstream boundary conditions: application to trailing-edge problem
-
- Published online by Cambridge University Press:
- 06 July 2017, pp. 477-512
-
- Article
- Export citation
Numerical study of convective sedimentation through a sharp density interface
-
- Published online by Cambridge University Press:
- 06 July 2017, pp. 513-549
-
- Article
- Export citation
Pore-filling events in single junction micro-models with corresponding lattice Boltzmann simulations
-
- Published online by Cambridge University Press:
- 06 July 2017, pp. 550-573
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Asymptotic analysis of the evaporation dynamics of partially wetting droplets
-
- Published online by Cambridge University Press:
- 06 July 2017, pp. 574-623
-
- Article
- Export citation