Understanding the fluid dynamics associated with a circular cylinder oscillating normal to a plane wall is important for safe design of offshore infrastructure, such as power cables and pipeline risers. This paper investigates the fluid dynamics of an oscillating cylinder with no imposed incident current experimentally using flow visualisation and force measurements where the ratio of the cylinder Reynolds number ($Re$) to Keulegan–Carpenter number ($KC$) is $\beta =500$ and $KC$ varies between 2 and 12. The minimum distance between the cylinder and wall was between 12.5 % and $50\,\%$ of the diameter. Across this parameter space three primary vortex flow regimes were observed: (i) for $KC\leq 5$, the flow field is approximately symmetric about the cylinder centreline and the velocity field between the cylinder and the wall resembled a pumping flow in phase with cylinder motion, which is well predicted by potential theory for most of the cycle; (ii) for $5< KC<8$, the flow field is increasingly asymmetric but with frequent switching of the side associated with vortex shedding; and (iii) for $KC\geq 8$, the flow field is consistently asymmetric due to vortex shedding. The in-line force increases when the cylinder is near the wall due to dynamic pressures associated with pumping. This increase can be estimated using potential theory superimposed onto the force time history for an isolated cylinder at the same $KC$ and $Re$. This study complements recent numerical modelling focused on low Reynolds number conditions and provides important insights into the fluid mechanics associated with trenching beneath cable and pipeline risers.

We show that in free surface flows, a uniform, streamwise current over small-amplitude wavy bottom topography generates cross-stream drift velocity. This drift mechanism, referred to as the current–bathymetry interaction-induced drift (CBIID), is specifically understood in the context of a simplified nearshore environment consisting of a uniform alongshore current, onshore-propagating surface waves and monochromatic wavy bottom making an oblique angle with the shoreline. The CBIID is found to originate from the steady, non-homogeneous solution of the governing system of equations. Similar to Stokes drift induced by surface waves, CBIID also generates a compensating Eulerian return flow to satisfy the no-flux lateral boundaries, e.g. the shoreline. The CBIID increases with an increase in particle's initial depth, bottom undulation amplitude and the strength of the alongshore current. Additionally, CBIID near the free (bottom) surface increases (decreases) with an increase in bottom undulation's wavelength. Maximum CBIID is obtained for long-wavelength bottom topography that makes an angle of approximately ${\rm \pi} /4$ with the shoreline. Unlike Stokes drift, particle excursions due to current–bathymetry interactions might not be small, and hence analytical expressions based on the small-excursion approximation could be inaccurate. We provide an alternative $z$-bounded approximation, which leads to highly accurate expressions for drift velocity and time period of particles especially located near the free surface. Realistic parametric analysis reveals that in some nearshore environments, CBIID's contribution to the net Lagrangian drift can be as important as Stokes drift, implying that CBIID can have major implications in cross-shelf tracer transport.

A recent experiment by Wang et al. (Soft Matt., vol. 17, 2021, pp. 2985–2993) shows that a self-propelled compound drop in a surfactant-laden solution can autonomously change its motion from a straight line to a spiraling trajectory, enhancing its capability for chemical detection, catalytic reaction and pollutant removal in a large fluid region. To understand the underlying physics of this peculiar motion, we develop a two-dimensional minimal model to study the swimming dynamics of a compound droplet driven by a self-generated Marangoni stress. We find that, depending on the Péclet number ($Pe$) and the viscosity and volume ratios of the two compound phases, the drop can swim in a variety of trajectories, including straight lines, circles, zigzag curves and chaotic trajectories. The drop moves in circles when its two components have comparable volumes. Otherwise, it shows other types of motions depending on $Pe$. Our simulation results for the circular motion at small $Pe$ are qualitatively comparable to the experiment. The transition between zigzag and circular trajectories is mainly determined by the orientation of high-order modes with respect to the drop's swimming direction. For most compound drops, the speed decays as $Pe^{-1/3}$ at high Péclet numbers as it does for a single-phase drop. A drop with two equal components undergoes a run-and-reorient motion due to the competition between the even and odd modes.

We analyse the approach towards local isotropy in statistically stationary turbulent shear flows using the transport equations for the fourth-order moments of the velocity derivative. It is found that terms of these equations representing the large-scale contribution associated with the uniform mean velocity gradient gradually decrease as the Taylor microscale Reynolds number $Re_\lambda$ increases, and finally disappear when $Re_\lambda$ is sufficiently large. This gradual weakening of the large-scale effect is accompanied by a gradual approach towards local isotropy of the small-scale motion. The rate at which local isotropy is approached depends on the weakening of the large-scale forcing, which is controlled by the magnitude of the non-dimensional velocity shear parameter $S^*$ ($\equiv \overline {u_1^2}({{\partial {{\bar U}_1}}}/{{\partial {x_2}}})/{\bar {\varepsilon }_{iso}}$, where $\bar {\varepsilon }_{iso}$ is the isotropic mean turbulent energy dissipation rate, $\overline {u_1^2}$ is the streamwise velocity variance, and ${\partial {{\bar U}_1}/\partial {x_2}}$ is the uniform mean velocity gradient in the transverse direction). In particular, we show that the approach towards local isotropy can be recast in the form $C\, Re_\lambda ^{-1}$, where $C$ is the product of $S^*$ and a ratio of transverse-to-streamwise velocity derivative variances. This is consistent with the behaviour of the normalized third-order moments of transverse velocity derivatives. With the further use of the transport equations for the eighth- and twelfth-order velocity derivative moments, it is found that the even moments of transverse velocity derivatives can significantly affect the rate at which local isotropy is approached, especially for higher orders.

We revisit and extend the turbulent Froude number (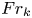$Fr_k$) scaling for the mixing coefficient (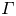$\varGamma$) introduced by Garanaik & Venayagamoorthy (GV) (J. Fluid Mech., vol. 867, 2019, pp. 323–333) by directly incorporating the effects of mean shear through the non-dimensional shear parameter 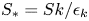$S_{\ast } = S k/\epsilon _k$. For flows where the effects of mean shear are stronger than the background vertical stratification, we find 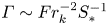$\varGamma \sim Fr_k^{-2} S_\ast ^{-1}$ for weakly stratified sheared turbulence and 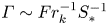$\varGamma \sim Fr_k^{-1}S_\ast ^{-1}$ for moderately stratified sheared turbulence. The scaling procedure is inconclusive for strongly stratified sheared turbulence, but using two independent datasets of homogeneous, sheared, stably stratified turbulence, we empirically observe 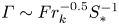$\varGamma \sim Fr_k^{-0.5} S_\ast ^{-1}$. Our revised scaling better collapses both datasets compared with the original GV scaling, and we note that the moderately stratified sheared regime is extremely narrow (or maybe even non-existent). We also apply our scaling to the time-varying open channel simulations of Issaev et al. (J. Fluid Mech., vol. 935, 2022) and observe 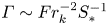$\varGamma \sim Fr_k^{-2}S_\ast ^{-1}$ for weakly stratified sheared turbulence, but we observe deviations from our revised scaling for moderate and strong stratifications due to time-varying mean shear and vertical transport. Finally, we apply our revised scaling to field measurements of Conry, Kit & Fernando (Environ. Fluid Mech., vol. 20, 2020, pp. 1177–1197) and observe $\varGamma \sim Fr_k^{-2} S_\ast ^{-1}$. We emphasize that our revised scaling is applicable only for stably stratified, vertically sheared turbulence with weak spatio-temporal variations of the mean shear and stratification, and we expect different scaling to apply when additional effects such as depth-varying radiative heating/cooling are present or when the orientation of the mean shear relative to the gravity vector is modified (e.g. horizontal shear).

Since its development, Stokesian dynamics has been a leading approach for the dynamic simulation of suspensions of particles at arbitrary concentrations with full hydrodynamic interactions. Although developed originally for the simulation of passive particle suspensions, the Stokesian dynamics framework is equally well suited to the analysis and dynamic simulation of suspensions of active particles, as we elucidate here. We show how the reciprocal theorem can be used to formulate the exact dynamics for a suspension of arbitrary active particles, and then show how the Stokesian dynamics method provides a rigorous way to approximate and compute the dynamics of dense active suspensions where many-body hydrodynamic interactions are important.

We characterise the incompressible turbulence cascade in terms of the concurrent inter-scale and inter-space exchanges of the scale-by-scale energy, helicity and enstrophy. The governing equations for the scale-by-scale helicity and enstrophy are derived in a similar fashion to that of the second order structure function following Hill (J. Fluid Mech., vol. 468, 2002, pp. 317–326). We examine the instantaneous dynamics, applying these equations to forced periodic turbulence and a von Kármán flow focusing on scales in the dissipative range $r=2.5\eta$, the near-dissipative range $r=0.5\lambda$ and the onset inertial range $r=\lambda$ (where $\eta$ and $\lambda$ are the Kolmogorov and Taylor length scales, respectively). The signature of the random sweeping effect is observed in all three individual budgets and between the energy and enstrophy transfers. As in the energy cascade, the anti correlation of the pressure transport and non-linear transfer is identified also in the helicity cascade. Owing to its lack of positive definiteness, the helicity transfers are found to be decorrelated from the others. However a connection between the energy cascade and helicity is identified kinematically. This connection reveals the large-scale sweeping motions are a key element in the overall energy cascade and underpins previous observations of large-scale intermittency. Taken together, this work extends a classic framework to gain novel insight on turbulence dynamics that underlay the statistically steady state, and demonstrates how transfers are interconnected.

Passive heat transfer enhancement by spanwise rollers associated with the Kelvin–Helmholtz instability was studied through direct numerical simulations of high-aspect-ratio longitudinal ribs at the friction Reynolds number $300$. The temperature was treated as a passive scalar with Prandtl number unity to discuss the similarity between the heat and momentum transfer. The results reveal that the high-aspect-ratio longitudinal ribs lead to a favourable breakdown of the Reynolds analogy, that is, the enhancement of the heat transfer rate surpasses that of the frictional resistance. The favourable breakdown of the Reynolds analogy can be attributed to the enhanced turbulent heat flux compared with the Reynolds shear stress, whereas the rib-induced secondary flow plays a role in reducing the favourable breakdown of the Reynolds analogy. The conditional average statistics reveal that the high-pressure region accompanied by the spanwise rollers suppresses the spanwise roller-induced sweep and ejection motions, leading to smaller Reynolds shear stress than for the turbulent heat flux.

The radiation force exerted on an object by an acoustic wave is a widely studied phenomenon since the early work of Rayleigh, Langevin and Brillouin, and has led in the last decade to tremendous developments for acoustic micromanipulation. Despite extensive work on this phenomenon, the expressions of the acoustic radiation force applied on a particle have so far been derived only for a steady particle, hence neglecting the effect of its displacement on the radiated wave. In this work, we study the acoustic radiation force exerted on a monopolar source translating at a constant velocity that is small compared to the sound speed. We demonstrate that the asymmetry of the emitted field resulting from the Doppler effect induces a radiation force on the source opposite to its motion.

We explore the motion of an axisymmetric gravity current in an anisotropic porous medium in which the horizontal permeability is larger than the vertical permeability. It is well known that the classical axisymmetric gravity current supplied by a constant point source of fluid has an unphysical singularity near the origin. We address this by considering a pressure-dominated region near the origin which allows for vertical flow from the source, such that the current remains of finite depth, whilst beyond this region the flow is gravity dominated. At early times the inner pressure-driven region controls the spreading of the current, but at late times the inner region occupies a progressively smaller fraction of the current such that the radius increases as ${\sim }t^{3/7}$, while the depth near the origin increases approximately as ${\sim }t^{1/7}$. The presence of anisotropy highlights this phenomenon, since the vertical permeability maintains an effect on the flow at late times through the pressure-driven flow near the origin. Using these results we provide some quantitative insights into the dominant dynamics which controls CO$_2$ migration through permeable aquifers, as occurs in the context of carbon capture and storage.

The propagation of the gravity current generated from a moving source of buoyancy is of interest in deep-sea mining and related technologies. The study by Ouillon et al. (J. Fluid Mech., vol. 924, 2021, A43) elucidated some salient patterns of the flow concerning a source close to the bottom on the basis of direct numerical simulation on a supercomputer. Here, we present a simple box model that provides further insights and useful analytical approximations for this gravity-current flow system. We show that this flow is very different from that produced by a moving source at the top, studied by Hogg et al. (J. Fluid Mech., vol. 539, 2005, pp. 349–385). The model confirms that the main governing parameter is the ratio $a$ of speed of source to that of buoyancy propagation. The model points out dependency also on the front-jump Froude number (which implies dependency on the height of the ambient fluid). For a sufficiently large $a >a_{crit}$, a supercritical regime appears in which the gravity current forms a wedge behind the moving source; in the subcritical regime, the upstream propagation attains a maximum $x_m$ at time $t_m$. The model predicts the value $a_{crit}$, the distance and time $x_m$ and $t_m$ in the subcritical case, and the shape of the wedge in the supercritical case, without any adjustable constant. Comparisons with the numerical data show fair agreement.

Correlations for the interfacial terms in the fluid dissipation rate equation and Reynolds stress equations are established for particle-laden flows, based on data from the interfaced-resolved direct numerical simulations of particle sedimentation in a periodic domain at a density ratio ranging from 0.01 to 1000, a particle concentration ranging from 2.3 % to 30.2 % and a particle Reynolds number below 250. The correlations for the mean drag and the pseudo-turbulent kinetic energy are also reported, which are used for the modelling of the interfacial term in the fluid dissipation rate equation. The interfacial term correlations obtained are then incorporated in the Reynolds stress model (RSM) (i.e. second-moment closure) for the simulation of vertical turbulent channel flows laden with the finite-size particles at relatively low particle volume fractions. The results show that the RSM with new interfacial term correlations can quantitatively predict particle-induced turbulence enhancement or suppression in vertical channel flows.

Vortex-induced vibration (VIV) of a two degree-of-freedom (DOF) circular cylinder, placed in the test section of a recirculating water tunnel and free to oscillate in its first two vibrational modes in the crossflow direction, is studied experimentally. The dynamic response of the cylinder is studied for a reduced velocity range of $U^*=4\unicode{x2013}30$ for eigenfrequency ratios in the range of 1.3–3.0. For the two DOF system, while the onset of the VIV response followed a similar lock-in region as those observed for a classical VIV response of a single DOF system, by increasing the reduced velocity a secondary lock-in region was observed over which the oscillations of the cylinder were locked into the system's second mode. In addition, there existed an intermediate range of reduced velocity over which the VIV response consisted of oscillations at a combination of the first two natural modes of the system. As the eigenfrequency ratio between the first two modes increased, the secondary lock-in range was extended to higher reduced velocities and the reduced velocity range over which multi-modal oscillations were observed was decreased. A full map of vortex dynamics in the wake of the cylinder was developed qualitatively and quantitatively using hydrogen bubble flow visualization and time-resolved volumetric particle tracking velocimetry techniques, respectively. A Q-criterion analysis revealed the existence of highly three-dimensional vortex structures in the wake of the cylinder. The spatiotemporal mode analysis using the proper orthogonal decomposition technique revealed strong coupling between the vortex shedding modes in the wake of the cylinder and the structural vibration modes.

We study the flow above non-optimal riblets, specifically large drag-increasing and two-scale trapezoidal riblets. In order to reach large Reynolds numbers and large scale separation while retaining access to flow details, we employ a combination of boundary-layer hot-wire measurements and direct numerical simulation (DNS) in minimal-span channels. Although the outer Reynolds numbers differ, we observe fair agreement between experiments and DNS at matched viscous–friction-scaled riblet spacings $s^+$ in the overlapping physical and spectral regions, providing confidence that both data sets are valid. We find that hot-wire velocity spectra above very large riblets with $s^+ \gtrsim 60$ are depleted of near-wall energy at scales that are (much) greater than $s$. Large-scale energy likely bypasses the turbulence cascade and is transferred directly to secondary flows of size $s$, which we observe to grow in strength with increasing riblet size. Furthermore, the present very large riblets reduce the von Kármán constant $\kappa$ of the spanwise uniform mean velocity in a logarithmic layer and, thus, reduce the accuracy of the roughness-function concept, which we link to the near-wall damping of large flow structures. Half-height riblets in the groove, which we use as a model of imperfectly repeated (spanwise-varying) riblets, impede in-groove turbulence. We show how to scale the drag optimum of imperfectly repeated riblets based on representative measurements of the true geometry by solving inexpensive Poisson equations.

This paper presents a detailed analysis of the flows induced in a long two-dimensional cavity heated from below in the presence of streaming due to ultrasound acoustic waves emitted by a source. The problem is tackled by using performing spectral element codes, allowing continuation of steady solutions, bifurcation points and periodic cycles. For a given dimensionless source size, the governing parameters are the acoustic streaming parameter $A$ which modulates the acoustic force generating the Eckart streaming and the Rayleigh number ${\textit {Ra}}$ which quantifies the buoyant force responsible for the convection. The streaming flow, which goes to the right along the horizontal axis and returns along the lower and upper boundaries, influences the instability thresholds, which are first strongly stabilized above the pure Rayleigh–Bénard threshold ${\textit {Ra}}_0$ when $A$ is increased, before a destabilization to reach the pure streaming threshold $A_c$ at ${\textit {Ra}}=0$. The steady multi-roll convective flow generated without streaming is replaced by periodic waves when $A$ is increased, forward waves for moderate $A$ and backward waves for large $A$. The transition between these waves induces a specific dynamics involving steady flows, which has been elucidated. The waves also eventually disappear for a sufficient increase of the Rayleigh number, replaced by steady multi-roll flows hardly influenced by the streaming flow. A very rich dynamics is thus observed with the competition between the waves and the steady flows.

The hydrodynamic cavitation in semidilute solution flows of a flexible polymer additive in water was experimentally explored in a mesoscale converging–diverging nozzle to elucidate the cavitation reduction effects of polymer additives. Rheological measurements demonstrated that polymer solutions were shear-thinning, with infinite viscosities larger than pure water. The polymer additives significantly mitigated the intensity of cloud cavitation and the growth of violent cavity structures in the tested solution concentrations. Under conditions of supercavitation, the tested polymer solutions could not suppress the growth of large structures but showed a reduction in the population of cavitation bubbles. The temporal evolution and spatial variation of cavitation structures in different concentrations were captured using high-speed imaging. Statistical analysis of the images showed that polymers reduce cavitation via three main mechanisms. (1) The longitudinal expansion of cavities downstream is attenuated relative to the pure water. The streamwise distribution of vapour-ratio fluctuations was flattened, and its peak was shifted upstream in the solutions. (2) Mean collapse and growth rate of cavitating bubble pockets and their fluctuations were noticeably relaxed by polymer additives. For a 400 p.p.m. solution (parts per million (p.p.m.)), a reduction of 65 % was measured relative to pure water flow at the highest tested flow rate. (3) Spectral analysis of the downstream pressure indicated that the shedding frequency at the cavitation inception was reduced as the solution's concentration increased. This reduction was as high as 70 % for a 400 p.p.m. solution. These results highlight the strong interplay between polymer additives and the generation of cavitation-related structures.

We study a two-dimensional (2-D) potential flow of an ideal fluid with a free surface with decaying conditions at infinity. By using the conformal variables approach, we study a particular solution of the Euler equations having a pair of square-root branch points in the conformal plane, and find that the analytic continuation of the fluid complex potential and conformal map define a flow in the entire complex plane, excluding a vertical cut between the branch points. The expanded domain is called the ‘virtual’ fluid, and it contains a vortex sheet whose dynamics is equivalent to the equations of motion posed at the free surface. The equations of fluid motion are analytically continued to both sides of the vertical branch cut (the vortex sheet), and additional time invariants associated with the topology of the conformal plane and Kelvin's theorem for a virtual fluid are explored. We called them ‘winding’ and virtual circulation. This result can be generalized to a system of many cuts connecting many branch points, resulting in a pair of invariants for each pair of branch points. We develop an asymptotic theory that shows how a solution originating from a single vertical cut forms a singularity at the free surface in infinite time, the rate of singularity approach is double exponential and supersedes the previous result of the short branch cut theory with finite time singularity formation. The present work offers a new look at fluid dynamics with a free surface by unifying the problem of motion of vortex sheets, and the problem of 2-D water waves. A particularly interesting question that arises in this context is whether instabilities of the virtual vortex sheet are related to breaking of steep ocean waves when gravity effects are included.

In this study, the effects of rotor–rotor interaction on wake characteristics were investigated experimentally for a twin-rotor configuration in axial descent. The wake velocities were measured at descent rates (descent speed/induced velocity at the rotor disk during hover) from 0.87 to 1.52, and the rotor–rotor interaction strength was controlled by adjusting the distance between the rotor tips. As the descent rate increased, the wake of the isolated rotor gradually entered the vortex ring state (VRS), where the flow established an extensive recirculation zone. Correlation analysis was performed to distinguish the rotor wake between tubular and VRS topologies. The flow states for the isolated rotor were classified into pre-VRS, incipient VRS, and fully developed VRS, depending on the probability of vortex ring formation. The results reveal that the effects of rotor–rotor interaction on the wake characteristics of twin rotors differ depending on the descent rate, distance between rotor tips, and wake region. In the outer region, the flow state of the rotor wake remains consistent with that of the isolated rotor, irrespective of the distance between rotor tips. Conversely, the strong rotor–rotor interaction changes the flow state in the inner region by disrupting the vortex ring structure, intensifying the wake asymmetry about the rotational axis. The thrust measurements show that under the VRS, as the two rotors get closer, the thrust coefficient increases until vortex ring disruption occurs, and then decreases after the vortex ring is disrupted.

The classical Gill's stability problem for stationary and parallel buoyant flow in a vertical porous slab with impermeable and isothermal boundaries kept at different temperatures is reconsidered from a different perspective. A three-layer slab is studied instead of a homogeneous slab as in Gill's problem. The three layers have a symmetric configuration where the two external layers have a high thermal conductivity, while the core layer has a much lower conductivity. A simplified model is set up where the thermal conductivity ratio between the external layers and the internal core is assumed as infinite. It is shown that a flow instability in the sandwiched porous slab may arise with a sufficiently large Rayleigh number. It is also demonstrated that this instability coincides with that predicted in a previous analysis for a homogeneous porous layer with permeable boundaries, by considering the limiting case where the permeability of the external layers is much larger than that of the core layer.

We study a gravity-driven viscous flow coating a vertical cylindrical fibre. The destabilisation of a draining liquid column into a downward moving train of beads has been linked to the conjunction of the Rayleigh–Plateau and Kapitza instabilities in the limit of small Bond numbers $Bo$. Here, we focus on quasi-inertialess flows (large Ohnesorge number $Oh$) and conduct a linear stability analysis on a unidirectional flow along a rigid eccentric fibre for intermediate to large $Bo$. We show the existence of two unstable modes, namely pearl and whirl modes. The pearl mode depicts asymmetric beads, similar to that of the Rayleigh–Plateau instability, whereas a single helix forms along the axis in the whirl mode instability. The geometric and hydrodynamic thresholds of the whirl mode instability are investigated, and phase diagrams showing the transition thresholds between different regimes are presented. Additionally, an energy analysis is carried out to elucidate the whirl formation mechanism. This analysis reveals that despite the unfavourable capillary energy cost, the asymmetric interface shear distribution, caused by the fibre eccentricity, has the potential to sustain a whirling interface. In general, small fibre radius and large eccentricity tend to foster the whirl mode instability, while reducing $Bo$ tends to favour the dominance of the pearl mode instability. Finally, we compare the predictions of our model with the results of some illustrative experiments, using highly viscous silicone oils flowing down fibres. Whirling structures are observed for the first time, and the measured wavenumbers match our stability analysis prediction.